[page i]

THE

ELEMENTS OF ALGEBRA:

DESIGNED

FOR THE USE OF STUDENTS

IN THE

UNIVERSITY.

BY JAMES WOOD, D. D.

DEAN OF ELY, AND

MASTER OF ST. JOHN'S COLLEGE, CAMBRIDGE.

EIGHTH EDITION.

CAMBRIDGE:

Printed by J. Smith, Printer to the University;

AND SOLD BY DEIGHTON & SONS, AND T. STEVENSON, CAMBRIDGE;
AND J. MAWMAN, LUDGATE-STREET, LONDON.

1825

[page ii]

[page iii]

CONTENTS.

	PAGE
ON Vulgar Fractions	1
On Decimal Fractions	16
Signs used in Algebra	24
The Addition of Algebraical Quantities	29
Subtraction	32
Multiplication	33
Division	38
On Algebraical Fractions	42
Involution and Evolution	51
Simple Equations	64
Quadratic Equations	79
On Ratios	91
On Proportion	95
On Variation	103
On Arithmetical Progression	109
On Geometrical Progression	111
On Permutations and Combinations	115
The Binomial Theorem	117
On Surds	123
The Nature of Equations	135
The Transformation of Equations	142
The Limits of the Roots of Equations	151
The Depression of Equations	164
The Solution of Recurring Equations	168
The Solution of a Cubic Equation	170
Des Cartes's Solution of a Biquadratic	174
Dr. Waring's Solution	176
The Method of Divisors	178
The Method of Approximation	183
The Reversion of Series	187
The Sums of the Powers of the Roots of an Equation	190

[page iv]

	PAGE
On the Impossible roots of an Equation	194
On Unlimited Problems	203
On Continued Fractions	209
The Value of a Fraction whose Numerator and Denominator are evanescent	213
The least common Multiple	214
The cube root of a + √- b2	217
On Logarithms	ibid.
On Interest and Annuities	220
On the Summation of Series	227
On Chances	259
On Life Annuities	272
On the Nature of Curves	276
On the Construction of Equations	292
General Properties of Curve Lines	298

[page 1]

INTRODUCTION.

ON VULGAR FRACTIONS.

ART. 1. A fraction is a quantity which represents a part of parts of an integer or whole.

(2.) A simple fraction consists of two members, the numerator and the denominator; the denominator shews into how many equal parts the whole, or unity, is divided; and the numerator, the number of those parts taken. The numerator is usually placed over the denominator with a line between them. Thus, 2/3, two thirds, signifies that unity is divided into three equal parts, and that two of those parts are taken.

It must be observed, that we suppose every integer to be divisible into any number of equal parts at pleasure.

(3.) A proper fraction is one whose numerator is less than it's denominator, as 7/8.

(4.) An improper fraction is one whose numerator is equal to, or greater than its denominator, as 6/6;7/5.

A

[page] 2

(5.) A compound fraction is a fraction of a fraction, as 3/4 of 5/6, where 5/6 is the whole quantity of which 3/4 is to be taken; also, 2/3 of 4/5 of 9/11, is a compound fraction; &c.

(6.) A quantity consisting of a whole number and a fraction is called a mixed number, as 7 3/10, which signifies 7 integers together with 3/10 of an integer.

(7.) COR. 1. Every integer may be considered as a fraction whose denominator is 1; thus 5, or five units, is 5/1.

(8.) COR. 2. To multiply a fraction by any number, multiply the numerator by that number and retain the same denominator. Thus, 2/15 multiplied by 7 is 14/15. For, the unit, in each of the fractions and 2/15 and 14/15, is divided into 15 equal parts, and 7 times as many of those parts are taken in the latter case as in the former.

(9.) COR. 3. To divide a fraction by any number, multiply the denominator by that number and retain the same numerator. Thus, 3/5 divided bv 4 is 3/20. For, the unit being divided into four times as many equal parts in 3/20 as it is in 3/5, each of the parts in the latter case is four times as great as in the former, and

[page] 3

the same number of parts is taken in both cases; therefore, the former fraction is one fourth of the latter.

(10.) A simple fraction may be considered as representing the quotient arising from the division of the numerator by the denominator; thus the fraction 3/4 represents the quotient of 3 divided by 4; for 3 is 3/1 (Art. 7.), and this divided by 4 is the fraction 3/4 (Art. 9.) If the integer be supposed a pound, or twenty shillings, 3/4 of £.1, which is 15 shillings, is equal to 1/4 of £.3, which is also 15 shillings.

(11.) If the numerator and denominator of a fraction be both multiplied by the same number, it's value is not altered. For, if the numerator be multiplied by any number, the fraction is multiplied by that number (Art. 8); and if the denominator be multiplied by the same number, the fraction is divided by it (Art. 9); and if a quantity be both multiplied and divided by the same number, it's value is not altered. Thus, 5/14 = 15/42 = 150/420, &c. Hence, if the numerator and denominator be both divided by the same number, it's value is not altered; since 150/420 = 15/42 = 5/14*.

* To avoid repetition, the Reader is referred to the first section of the Algebra, for the explanation of the signs +, -, ×, and =

A 2

[page] 4

ON REDUCTION.

The operation by which a quantity is changed from one denomination to another, without altering it's value, is called Reduction.

(12.) To reduce a whole number to a fraction with a given denominator.

Multiply the proposed number by the given denominator, and the product will be the numerator of the fraction required.

Ex. Reduce 5 to a fraction whose denominator is 6.

This is or 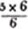or 30/6; because 5 may be considered as a fraction 5/1 (Art. 7), the numerator and denominator of which are multiplied by 6, therefore it's value is not altered. (Art. 11).

(13.) To reduce a mixed number to an improper fraction.

Multiply the integral by the denominator of the fractional part, to this product add the numerator of the fractional part, and make it's denominator, the denominator of the sum.

Ex. 1. Reduce 7 4/5 to an improper fraction.

The quantity 7 4/5 is equal to for 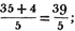for 7 (by the last Art.) is equal to 35/5, and if to this 4/5 be added, the whole is 39/5.

Ex. 2. Also,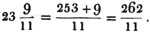

[page] 5

(14.) To reduce an improper fraction to a mixed number.

Divide the numerator by the denominator for the integral part, and make the remainder the numerator of the fractional part, and the divisor it's denominator.

Ex. Reduce 39/5 to an improper fraction. The fraction 39/5 = 7 4/5; because the unit being divided into 5 parts, 39 such, parts are to be taken, that is, 7 units and 4 such parts.

(15.) To reduce a compound fraction to a simple one.

Multiply all the numerators together for a new numerator, and all the denominators for a new. denominator.

Ex. 1. 2/3 of 4/5 = 8/15; for, one third of 4/5 is 4/15, (Art. 9); therefore two thirds, which must be twice as, great, is 8/15 (Art. 8).

Ex. 2. 3/4 of 5 = 3/4 of 5 = 3/4 of 5/1 = 15/4.

Mixed numbers must be reduced to improper fractions, before the rule can be applied.

Ex. 3. 5/8 of 2/9 of 3 1/12 = 10/72 of 3 1/12 = 10/72 of 37/122 = 370/864.

(16.) To reduce a fraction to lower terms.

Whenever the numerator and denominator of a fraction have a common measure (or number which divides each of them without remainder) greater than

[page] 6

unity, the fraction may be reduced to lower terms, by dividing both the numerator and denominator by this common measure.

Ex. 105/120 is reduced to 21/24, by dividing both the numerator and denominator by 5; and 21/24 is again reduced to 7/8, by dividing it's numerator and denominator by 3. That the value of the fraction is not altered, appears from Art. 11.

In the same manner, 168/210 = 84/105 = 28/35 = 4/5.

(17.) The greatest common measure of two numbers is found by dividing the greater by the less, and the preceding divisor by the remainder, continually, till nothing is left: the last divisor is the greatest common measure required.

To find the greatest common measure of 189 and 224.

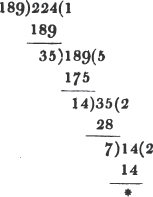
By proceeding according to the rule, it appears that 7

[page] 7

is the last divisor, or the greatest common measure sought. The proof of this rule will be given hereafter*.

(18.) A fraction is reduced to it's lowest terms, by dividing it's numerator and denominator by their greatest common measure.

Ex. To reduce 385/396 to it's lowest terms.

By the last Art. the greatest common measure of the numerator and denominator is found to be 11, and therefore 35/36 is the fraction in it's lowest terms.

COR. If unity be the greatest common measure of the numerator and denominator, the fraction is in it's lowest terms.

(19.) To reduce fractions to a common denominator.

Having reduced, if necessary, compound fractions to simple ones, and mixed numbers to improper fractions, multiply each numerator by all the denominators except it's own, for the new numerator, and all the denominators together for a common denominator.

Ex. 1. Reduce 1/2, 2/3 and 3/4 to a common denominator.

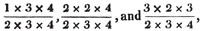or 12/14, 16/24 and 18/24 are the fractions required. These fractions are respectively equal to the former, the numerator and denominator in each case, having been multiplied by the same numbers, namely, the denominators of the rest.

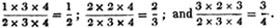
* Art. 90.

[page] 8

Ex. 2. Reduce 2/5 of 3/4 and 4 1/3 to a common denominator.

These are 6/20 and 13/3, or 3/10 and 13/3; therefore 9/30 and 130/30 are the fractions required.

(20.) If the denominator of one of two fractions contain the denominator of the other a certain number of times exactly, multiply the numerator and denominator of the latter by that number, and it will be reduced to the same denominator with the former.

Ex. Reduce 5/12 and 2/3 to a common denominator.

Since 12 contains 3 four times exactly, multiply both the numerator and denominator of 2/3 by 4, and it becomes 8/12, a fraction having the same denominator with 5/12.

(21.) COR. By reducing two fractions to a common denominator their values may be compared.

Thus, 4/7 and 7/12; when reduced to a common denominator are 48/84 and 49/84; that is, the fractions have the same relative values that 48 and 49 have.

(22.) To find the value of a fraction of a proposed denomination, in terms of a lower denomination.

[page] 9

Multiply the fraction by the number of integers of the lower denomination contained in one integer of the higher, and the product is the value required. The value of any fractional part of the lower denomination may be obtained in the same manner, till we come to the lowest.

Ex. 1. What is the value of 5/7 of a pound?

First, 5/7 of £.1 is 5/7 of 20 shillings, or 5/7 of 20/1 shillings = 100/7 = 14 2/7 shillings;

Next, 2/7 of a shilling = 2/7 of 12/1 pence = 24/7 pence = pence = 3 3/7 pence;

Lastly, 3/7 of a penny = 3/7 of farthings = 3/7 of 4/1, or 12/7 farthings = 1 5/7 farthings: hence, 5/7 of a pound is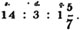

The operation is usually performed in the following manner:

[page] 10

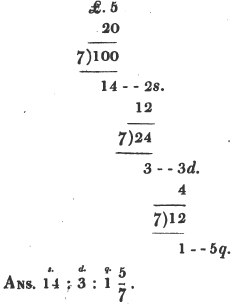
Ex. 2. What is the value of 5/9 of a crown?

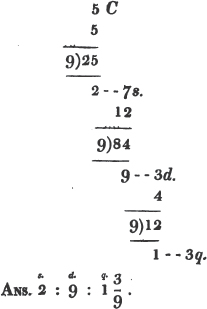
[page] 11

(23.) To reduce a quantity to a fraction of any denomination.

Make the given quantity the numerator, and the number of integers of it's denomination in one of the proposed denomination, the denominator, and the fraction required is determined.

Ex. 1. What fraction of a pound is 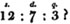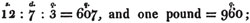therefore 607/960 is the fraction sought. Because the integer being divided into 960 equal parts, 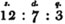contains 607 such parts.

(24.) In this example, we are obliged to reduce the whole to farthings; and in general, if the higher denomination do not contain the lower an exact number of times, reduce them to a common denomination, and proceed as before.

Ex. 2. What fraction of a guinea is half a crown?

Here sixpence is the greatest common denomination, of which a guinea contains 42, and half a crown 5, therefore 5/42 is the fraction required.

Any common denomination would answer thp purpose, but if the greatest be taken, the resulting fraction is in the lowest terms.

(25.) To reduce a fraction to any denomination.

Find what fraction of the proposed denomination an integer of the denomination of the given fraction is, and the fraction required will be found by Art. 15.

[page] 12

Ex. 1. What fraction of a pound is 2/3 of a shilling? 1 shilling is 1/20 of a pound, therefore 2/3 of 1 shilling is 2/3 of 1/20 of a pound, or 2/60 = 1/30 of a pound.

Ex. 2. What fraction of a yard is 5/7 of an inch?

1 inch is 1/36 of a yard, therefore 5/7 of an inch is 5/7 of 1/36 of a yard, or 5/252 of a yard.

Ex. 3. What fraction of a guinea is 4/9 of a pound?

1 pound is 20/21 of a guinea (Art. 24); hence 4/9 of a pound is 4/9 of 20/21 of a guinea, or 80/189 of a guinea.

ADDITION OF FRACTIONS.

(26.) If fractions have a common denominator, their sum is found, by taking the sum of the numerators, and subjoining the common denominator.

Ex. 1/5 + 2/5 = 3/5. For, if an integer be divided into five equal parts, one of those parts, together with two parts of the same kind, must make three such parts.

(27.) If the fractions have not a common denominator, reduce them to a common denominator, and proceed as before.

[page] 13

Ex. Required the sum of 2/3, 3/4 and 4/5.

These reduced to a common denominator are 40/60, 45/60 and 48/60, whose sum is 133/60, or 2 13/60.

When mixed numbers are to be added, to the sum of the fractions, taken as before, add the sum of the integers.

Ex. Add together 5 3/4, 6 1/3 and 2/5 of 1/7.

3/4 + 1/3 + 2/35 = 315/420 + 140/420 + 24/420 = 479/420 = 1 59/420; therefore the whole sum is 12 59/420.

SUBTRACTION.

(28.) The difference of two fractions which have a common denominator is found by taking the difference of their numerators and subjoining the common denominator.

Ex. 4/5 - 3/5 = 1/5. For, if the unit be supposed to be divided into five equal parts, and three of those parts be taken from four, the remainder must be one, or 1/5.

(29.) If the fractions have not a common denominator, let them be reduced to a common denominator, and take the difference as before.

[page] 14

Ex. 1. From 9/11 take 4/5.

9/11 - 4/5 = 45/55 - 44/55 = 1/55.

Ex. 2. From 11/12 of 3/5 take 1/3 of 7/8.

33/60 - 7/24 = 792/1440 - 420/1440 = 372/1440 = 31/120.

MULTIPLICATION.

(30.) DEF. To multiply one fraction by another, is to take such part or parts of the former as the latter expresses. This is done by multiplying the numerators of the two fractions together for a new numerator, and the denominators for a new denominator.

Ex. 3/4×5/7 = 15/28; for 3/4 multiplied by 5/7 is, according to the definition of multiplication, 5/7 of 3/4 15/28, (Art. 15.)

Compound fractions must be reduced to simple ones, and mixed numbers to improper fractions, and they may then be multiplied as before.

Ex. 1. Multiply 2/5 of 9/13 by 7 1/8.

2/5 of 9/13 = 18/65; and 7 1/8 = 57/8; therefore their product is 18/65×57/8 = 1026/520 = 1 506/520 = 1 253/260.

[page] 15

Ex. 2. Multiply 2/217 by 7,

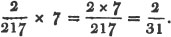
Hence it appears, that a fraction may be multiplied by a whole number, by dividing the denominator by that number, when this division can take place.

DIVISION.

(31.) To divide one fraction by another, or to determine how often one is contained in the other, invert the numerator and denominator of the divisor, and proceed as in multiplication.

Ex. 3/4 divided by 5/7 is 3/4×7/5 = 21/20 = 1 1/20.

For, from the nature of division, the divisor multiplied by the quotient must produce the dividend; therefore 5/7×quotient = 3/4; let these equal quantities be multiplied by the same quantity 7/5, and the products must be equal; that is, 7/5×5/7×quotients = 3/4×7/5 or 35/35×quotient = 21/20; but 35/35 = 1 (Art. 14); therefore the quotient = 21/20 according to the rule. And the same method of proof is applicable to all cases.

Compound fractions must be reduced to simple ones, and mixed numbers to improper fractions, before the rule can be applied.

[page] 16

Ex. Divide 5/9 of 4/7 by 3 1/3.

5/9 of 4/7 = 20/63, and 3 1/3 = 10/3; therefore the quotient is 20/63×3/10 = 60/630 = 2/21.

ON DECIMAL FRACTIONS.

(32.) In order to lessen the trouble which in many cases attends the use of vulgar fractions, decimal fractions have been introduced, which differ from the former in this respect, that their denominators are always 10 or some power of 10, as 100, 1000, 10000, &c. and instead of writing the denominator under the numerator, it is expressed by pointing off, from the right of the numerator, as many figures as there are cyphers in the denominator; thus,.2,.23,.127,.0013, 43.7, signify respectively 2/10, 23/100, 127/1000, 13/10000, 43 7/10 or 437/10.

(33.) COR. 1. The value of each figure in a decimal, decreases from the left to the right in a tenfold proportion; that is, each figure is ten times as great as if it were removed one place to the right, as in whole numbers; thus.2,.02,.002, are 2/10, 2/100, 2/1000, &c. and the decimal.127 is one tenth, two hundredths and seven thousandths of an unit.

[page] 17

(34.) COR. 2. Adding cyphers to the right of a decimal does not alter it's value; thus, .2, .20, .200, or 2/100, 20/100, 200/1000 are equal to each other, the numerator and denominator having been multiplied by the same number. (See Art. 11.)

(35.) COR. 3. Decimals may be reduced to a common denominator by adding cyphers to the right, where it is necessary, till the number of decimal places is the same in all.

Ex. 5,.01 and.311 reduced to a common denominator, are.500,.010 and.311; that is, 500/1000, 10/1000 and 311/1000.

As decimals are only fractions of a particular description, their operations must depend upon the principles already laid down.

ADDITION OF DECIMALS.

(36.) To find the sum of any number of decimals, place the figures in such a manner that those of the same denomination may stand under each other; add them together as in whole numbers, and place the decimal point in the sum under the other points.

Ex. Add together 7.9, 51.43 and.0118.

These, when reduced to a common denominator,

B

[page] 18

are 7.9000, 51.4300 and.0118; and proceeding according to the rule,

7.9000
51.4300
.0118
59.3418 is the sum required. (Art. 26.)

In the operation, the cyphers may be omitted; thus,

7.9
51.43
.0118
59.3418

SUBTRACTION.

(37.) To find the difference of two decimals, place the figures of the same denomination under each other; then subtract as in whole numbers, and place the decimal point under the other points.

From 61.3 take 42.012.

These, reduced to a common denominator, are 61.300 and 42.012; therefore their difference is 19.288 (Art. 28). In the operation, the cyphers may be omitted; thus,

61.3
42.012
19.288

[page] 19

MULTIPLICATION.

(38.) To multiply one decimal by another, multiply the figures as in whole numbers, and point off as many decimal places in the product as there are in the multiplier and multiplicand together.

Ex. 51.3×4.6 = 235.98. For, 513/10×46/10 = 23598/100 = (according to the decimal notation) 235.98. And a similar proof may be given in all other cases.

(39.) When there are fewer figures in the product than there are decimals in the multiplier and multiplicand together, cyphers must be annexed to the left of the product, that the decimal places may be properly represented.

Ex. 25 ×.3 = .075; for 25/100×3/10 = 75/1000 = (according to the decimal notation) .075.

DIVISION.

(40.) Division in decimals is performed as in whole numbers, observing to point off as many decimals in the quotient as the number of decimal places in the dividend exceeds the number in the divisor.

Ex. Divide 77.922 by 3.7.

77.922/3.7 = 21.06: here there are three decimals in the dividend, and one in the divisor; therefore, there are two in the quotient.

The truth of this rule is apparent from the nature of multiplication; for, the product of the divisor and

B 2

[page] 20

quotient is the dividend; there are, therefore, as many places of decimals in the dividend, as there are in the divisor and quotient together (Art. 38); consequently, there are as many in the quotient as the number in the dividend exceeds the number in the divisor.

(41.) If figures be wanting to make up the proper number of decimal places, cyphers must be added to the left.

Ex. Divide.336 by 42.

336/42 = 8; and as the quotient of.336 divided by 42 must contain three decimal places, that quotient is.008. For, 336/1000 divided by 42 is 336/42000, or 8/1000 (Art. 9); that is (according to the decimal notation).008 (Art. 32).

(42.) When the dividend does not contain as many decimals as the divisor, cyphers must be added to the right of the decimals in the dividend, till that is the case.

Ex. Divide 36 by. 012.

36 = 36.000; and 36.000 divided by.012 is 3000, according to the rule.

REDUCTION.

(43.) To reduce a vulgar fraction to a decimal.

Add cyphers at pleasure, as decimals, in the numerator, and divide by the denominator according to the rule for the division of decimals. The truth of this rule is evident from Art. 10.

[page] 21

Ex. 1. 3/4 = 3.00/4 = .75.

Ex. 2. 7/8 = 7.000/8 = .875.

Ex. 3. 4/625 = 4.0000/625 = .0064.

Ex. 4. 1/3 = 1.000 &c./3 = .333 &c.

Ex. 5. 4/33 = 4.0000 &c./33 = .1212 &c.

(44.) In some cases, as in the two last examples, the vulgar fraction cannot exactly be made up of tenths, hundredths, &c. but the decimal will go on without ever coming to an end, the same figure or figures recurring in the same order; but though we cannot represent the exact value of the vulgar fraction, yet, by increasing the number of decimal places, we may approach to it as near as we please. Thus, 1/9 = .1111 &c. now.1, or 1/10, is less than the true value by 1/90;.11, or 11/100, is too little by 1/900; &c.

Decimals of this kind are called recurring, or circulating decimals.

(45.) To find the value of a decimal of one denomination in terms of a lower denomination.

This may be done by the rule laid down in Art. 22.

[page] 22

Ex. Required the value of.615625£.

The value required is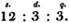

First,.615625£. = 12.3125 shillings.

Next,.3125s. = 3.75. pence.

Lastly,.75d. = 3. farthings.

(46.) To reduce a quantity to a decimal of a Superior denomination.

Divide the quantity by the number of integers of it's denomination contained in one of the superior denomination, and the quotient is the decimal required.

Ex. 1. What decimal of a shilling is three-pence?

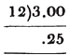Answ.

For, in the denomination shillings, it's numerical value must be 1/12 of it's value in the denomination pence.

Ex. 2. What decimal of a pound is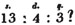

[page] 23

First, we find what decimal of a penny is; this, by the rule, is.75; then, what decimal of a shilling 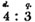or 4.75d. is; this is found in the same manner to be.3958333 &c. lastly, we find, by the same rule, what decimal of a pound 13.3958333 &c. sh. is; which appears to be .66979166 &c.

The conclusion will be the same if we reduce the quantity to a vulgar fraction (Art. 23), and this fraction to a decimal (Art. 43).

The proofs of the rules for the management of vulgar and decimal fractions, here given, are necessarily confined to particular instances, but the same reasoning may be applied in every case; and by using general signs, the proofs may be made general.

[page 24]

THE

ELEMENTS OF ALGEBRA.

PART I.

DEFINITIONS AND EXPLANATION OF SIGNS.

(47.) THE method of representing the relation of abstract quantities by letters and characters, which are made the signs of such quantities and their relations, is called Algebra.

Known or determined quantities are usually represented by the first letters of the alphabet, a, b, c, d, &c. and unknown or undetermined quantities, by the last, y, x, w, &c.

The following signs are made use of to express the relations which the quantities bear to each other.

(48.) + Plus, signifies that the quantity to which it is prefixed must be added. Thus, a + b signifies that the quantity represented by b is to be added to the quantity represented by a; if a represent 5, and b, 7, then a + b represents 12.

If no sign be placed before a quantity, the sign + is understood. Thus, a signifies + a. Such quantities are called positive quantities.

(49.) - Minus, signifies that the quantity to which

[page] 25

it is prefixed must be subtracted. Thus, a - b signifies that b must be taken from a; if a be 7, and b, 5, a - b expresses 7 diminished by 5, or 2.

Quantities to which the sign - is prefixed are called negative quantities.

(50.)×Into, signifies that the quantities between which it stands are to be multiplied together. Thus, a×b signifies that the quantity represented by a is to be multiplied by the quantity represented by b*.

This sign is frequently omitted; thus abc signifies a×b×c. Or a full point is used instead of it; thus 1×2×3, and 1.2.3, signify the same thing.

(51.) If in multiplication the same quantity be repeated any number of times, the product is usually expressed by placing, above the quantity, the number which represents how often it is repeated; thus a, a×a, a×a×a, a×a×a×a, and a¹, a², a³, a⁴, have respectively the same signification. These quantities are called powers; thus a¹, is called the first power of a; a², the second power, or square of a; a³, the third power, or cube of a, &c.

The numbers 1, 2, 3, &c. are called the indices of a; or exponents of the powers of a.

(52.) ÷ Divided by, signifies that the former of the quantities between which it is placed is to be divided by the latter. Thus, a ÷ b signifies that the quantity a is to be divided by b.

The division of one quantity by another is frequently represented by placing the dividend over the

* By quantities, we understand such magnitudes as can be represented by numbers; we may therefore without impropriety speak of the multiplication, division, &c. of quantities by each other.

[page] 26

divisor with a line between them, in which case the expression is called a fraction. Thus, a/b signifies a divided by b; and a is the numerator, and b the denominator of the fraction; also, 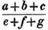siginifies that a, b, and c added together, are to be divided by e, f, and g added together; see Art. 10.

(53.) A quantity in the denominator of a fraction is also expressed by placing it in the numerator, and prefixing the negative sign to it's index; thus, a^{- 1}, a^{- 2}, a^{- 3}, a^{- n}, signify 1/a¹, 1/a², 1/a³, 1/aⁿ, respectively; these are called the negative powers of a.

(54.) The sign ~ between two quantities signifies their difference. Thus, a ~ x, is a - x or x - a, according as a or x is the greater; and a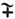x signifies the sum or difference of a and x.

(55.) A line drawn over several quantities signifies that they are to be taken collectively, and it is called a vinculum. Thus, 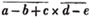signifies that the quantity represented by a - b + c is to be multiplied by the quantity represented by d - e. Let a stand for 6; b, 5; c, 4; d, 3; and e, 1; then a - b + c is 6 - 5 + 4, or 5; and d - e is 3 - 1, or 2; therefore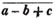 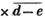is 5×2 or 10. 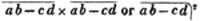signifies that the quantity represented by ab - cd is to be multiplied by itself.

(56.) = Equal to, signifies that the quantities between which it is placed are equal to each other; thus,

[page] 27

ax - by = cd + ad, signifies that the quantity ax - by is equal to the quantity cd + ad.

(57.) The square root of any proposed quantity is that quantity whose square, or second power, gives the proposed quantity. The cube root, is that quantity whose cube gives the proposed quantity, &c.

The signs √, 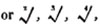&c. are used to express the square, cube, biquadrate, &c. roots of the quantities before which they are placed.

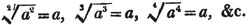
These roots are also represented by the fractions 1/2, 1/3, 1/4, &c. placed a little above the quantities, to the right. Thus, a^1/2, a^1/3, a^1/4 a^1/n, represent the square, cube, fourth and n^th root of a, respectively; a^5/2, a^7/3, a^3/5, represent the square root of the fifth power, the cube root of the seventh power, the fifth root of the cube of a.

(58.) If these roots cannot be exactly determined, the quantities are called irrational, or surds.

(59.) Points are made use of to denote proportion thus, a : b :: c : d, signifies that a bears the same proportion to b that c bears to d.

(60.) The number prefixed to any quantity, and which shews how often it is to be taken, is called it's coefficient. Thus, in the quantities 7ax, 6by, 3dz, 7, 6 and 3 are called the coefficients of ax, by, and dz, respectively.

When no number is prefixed, the quantity is to be taken once, or the coefficient 1 is understood.

[page] 28

These numbers are sometimes represented by letters, which are called coefficients.

(61.) Similar, or like algebraical quantities, are such as differ only in their coefficients; 4a, 6ab, 9a², 3a²bc, are respectively similar to 15a, 3ab, 12a², 15a²bc, &c.

Unlike quantities are different combinations of letters; thus, ab, a²b, ab², abc, &c. are unlike.

(62.) A quantity is said to be a multiple of another, when it contains it a certain number of times exactly; thus, l6a is a multiple of 4a, as it contains it exactly four times.

(63.) A quantity is called a measure of another, when the former is contained in the latter a certain number of times exactly; thus, 4a is a measure of l6a.

(64.) When two numbers have no common measure but unity, they are said to be prime to each other.

(65.) A simple algebraical quantity is one which consists of a single term, as a²bc.

(66.) A binomial is a quantity consisting of two terms, as a + b, or 2a - 3bx. A trinomial is a quantity consisting of three terms, as 2a + bd + 3c.

The following examples will serve to illustrate the method of representing quantities algebraically.

Let a = 8, b = 7, c = 6, d = 5and e = 1; then,

3a - 2b + 4c - e = 24 - 14 + 24 - 1 = 33.

ab + ce - bd = 56 + 6 - 35 = 27.

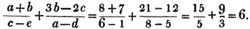
d²×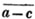 - 3ce² + d³ = 25×2 - 18 + 125 = 50 - 18 + 125 = 157.

[page] 29

AXIOMS.

(67.) If equal quantities be added to equal quantities, the sums will be equal.

(68.) If equal quantities be taken from equal quantities, the remainders will be equal.

(69.) If equal quantities be multiplied by the same, or equal quantities, the products will be equal.

(70.) If equal quantities be divided by the same, or equal quantities, the quotients will be equal.

(71.) If the same quantity be added to and subtracted from another, the value of the latter will not be altered.

(72.) If a quantity be both multiplied and divided by another, it's value will not be altered.

ADDITION OF ALGEBRAICAL QUANTITIES.

(73.) The addition of algebraical quantities is performed by connecting those that are unlike with their proper signs, and collecting those that are similar into one sum.

Ex. 1. Add together the following unlike quantities;

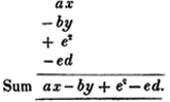
[page] 30

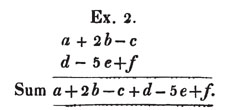
It is immaterial in what order the quantities are set down, if we take care to prefix to each it's proper sign.

When any terms are similar, they may be incorporated, and the general expression for the sum shortened.

1^st. When similar quantities have the same sign, their sum is found by taking the sum of the coefficients with that sign, and annexing the common letters.

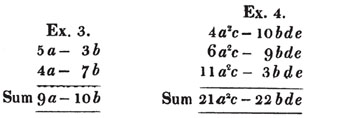
The reason is evident; 5a to be added, together with 4a to be added, makes 9a to be added; and 3b to be subtracted, together with 7b to be subtracted, is 10b to be subtracted.

2^d. If similar quantities have different signs, their sum is found by taking the difference of the coefficients with the sign of the greater, and annexing the common letters as before.

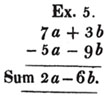
[page] 31

In the first part of the operation we have 7 times a to add, and 5 times a to take away; therefore upon the whole we have 2a to add. In the latter part, we have 3 times b to add, and 9 times b to take away; i. e. we have upon the whole 6 times b to take away; and thus the sum of all the quantities is 2a - 6b.

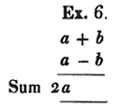
If several similar quantities are to be added together, some with positive and some with negative signs, take the difference between the sum of the positive, and the sum of the negative coefficients, prefix the sign of the greater sum, and annex the common letters.

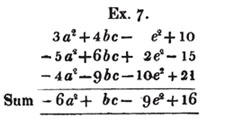
The method of reasoning in this case is the same as in the last example.

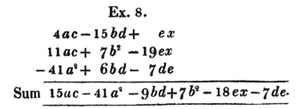
[page] 32

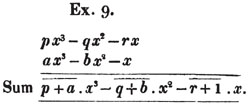
In this example, the coefficients of x and its powers are united; 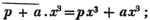also - 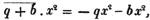because the negative sign affects the whole quantity under the vinculum; and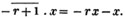

SUBTRACTION.

(74.) Subtraction, or the taking away of one quantity from another, is performed by changing the sign of the quantity to be subtracted, and then adding it to the other by the rules laid down in Art. 73.

Ex. 1.

From 2bx take cy, and the difference is properly represented by 2bx - cy; because the - prefixed to cy, shews that it is to be subtracted from the other; and 2bx - cy is the sum of 2bx and - cy, Art. 73.

Ex. 2.

Again, from 2bx take - cy, and the difference is 2bx + cy; because 2bx = 2bx + cy - cy Art. 71, take away - cy from these equal quantities, and the differences will be equal; i. e. the difference between 2bx and - cy is 2bx + cy, the quantity which arises from adding + cy to 2bx.

[page] 33

In this example the coefficients are united; 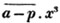is equal to ax³ - px³; 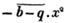is equal to - bx² + qx²; and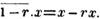

MULTIPLICATION.

(75.) The multiplication of simple algebraical quantities must be represented according to the notation pointed out Art. 50.

C

[page] 34

Thus, a×b, or ab, represents the product of a Multiplied by b; abc, the product of the three quantities a, b and c.

It is also indifferent in what order they are placed, a×b and b×a being equal.

For, 1×a = a×1, or 1 taken a times is the same with a taken once; also, b taken a times, or b×a, is b times as great as 1 taken a times; and a taken b times, or a×b, is b times as great as a taken once; therefore (Art. 69.) b×a = a×b. Also, abc = cab = bca = acb, &c. for, as in the former case, 1×a×b = a×b×1; and c×a×b is c times as great as 1×a×b; also a×b×c is c times as great as a×b×1; therefore a×b×c = c×a×b (Art. 69.); and a similar proof may be applied to the other cases.

(76.) To determine the sign of the product, observe the following rule:

If the multiplier and multiplicand have the same sign, the product is positive; if they have different signs, it is negative.

1^st. + ax + b = + ab; because in this case a is to be taken positively b times; therefore the product ab must be positive.

2^d. - ax + b = - ab; because - a is to be taken b times; that is, we must take - ab.

3^d. + ax - b = - ab; for a quantity is said to be multiplied by a negative number - b, if it be subtracted b times; and a subtracted b times is - ab. This also appears from Art. 79. Ex. 2.

4^th. - ax - b = + ab. Here - a is to be subtracted b times; that is, - ab is to be subtracted; but subtracting ab is the same as adding + ab (Art. 74.); therefore we have to add + ab.

[page] 35

The 2^d and 4^th cases may be thus proved; a - a = 0, multiply both sides by b, and ab together with - a×- b must be equal to b×0, or nothing; therefore - a multiplied by b must give - ab, a quantity which when added to ab makes the sum nothing.

Again, a - a = 0; multiply both sides by - b, then - ab together with - a×- b must be = 0; therefore - a×- b = + ab.

(77.) If the quantities to be multiplied have coefficients, these must be multiplied together as in common arithmetic; the sign and the literal product being determined by the preceding rules.

Thus, 3a×5b = l5ab; because 3×a×5×6 = 3×5×a×b = 15ab (Art. 75); 4x×- 11y = - 44xy; - 9b×- 5c = + 45bc; - 6d×4m = - 24md.

(78.) The powers of the same quantity are multiplied together by adding the indices; thus, a²×a³ = a⁵; for aa×aaa = aaaaa. In the same manner, a^m×aⁿ = a^{m + n}; and - 3a²x³×5axy² = - 15a³x⁴y²

(79.) If the multiplier or multiplicand consist of several terms, each term of the latter must be multiplied by every term of the former, and the sum of all the products taken, for the whole product of the two quantities.

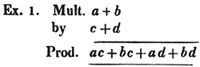
Here a + b is to be added to itself c + d times, i. e. c times and d times.

C 2

[page] 36

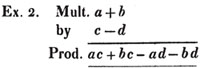
Here a + b is to be taken c - d times; that is, c times wanting d times; or c times positively and d times negatively.

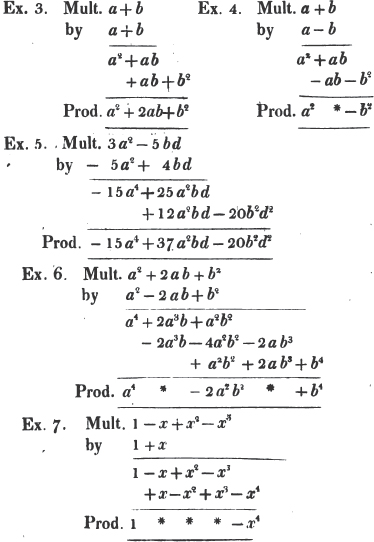
[page] 37

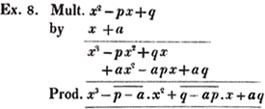
Here the coefficients of x² and x are collected;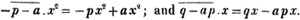

SCHOLIUM.

(80.) The method of determining the sign of a product from the consideration of abstract quantities, has been found fault with by some algebraical writers, who contend that - a, without reference to other quantities, is imaginary, and consequently not the object of reason or demonstration. In answer to this objection we may observe, that whenever we make use of the notation - a, and say it signifies a quantity to be subtracted, we make a tacit reference to other quantities.

Thus, in numbers, - a represents a number to be subtracted from those with which it is connected; and when we suppose - a to be taken b times, we must understand that a is to be taken a times from some other numbers. In estimating lines, or distances, - a represents a line, or distance, in a particular direction. The negative sign does not render quantities imaginary, or impossible, but points out the relation of real quantities to others with which they are concerned.

[page] 38

DIVISION.

(81.) To divide one quantity by another, is to determine how often the latter is contained in the former, or what quantity multiplied by the latter will produce the former.

Thus, to divide ab by a is to determine how often a must be taken to make up ab; that is, what quantity multiplied by a will give ab; which we know is b. From this consideration are derived all the rules for the division of algebraical quantities.

(82.) If the divisor and dividend be affected with like signs, the sign of the quotient is +: but if their signs be unlike, the sign of the quotient is -.

If - ab be divided by - a, the quotient is + b; because - ax + b gives - ab; and a similar proof may be given in the other cases.

(83.) In the division of simple quantities, if the coefficient and literal product of the divisor be found in the dividend, the other part of the dividend, with the sign determined by the last rule, is the quotient.

Thus, abc/ab = c; because ab multiplied by c gives abc.

If we first divide by a, and then by b, the result will be the same: for abc/a = bc, and bc/b = c, as before.

(84.) COR. Hence, any power of a quantity is divided by any other power of the same quantity, by taking the index of the divisor from the index of the dividend.

Thus, a⁵/a³ = a²; a³/a⁶ = 1/a³ = a^{- 3} (Art. 53); a^m/aⁿ = a^{m - n}.

[page] 39

(85.) If only a part of the product which forms the divisor, be contained in the dividend, the division must be represented according to the direction in Art. 52, and the quantities contained both in the divisor and dividend expunged.

Thus, 15a³b²c divided by - 3a²bx,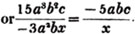

First, divide by - 3a²b, and the quotient is - 5abc; this quantity is still to be divided by x (Art. 83.), and as x is not contained in it, the division can only be represented in the usual way; that is, - 5abc/x is the quotient.

(86.) If the dividend consist of several terms, and the divisor be a simple quantity, every term of the dividend must be divided by it.

Thus,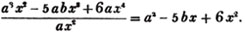

(87.) When the divisor also consists of several terms, arrange both the divisor and dividend according to the powers of some one letter contained in them; then, find how often the first term of the divisor is contained in the first term of the dividend, and write down this quantity for the first term in the quotient; multiply the whole divisor by it, subtract the product from the dividend, and bring down to the remainder as many other terms of the dividend as the case may require, and repeat the operation till all the terms are brought down.

Ex. 1.

If a² - 2ab + b² be divided by a - b, the operation will be as follows:

[page] 40

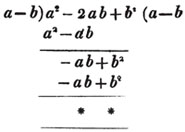
The reason of this, and the foregoing rule, is, that as the whole dividend is made up of all it's parts, the divisor is contained in the whole, as often as it is contained in all the parts. In the preceding operation we inquire first, how often a is contained in a², which gives a for the first term of the quotient, then multiplying the whole divisor by it, we have a² - ab to be subtracted from the dividend, and the remainder is - ab + b², with which we are to proceed as before.

The whole quantity a² - 2ab + b², is in reality divided into two parts by the process, each of which is divided by a - b, therefore the true quotient is obtained.

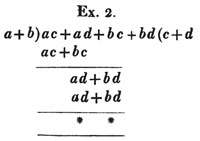
[page] 41

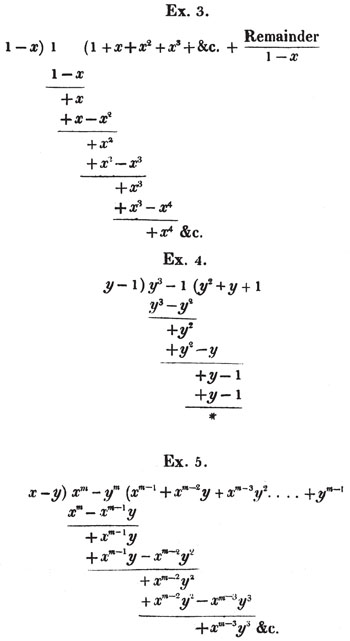
[page] 42

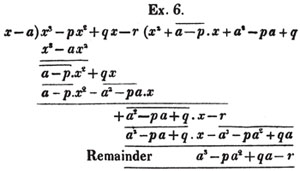
ON THE TRANSFORMATION OF FRACTIONS TO OTHERS OF EQUAL VALUE.

(88.) If the signs of all the terms both in the numerator and denominator of a fraction be changed, it's value will not be altered. For - ab/- a = + b = + ab/+ a; and ab/- a = - b = - ab/a.

(89.) If the numerator and denominator of a fraction be both multiplied, or both divided by the same quantity, its value is not altered.

For ac/bc = a/b (Art. 85).

Hence, a fraction is reduced to it's lowest terms, by dividing both the numerator and denominator by the greatest quantity that measures them both.

(90.) The greatest common measure of two quantities is found by arranging them according to the powers of some letter, and then dividing the greater by the less, and the preceding divisor always by the last remainder, till the remainder is nothing; the last divisor is the greatest common measure required.

[page] 43

Let a and b be the two quantities, and let b be contained in a, p times, with a remainder c; again, let c be contained in b, q times with a remainder d, and so on, till nothing remains; let d be the last divisor, and it will be the greatest common measure of a and b.

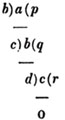
(91.) The truth of this rule depends upon these two principles;

1^st. If one quantity measure another, it will also measure any multiple of that quantity. Let x measure y by the units in n, then it will measure cy by the units in nc.

2^d. If a quantity measure two others, it will measure their sum or difference. Let a be contained in x, m times, and in y, n times; then ma = x and na = y; therefore 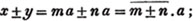i. e. a is contained in x ± y, m ± n times, or it measures x ± y by the units in m ± n.

(92.) Now it appears from the operation (Art. 90.), that a - pb = c, and b - qc = d; every quantity, therefore, which measures a and b, measures pb, and a - pb, or c; hence also it measures qc, and b - qc, or d; that is, every common measure of a and b measures d.

It appears also from the division, that a = pb + c, b = qc + d, c = rd; therefore d measures c, and qc, and qc + d or b; hence it measures pb, and pb + c, or a. Every common measure then of a and b measures d, and d measures a and b; therefore d is their greatest common measure.

Ex.

To find the greatest common measure of a⁴ - x⁴ and a³ - a²x - ax² + x³, and to reduce 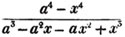to it's lowest terms.

[page] 44

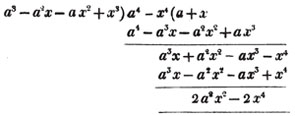
leaving out 2x², which is found in each term of the remainder, the next divisor is a² - x².

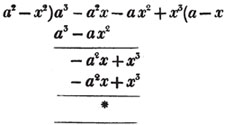
a² - x² is, therefore, the greatest common measure of the two quantities, and if they be respectively divided by it, the fraction is reduced to 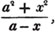it's lowest terms.

The quantity 2x², found in every term of one of the divisors, 2a² - 2x⁴, but not in every term of the dividend, a³ - a²x - ax³ - x³, must be left out; otherwise the quotient will be fractional, which is contrary to the supposition made in the proof of the rule; and by omitting this part, 2x², no common measure of the divisor and dividend is left out; because, by the supposition, no part of 2x² is found in all the terms of the dividend.

(93.) To find the greatest common measure of three quantities, a, b, c; take d the greatest common measure of a and b; and the greatest measure of d and c, is the greatest common measure required.

[page] 45

Because every common measure of a, b and c, measures d and c; and every measure of d and c measures a, b and c (Art. 92); therefore, the greatest common measure of d and c must be the greatest common measure of a, b and c.

(94.) In the same manner, the greatest common measure of four or more quantities may be found.

The greatest common measure of four quantities, a, b, c, d, may also be found by taking x the greatest common measure of a and b, and y the greatest common measure of c and d; then the greatest common measure of x and y will be the common measure required.

(95.) If one number be divided by another, and the preceding divisor by the remainder, according to Art. 90, the remainder will at length be less than any quantity that can be assigned.

For a = pb + c; and b, and consequently pb, is greater than c; therefore pb + c, or a, is greater than 2c, and a/2 is greater than c; therefore from a, a quantity greater than it's half has been taken; in the same manner, when c is the dividend, more than it's half is taken away, and so on: but if from any quantity there be taken more than it's half, and from the remainder more than it's half, and so on, there will, at length, remain a quantity less than any that can be assigned (Euc. 1. x).

(96.) Fractions are changed to others of equal value with a common denominator, by multiplying each numerator by every denominator except it's own, for the new numerator; and all the denominators together for the common denominator.

[page] 46

Let a/b, c/d, e/f be the proposed fractions; then adf/bdf, cbf/bdf, edb/bdf, are fractions of the same value with the former, having the common denominator bdf. For adf/bdf = a/b; cbf/bdf = c/d; and edb/bdf = e/f (Art. 89); the numerator and denominator of each fraction having been multiplied by the same quantity, vis. the product of the denominators of all the other fractions.

(97.) When the denominators of the proposed fractions are not prime to each other, find their greatest common measure; multiply both the numerator and denominator of each fraction; by the denominators of all the rest, divided respectively by their greatest common measure; and the fractions will be reduced to a common denominator in lower terms* than they would have been by proceeding according to the former rule.

Thus, a/mx, b/my, c/mz reduced to a common denominator, are ayz/mxyz; bxz/mxyz; cxy/mxyz.

ON THE ADDITION AND SUBTRACTION OF FRACTIONS.

(98.) If the fractions to be added have a common denominator, their sum is found by adding the numerators together and retaining the common denominator.

* To obtain them in die lowest terms, each must be reduced to another of equal value, with the denominator which is the least common multiple of all the denominators. See Art. 374.

[page] 47

Thus, 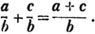This follows from the principle laid down in Art.87.

(99.) If the fractions have not a common denominator they must be transformed to others of the same value, which have a common denominator (Art. 96), and then the addition may take place as before.

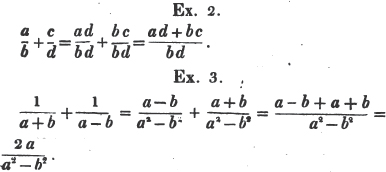
Ex.4

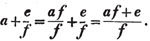Here a is considered as a fraction whose denominator is unity.

Ex. 5.

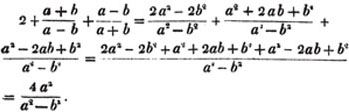
(100.) If two fractions have a common denominator, their difference is found by taking the difference of the numerators and retaining the common denominator.

Thus, 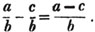(See Art. 87).

[page] 48

(101.) If they have not a common denominator, they must be transformed to others of the same value, which have a common denominator, and then the subtraction may take place as before.

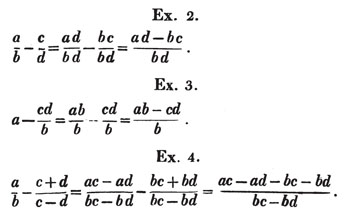
The sign of bd is negative, because every part of the latter fraction is to be taken from the former.

ON THE MULTIPLICATION AND DIVISION OF FRACTIONS.

(102.) To multiply a fraction by any quantity, multiply the numerator by that quantity and retain the denominator.

Thus, a/b×c = ac/b. For if the quantity to be divided be c times as great as before, and the divisor the same, the quotient must be c times as great.

[page] 49

(103.) COR 1. a/b×b = ab/b = a. That is, if a fraction be multiplied by it's denominator, the product is the numerator.

(104.) COR. 2. The result is the same, whether the numerator be multiplied by a given quantity, or the denominator divided by it. Let the fraction be ad/bc, and let it's numerator be multiplied by c, the result is adc/bc, or ad/b (Art. 89.), the quantity which arises from the division of it's denominator by c.

(105.) The product of two fractions is found by multiplying the numerators together for a new numerator, and the denominators for a new denominator.

Let a/b and c/d be the two fractions; then a/b×c/d = ac/bd. For if a/b = x, and c/d = y, by multiplying the equal quantities a/b and x, by b, a = bx (Art. 69); in the same manner, c = dy; therefore, by the same axiom, ac = bdxy; dividing these equal quantities, ac/bd = xy = a/b×c/d.

(106.) To divide a fraction by any quantity, multiply the denominator by that quantity, and retain the numerator.

The fraction a/b divided by c, is a/bc. Because a/b = ac/bc,

D

[page] 50

and a c^th of this a/bc; the quantity to be divided being a c^th part of what it was before, and the divisor the same.

(107.) COR. The result is the same, whether the denominator is multiplied by the quantity, or the numerator divided by it.

Let the fraction be ac/bd, if the denominator be multiplied by c, it becomes ac/bdc or a/bd; the quantity which arises from the division of the numerator by c.

(108.) To divide one fraction by another, invert the numerator and denominator of the divisor, and proceed as in multiplication.

Let a/b and c/d be the two fractions, then a/b ÷ c/d = a/b×d/c = ad/bc.

For if a/b = x, and c/d = y then as in Art. 105, a = bx, and c = dy; also, ad = bdx, and bc = bdy; therefore by Art. 70, ad/bc = bdx/bdy = x/y = a/b ÷ c/d.

(109.) The rule for multiplying the powers of the same quantity (Art. 78), will hold when one or both of the indices are negative.

Thus, a^m×a^{- n} = a^{m - n}; for a^m×a^{- n} = a^m×1/aⁿ (Art. 53.) = a^m/aⁿ = a^{m - n}; in the same manner, x³×x^{- 5} = x³/x⁵ = 1/x² = x^{- 2}.

[page] 51

Again, a^{- m}×a^{- n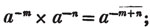} = because a^{- m}×a^{- n} = 1/a^m×1/aⁿ (Art. 53.), = 1/ a^{m + n} =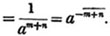

(110.) COR. If m = n, a^m×a^{- m} = a^{m - m} = a⁰; also, a^m×a^{- m} = a^m/a^m = 1; therefore a⁰ = 1; according to the notation adopted (Arts. 51. 53).

(111.) The rule for dividing any power of a quantity by any other power of the same quantity (Art. 84.) holds, whether those powers are positive or negative.

Thus, a^m ÷ a^{- n} = a^m ÷ 1/aⁿ (Art. 53), = a^m×aⁿ = a^{m + n}. Again, a^{- m} ÷ a^{- n} = 1/a^m ÷ 1/aⁿ = aⁿ/a^m (Art. 108.) = a^{n - m} (Art. 84).

(112.) COR. Hence it appears, that a quantity may be transferred from the numerator of a fraction to the denominator, and the contrary, by changing the sign of it's index. Thus,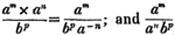

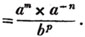
ON INVOLUTION AND EVOLUTION.

(113.) If a quantity be continually multiplied by itself, it is said to be involved, or raised; and the power to which it is raised, is expressed by the number of times the quantity has been employed in the multiplication.

D 2.

[page] 52

Thus, a×a, or a², is called the second power of a; a×a×a, or a³, the third power; a×a…. (n), or aⁿ, the n^th power.

(114.) If the quantity to be involved be negative, the signs of the even powers will be positive, and the signs of the odd powers negative.

For - ax - a = a²; - ax - ax - a = a³, &c.

(115.) A simple quantity is raised to any power, by multiplying the index of every factor in the quantity by the exponent of the power, and prefixing the proper sign determined by the last article.

Thus, a^m raised to the n^th power is a^mn. Because a^m×a^m×a^m… to n factors, by the rule of multiplication, is a^mn; also, 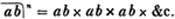to n factors, or a×a×a…. to n factors×b×b×b…. to n factors (Art. 75), = aⁿ×bⁿ; and a²b³c raised to the fifth power is a¹⁰b¹⁵c⁵. Also, - a^m raised to the n^th power is ± a^mn; where the positive or negative sign is to be prefixed, according as n is an even or odd number.

(116.) If the quantity to be involved be a fraction, both the numerator and denominator must be raised to the proposed power (Art. 105).

(117.) If the quantity proposed be a compound one, the involution may either be represented by the proper index, pr it may actually take place.

[page] 53

Let a + b be the quantity to be raised to any power.

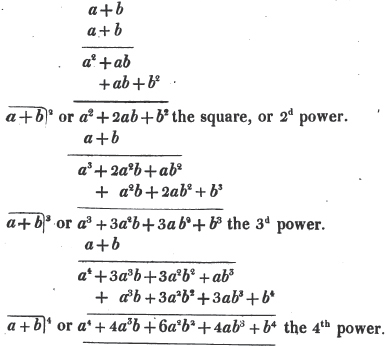
If b be negative, or the quantity to be involved be a - b, wherever an odd power of b enters, the sign of the term must be negative (Art. 114.)

Hence,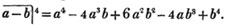

(118.) Evolution, or the extraction of roots, is the method of determining a quantity which raised to a proposed power will produce a given quantity.

(119.) Since the n^th power of a^m is a^mn, the n^th root of a^mn must be a^m; i. e. to extract any root of a single quantity, we must divide the index of that quantity by the index of the root required.

(120.) When the index of the quantity is not exactly divisible by the number which expresses the root to be extracted, that root must be represented

[page] 54

according to the notation pointed out in Art. 57. Thus, the square, cube, fourth, n^th root of a² + x², are respectively represented by 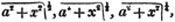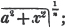the same roots of 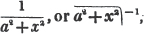are represented by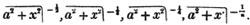

(121.) If the root to be extracted be expressed by an odd number, the sign of the root will be the same with the sign of the proposed quantity, as appears by Art. 114.

(122.) If the root to be extracted be expressed by an even number, and the quantity proposed be positive, the root may be either positive or negative. Because either a positive or negative quantity, raised to such a power, is positive (Art. 114).

(123.) If the root proposed to be extracted be expressed by an even number, and the sign of the proposed quantity be negative, the root cannot be extracted; because no quantity, raised to an even power, can produce a negative result. Such roots are called impossible.

(124.) Any root of a product may be found by taking that root of each factor, and multiplying the roots, so taken, together.

Thus, 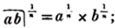because each of these quantities, raised to the n^th power, is ab (Art. 115).

COR. If a = b, then a^1/n×a^1/n = a^2/n; and in the same manner,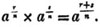

[page] 55

(125.) Any root of a fraction may be found by taking that root of both the numerator and denominator (Art. 116).

Thus, the cube root of a²/b² is a^2/3/b^2/3, or a^2/3×b^{- 2/3}; and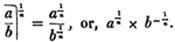

(126.) To extract the square root of a compound quantity.

Since the square root of a² + 2ab + b² is a + b (Art. 117), whatever be the values of a and b, we may obtain a general rule for the extraction of the square root, by observing in what manner a and b may be derived from a² + 2ab + b².

Having arranged the terms according to the dimensions of one letter, a, the square root of the first a², is a, the first factor in the root; subtract its square from the whole quantity; and bring down the remainder 2ab + b²; divide 2 ab by 2 a, and the result is b, the other factor in the root; then multiply the sum of twice the first factor and the second (2a + b), by the second (b), and subtract this product (2ab + b²) from the remainder. If there be more terms, consider a + b as a new value of a; and it's square, that is a² + 2ab + b², having, by the first part of the process, been subtracted from the proposed quantity, divide the remainder by the double of this new value of a, for a new factor in the root; and for a new subtrahend, multiply this factor by

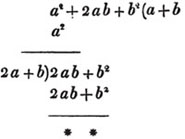
[page] 56

twice the sum of the former factors increased by this factor. The process must be repeated till the root, or the necessary approximation to the root, is obtained.

Ex. 1.

To extract the square root of a² + 2ab + b² + 2ac + 2bc + c²; or of its equal

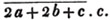
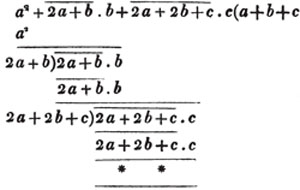
Ex. 2.

To extract the square root of a² - ax + x²/4.

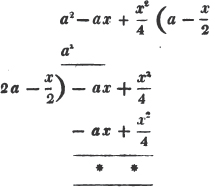
[page] 57

Ex. 3.

To extract the square root of 1 + x.

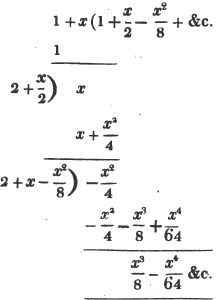
(127.) It appears from the second example, that a trinomial a² - ax + x²/4, in which four times the product of the first and last terms, is equal to the square of the middle term, is a complete square.

(128.) The method of extracting the cube root is discovered in the same manner.

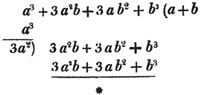
The cube root of a³ + 3a²b + 3ab² + b³ is a + b (Arts. 117, 118); and to obtain a + b from this compound quantity, arrange the terms as before, and the cube root of the first factor in the root of the first term, a³, is a the first factor in the root;

[page] 58

subtract it's cube from the whole quantity, and divide the first term of the remainder by 3a², the result is b, the second factor in the root; then subtract 3a²b + 3ab² + b³ from the remainder, and the whole cube of a + b has been subtracted. If any quantity be left, proceed with a + b as a new a, and divide the last remainder by 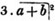for a third factor in the root; and thus any number of factors may be obtained.

SCHOLIUM.

(129.) The rules above laid down, for the extraction of the roots of compound quantities, are but little used in algebraical or fluxional operations; but it was necessary to give them at full length, for the purpose of investigating rules for the extraction of the square and cube roots in numbers.

The square root of 100 is 10, of 10000 is 100, of 1000000 is 1000, &c. from which consideration it follows, that the square root of a numberless than 100 must consist of only one figure, of a number between 100 and 10000 of two places of figures, of any number from 10000 to 1000000, of three places of figures, &c. If then a point be made over every second figure in any number, beginning with the units, the number of points will shew the number of figures, or places, in the square root. Thus the square root of 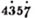consists of two figures, the square root of 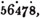of three figures, &c.

[page] 59

Let the square root of 4357 be required.

Having pointed it according to the direction, it appears that the root consists of two places of figures; let a + b be the root, where a is the value of the figure in the ten's place, and b, of that in the unit's; then is a the nearest square root of 4300 which does not exceed the true root, this appears to be 60; subtract the square of 60 (a²) from the given number, and the remainder is 757; divide this remainder by 120 (2a), and the quotient is 6 (the value of b), and the subtrahend, or quantity, to be taken from the last remainder 757, is 126×6, 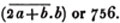or 756.

It is said that a must be the greatest number whose square does not exceed 4300: it evidently cannot be a greater number than this; and if possible let it be some quantity x, less than this; then since x is in the ten's place and b in the unit's, x + b is less than a; therefore the square of x + b, whatever be the value of b, must be less than a², and consequently x + b less than the true root.

If the root consist of three places of figures, let a represent the hundreds, and b the tens; then having obtained a and b as before, let the new value of a be the hundreds and tens together, and find a new value of b for the units: and thus the process may be continued when there are more places of figures in the root.

[page] 60

(130.) The cyphers being omitted for the sake of expedition, the following rule is obtained from the foregoing process.

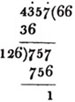
Point every second figure beginning with the unit's place, dividing by this process the whole number into several periods; find the greatest number whose square is contained in the first period, this is the first figure in the root; subtract it's square from the first period, and to the remainder bring down the next period; divide this quantity, omitting the last figure, by twice the part of the root already obtained, and annex the result to the root and also to the divisor; then multiply the divisor, as it now stands, by the part of the root last obtained, for the subtrahend. If there be more periods to be brought down, the operation must be repeated.

Ex. 2.

Let the square root of 611524 be required.

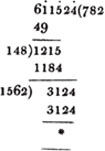
[page] 61

(131.) In extracting the square root of a decimal, the pointing must be made the contrary way, beginning with the place of hundredths, or care must be taken to have an even number of decimal places; because, if the root have 1, 2, 3, 4, &c. decimal places, the square must have 2, 4, 6, 8, &c. places (Art. 38).

Ex. 3.

To extract the square root of 64.853.

For every pair of cyphers which we suppose annexed to the decimal, another figure is obtained in the root.

(132.) The cube root of 1000 is 10, of 1000000 is 100, &c. therefore the cube root of a number less than 1000 consists of one figure, of any number between 1000 and 1000000, of two places of figures, &c. If then a point be made over every third figure contained in any number, beginning with the units, the number of points will shew the number of places in its cube root.

Let the cube root of 405224 be required.

[page] 62

By pointing the number according to the direction, it appears that the root consists of two places; let a be the value of the figure in the ten's place, and b, of that in the unit's. Then a is the greatest number whose cube is contained in 405000*, or 70; subtract it's cube from the whole quantity, and the remainder is 62224; divide this remainder by 3a², or 14700, and the quotient 4, or b, is the second term in the root: then subtract the cube of 74 from the original number, and as the remainder is nothing, 74 is the cube root required. Observe, that the cyphers may be omitted in the operation; and that as a³ was at first subtracted, if from the first remainder, 3a³b + 3ab² + b³ be taken, the whole cube of a + b will be taken from the original quantity.

(133.) In extracting the cube root of a decimal, care must be taken that the decimal places be three, or some multiple of three, before the operation is begun; because there are three times as many decimal places in the cube as there are in the root (Art. 38).

Ex. 2.

Required the cube root of 311897.91.

* See Art. 129.

[page] 63

The new value of a is 670, or, omitting the cypher, 67, and 3a², the new divisor, is 13467.. hence 8 is the next figure in the root; and

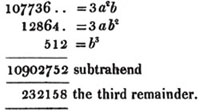
It appears from the pointing, that there is one decimal place in the root; therefore 67.8 is the root required, nearly. If three more cyphers be annexed to the decimal, another decimal place is obtained in the root; and thus approximation may be made to the true root of the proposed number, to any degree of accuracy.

Since the first remainder is 3a²b + 3 ab² + b³, the exact value of b is not obtained by dividing by 3a², and if upon trial the subtrahend be found to be greater than the first remainder, the value assumed for b is too great, and a less number must be tried. The

[page] 64

greater a is with respect to b, the more nearly is the true value obtained by division; and when a few places in the root are found, the number of figures may nearly be doubled, by division only.

ON SIMPLE EQUATIONS.

(134.) If one quantity be equal to another, or to nothing, and this equality be expressed algebraically, it constitutes an Equation.

Thus, x - a = b - x is an equation, of which x - a forms one side, and b - x the other.

(135.) When an equation is cleared of fractions and surds, if it contain the first power only of an unknown quantity, it is called a simple equation, or an equation of one dimension: if the square of the unknown quantity be in any term, it is called a quadratic, or an equation of two dimensions; and in general, if the index of the highest power of the unknown quantity be n, it is called an equation of n dimensions.

(136.) In any equation, quantities may be transposed from one side to the other, if their signs be changed, and the two sides will still be equal.

Let x + 10 = 15, then by subtracting 10 from each side, x + 10 - 10 = 15 - 10 (Art. 68), or x = 15 - 10.

Let x - 4 = 6, by adding 4 to each side, x - 4 + 4 = 6 + 4, or x = 6 + 4 (Art. 67).

If x - a + b = y; adding a - b to each side, x - a + b + a - b = y + a - b; or x = y + a - b.

(137.) COR. Hence, if the signs of all the terms on each side be changed, the two sides will still be equal.

[page] 65

Let x - a = b - 2x; by transposition, - b + 2x = x + a; or a - x = 2x - b.

(138.) If every term, on each side, be multiplied by the same quantity, the results will be equal (Art. 69).

(139.) COR. An equation may be cleared of fractions, by multiplying every term, successively, by the denominators of those fractions.

Let 3x + 5x/4 = 34; multiplying by 4, 12x + 5x = 136. (See Art. 103).

An equation may be cleared of fractions at once, by multiplying both sides by the product of all the denominators, or by any quantity which is a multiple of them all*.

Let x/2 + x/3 + x/4 = 13; multiplying by 2×3×4, 3×4×x + 2×4×x + 2×3×x = 2×3×4×13, or 12x + 8x + 6x = 312; that is, 26x = 312.

If each side be multiplied by 12, which is a multiple of 2, 3, and 4, the equation will become 12x/2 + 12x/3 + 12x/4 = 156; 6x + 4x + 3x = 156; that is, 13x = 156.

(140.) If each side of an equation be divided by the same quantity, the results will be equal.

Let 17x = 136; then x = 136/17 = 8 (Art. 70).

* If the least common multiple be made use of, the equation will be in the lowest terms.

E

[page] 66

(141.) If each side of an equation be raised to the same power, the results will be equal.

Let x^1/2 = 9; then x = 9×9 = 81 (Art. 69).

Also, if the same root be extracted on both sides, the results will be equal.

Let x = 81; then x^1/2 = 9 (Art. 118).

(142.) To find the value of an unknown quantity in a simple equation.

Let the equation first be cleared of fractions, then transpose all the terms which involve the unknown quantity to one side of the equation, and the known quantities to the other; divide both sides by the coefficient, or sum of the coefficients, of the unknown quantity, and the value required is obtained.

Ex. 1.

To find the value of x in the equation 3x - 5 = 23 - x.

by transp. 3x + x = 23 + 5 (Art. 136.)

or 4x = 28

by division x = 28/4 = 7 (Art. 140.)

Ex. 2.

Let x + x/2 - x/3 = 4x - 17.

Mult. by 2, and 2x + x - 2x/3 = 8x - 34.

Mult, by 3, and 6x + 3x - 2x = 24x - 102 (Art. 139).

by transp. 6x + 3x - 2x - 24x = - 102,

or - 17x = - 102

17x = 102 (Art. 137)

x = 102/17 = 6.

[page] 67

Ex. 3.

1/a + b/x = c.

1 + ba/x = ca

x + ba = cax

x - cax = - ba

or cax - x = ba (Art. 137)

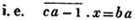
x = ba/ca - 1.

Ex. 4.

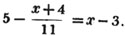
55 - x - 4 = 11x - 33

55 - 4 + 33 = 11x + x

84 = 12x

x = 84/12 = 7.

Ex. 5.

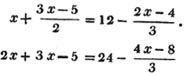
6x + 9x - 15 = 72 - 4x + 8

6x + 9x + 4x = 72 + 8 + 15

19x = 95

x = 95/19 = 5

E 2

[page] 68

(143.) If there be two independent simple equations involving two unknown quantities, they may be reduced to one, which involves only one of the unknown quantities, by any of the following methods:

1^st Method. In either equation, find the value of one of the unknown quantities in terms of the other and known quantities, and for it substitute this value in the other equation, which will then only contain one unknown quantity, whose value may be found by the rules before laid down.

Let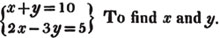

From the first equat. x = 10 - y; hence, 2x = 20 - 2y,

by subst. 20 - 2y - 3y = 5

20 - 5 = 2y + 3y

15 = 5y

y = 15/5 = 3

hence also, x = 10 - y = 10 - 3 = 7.

2^d Method. Find an expression for one of the unknown quantities, in each equation; put these expressions equal to each other, and from the resulting equation the other unknown quantity may be found.

Let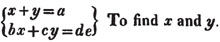

From the first equat. x = a - y

from the second, bx = de - cy, and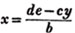

therefore,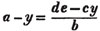

[page] 69

ba - by = de - cy

cy - by = de - ba

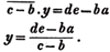
Also, x = a - y; that is,

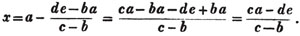
3^d Method. If either of the unknown quantities have the same coefficient in both equations, it may be exterminated by subtracting, or adding, the equations, according as the sign of the unknown quantity, in the two cases, is the same or different.

Let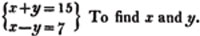

By subtraction, 2y = 8, and y = 4.

By addition, 2x = 22, and x = 11 (Art. 67).

If the coefficients of the unknown quantity to be exterminated be different, multiply the terms of the first equation by the coefficient of the unknown quantity in the second, and the terms of the second equation by the coefficient of the same unknown quantity, in the first; then add, or subtract, the resulting equations, as in the former case.

Ex. 1. Let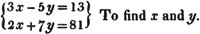

Multiply the terms of the first equation by 2, and the terms of the other by 3,

then 6x - 10y = 26

6x + 21y = 243

[page] 70

By subtraction, - 31y = - 217

and y = 217/31 = 7;

also, 3x - 5y = 13, or 3x - 35 = 13

therefore, 3x = 13 + 35 = 48

and x = 48/3 = 16.

Ex. 2.

Let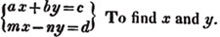

From the first, max + mby = mc

from the other, max - nay = ad

by subtraction, mby + nay = mc - ad

therefore,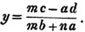

Again, nax + nby = nc

mbx - nby = bd

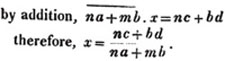
Ex. 3.

Let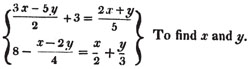

From the first equat.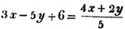

15x - 25y + 30 = 4x + 2y

15x - 4x - 25y - 2y = - 30

11x - 27y = - 30

[page] 71

from the second equat. 32 - x + 2y = 4x/2 + 4y/3 = 2x + 4y/3

96 - 3x + 6y = 6x + 4y

96 = 6x + 3x + 4y - 6y

or 9x - 2y = 96

and 11x - 27y = - 30

hence 99x - 22y = 1056

and 99x - 243y = - 270

221y = 1056 + 270 = 1326

y = 1326/221 = 6

also, 9x - 2y = 96

or 9x - 12 = 96

9x = 96 + 12 = 108

x = 108/9 = 12.

(144.) If there be three independent simple equations, and three unknown quantities, reduce two of the equations to one, containing only two of the unknown quantities, by the preceding rules; then reduce the third equation and either of the former to one, containing the same two unknown quantities; and from the two equations thus obtained, the unknown quantities which they involve may be found. The third quantity may be found by substituting their values in any of the proposed equations.

Ex.

Let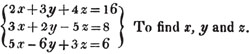

[page] 72

From the two first equat. 6x + 9y + 12z = 48

6x + 4y - 10z = 16

by subtr. 5y + 22z = 32

from the first and third, 10x + 15y + 20z = 80

10x - 12y + 6z = 12

by subtr. 27y + 14z = 68

and 5y + 22z = 32

hence 135y + 70z = 340

and 135y + 594z = 864

by subtr. 524z = 524

z = 1

5y + 22z = 32

that is, 5y + 22 = 32

5y = 32 - 22 = 10

y = 10/5 = 2

2x + 3y + 4z = 16

that is, 2x + 6 + 4 = 16

2x = 16 - 6 - 4 = 6

x = 3.

The same method may be applied to any number of simple equations.

(145.) That the unknown, quantities may have definite values, there must be as many independent equations as unknown quantities. When there are more equations than unknown quantities, the value of any one of these quantities may be determined from different equations; and should the values, thus found, differ, the equations are incongruous; should they be the same, one or more of the equations are unnecessary. When there are fewer equations than unknown quantities, one of these quantities cannot be

[page] 73

found, but in terms which involve some of the rest, whose values may be assumed at pleasure; and in such cases the number of answers is indefinite.

Thus, if x + y = a, then x = a - y; and assuming y at pleasure, we obtain a value of x, such, that x + y = a.

These equations must also be independent, that is, not deducible one from another.

Let x + y = a, and 2x + 2y = 2a; this latter equation being deducible from the former, it involves no different supposition, nor requires any thing more for it's truth, than that x + y = a should be a just equation.

PROBLEMS WHICH PRODUCE SIMPLE EQUATIONS.

(146.) From certain quantities which are known, to investigate others which have a given relation to them, is the business of Algebra.

When a question is proposed to be resolved, we must first consider fully it's meaning and conditions. Then substituting for such unknown quantities as appear most convenient, we must proceed as if they were already determined, and we wished to try whether they answer all the proposed conditions or not, till as many independent equations arise as we have assumed unknown quantities, which will always be the case if the question be properly limited (Art. 145); and by the solution of these equations, the quantities sought will be determined.

[page] 74

PROB. 1.

A bankrupt owes A twice as much as he owes B, and C as much as he owes A and B together; out of £.300, which is to be divided amongst them, what must each receive?

Let x represent what B must receive;

then 2x = what A must receive,

and x + 2x, or 3x, = what C must receive;

amongst them they receive £.300; therefore

x + 2x + 3x = 300

6x = 300

x = 300/6 = 50, what B must receive

2x = 100, what A must receive

3x = 150, what C must receive.

PROB. 2.

To divide a line of 15 inches into two such parts, that one may be three fourths of the other.

Let 4x = one part,

then 3x = the other.

7x = 15, by the question,

x = 15/7

4x = 60/7 = 8 4/7, one part,

3x = 45/7 = 6 3/7, the other.

PROB. 3.

If A can perform a piece of work in 8 days, and B in 10 days, in what time will they finish it together?

Let x be the time required.

[page] 75

In one day, A performs 1/8 part of the work; therefore in x days, he performs x/8 parts of it; and in the same time, B performs x/10 parts of it; and calling the work 1,

x/8 + x/10 = 1

10x + 8x = 80

18x = 80

x = 80/18 = 4 8/18 = 4 4/9 days.

PROB. 4.

A workman was employed for 60 days, on condition that for every day he worked he should receive 15 pence; and for every day he played he should forfeit 5 pence; at the end of the time he had 20 shillings to receive; required the number of days he worked.

Let x be the number of days he worked, then 60 - x is the number he played,

15x his pay, in pence,

300 - 5x, sum forfeited,

15x - 300 + 5x = 240, by the question,

20x = 240 + 300 = 540

x = 27, the days he worked,

60 - x = 33, the days he played.

PROB. 5.

How much rye, at four shillings and sixpence a bushel, must be mixed with 50 bushels of wheat, at

[page] 76

six shillings a bushel, that the mixture may be worth five shillings a bushel?

Let x be the number of bushels required;

then 9x is the price of the rye in sixpences 600 the price of the wheat

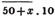the price of the mixture;

therefore, 9x + 600 = 500 + 10x

and 100 = x, the number of bushels required.

PROB. 6.

A and B engage together in play; in the first game, A wins as much as he had and four shillings more, and finds he has twice as much as B; in the second game, B wins half as much as he had at first and one shilling more, and then it appears that he has three times as much as A; what sum had each at first?

Let x be what A had, in shillings,

y what B had

2x + 4, what A has after the 1^st game

y - x - 4, what B has

by the question, 2x + 4 = 2y - 2x - 8

or 2y - 4x = 12

y - 2x = 6

also, y - x - 4 + y/2 + 1, what B has after the second game,

2x + 4 - y/2 - 1, what A has;

by the question, y - x - 4 + y/2 + 1 = 6x + 12 - 3y/2 - 3

or 2y - 2x - 8 + y + 2 = 12x + 24 - 3y - 6

hence 6y - 14x = 24

or 3y - 7x = 12

[page] 77

also, y - 2x = 6

therefore, 3y - 6x = 18

also, 3y - 7x = 12

by subtraction, x = 6

y - 2x = 6, or y - 12 = 6

y = 18.

PROB. 7.

A smuggler had a quantity of brandy which he expected would raise £9 : 18s.; after he had sold 10 gallons, a revenue officer seized one third of the remainder, in consequence of which he makes only £8 : 2s.; required the number of gallons he had, and the price per gallon.

Let x be the number of gallons;

then 198/x is the price per gallon, in shillings,

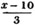the quantity seized,

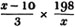the value of the quantity seized, which appears by the question to be 36 shillings;

therefore,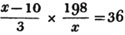

66x - 660 = 36x

30x = 660

x = 22 the number of gallons,

198/x = 198/22 = 9 shillings, the price per gallon.

PROB. 8.

A and B play at bowls, and A bets B three shillings

[page] 78

to two upon every game; after a certain number of games it appears, that A has won three shillings; but had he ventured to bet five shillings to two, and lost one game more out of the same number, he would have lost thirty shillings: how many games did they play?

Let x be the number of games A won,

y the number B won,

then 2x is what A won of B,

and 3y what B won of A.

2x - 3y = 3, by the question;

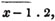A would win on the 2^d supposition,

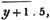B would win,

5y + 5 - 2x + 2 = 30, by the question,

or 5y - 2x = 30 - 5 - 2 = 23

therefore, 5y - 2x = 23

and 2x - 3y = 3

by addition, 5y - 3y = 26

2y = 26

y = 13

2x = 3 + 3y = 3 + 39 = 42

x = 21

x + y = 34, the number of games.

PROB. 9.

A sum of money was divided equally amongst a certain number of persons; had there been three more, each would have received one shilling less, and had there been two fewer, each would have received one shilling more than he did: required the number of persons, and what each received.

[page] 79

Let x be the number of persons,

y the sum each received, in shillings;

then xy is the sum divided,

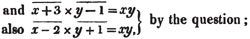
therefore, xy - x + 3y - 3 = xy

or - x + 3y = 3

and xy + x - 2y - 2 = xy

or x - 2y = 2

also, - x + 3y = 3

therefore, y = 5

hence x - 2y = x - 10 = 2.

or x = 12.

ON QUADRATIC EQUATIONS.

(147.) When the terms of an equation involve the square of the unknown quantity, but the first power does not appear, the value of the square is obtained by the preceding rules; and by extracting the square root on both sides, the quantity itself is found.

Ex. l.

Let 5x² - 45 = 0; to find x.

By trans. 5x² = 45

x² = 9

therefore (Art. 141), x = √9 = ± 3.

The signs + and - are both prefixed to the root, because the square root of a quantity may be either positive or negative (Art. 122). The sign of x may also be negative; but still x will be either equal to + 3 or - 3.

[page] 80

Ex. 2.

Let ax² = bcd; to find x.

x² = bcd/a

x = ± √bcd/a.

(148.) If both the first and second powers of the unknown quantity be found in an equation, arrange the terms according to the dimensions of the unknown quantity, beginning with the highest, and transpose the known quantities to the other side; then, if the square of the unknown quantity be affected with a coefficient, divide all the terms by this coefficient, and if it's sign be negative, change the signs of all the terms (Art. 137), that the equation may be reduced to this form, x² ± px = ± q. Then add to both sides the square of half the coefficient of the first power of the unknown quantity, by which means, the first side of the equation is made a complete square, and the other consists of known quantities; and by extracting the square root on both sides, a simple equation is obtained, from which the value of the unknown quantity may be found.

Ex. 1.

Let x² + px = q; now, we know that x² + px + p²/4 is the square of x + p/4 (Art. 127); add therefore, p²/4 to both sides, and we have

[page] 81

x² + px + p²/4 = q + p²/4 then by extracting the square root on both sides,

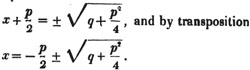
In the same manner, if x² - px = q, x is found to be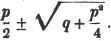

Ex. 2.

Let x² - 12x + 35 = 0; to find x.

By transposition, x² - 12x = - 35, and adding the square of 6 to both sides of the equation,

x² - 12x + 36 = 36 - 35 = l;

then extracting the square root on both sides,

x - 6 = ± l

x = 6 ± 1 = 7 or 5; either of which, substituted for x in the original equation, answers the condition, that is, makes the whole equal to nothing.

Ex. 3.

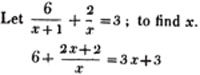
6x + 2x + 2 = 3x² + 3x

3x² - 5x = 2

x² - 5x/3 = 2/3

x² - 5x/3 + 25/36 = 25/36 + 2/3

F

[page] 82

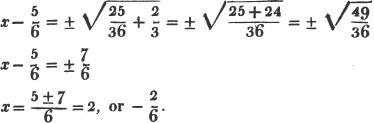
In this example, 25/36 and 2/3 are to be reduced to a common denominator, and since 36 is a complete square, the most convenient, method for the solution, is to multiply both the numerator and denominator of 2/3 by 12, that the common denominator may be a square number (Art. 20).

Ex. 4.

(149.) Let 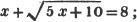to find x.

By transposition,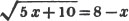

squaring both sides, 5x + 10 = 64 - 16x + x²

x² - 21x = 10 - 64 = - 54

completing the square, x² - 21x + 441/4 = 441/4 - 54

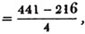or x² - 21x + 441/4 = 225/4

extracting the square root, x - 21/2 = ± 15/2

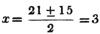or 18.

By this process two values of x are found; but on trial it appears, that 18 does not answer the condition of the equation, if we suppose that 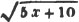represents the positive square root of

[page] 83

5x + 10. The reason is, that 5x + 10 is the square of 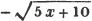as well as of 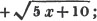thus by squaring both sides of the equation 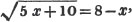a new condition is introduced, and a new value of the unknown quantity corresponding to it, which had no place before. Here, 18 is the value which corresponds to the supposition that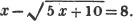

It should be particularly observed, that since + x x + y is equal to - x x - y, in the multiplication and involution of quantities, new values are always introduced, which, if not again excluded by the nature of the question, will appear in the final equation.

(150.) Every equation, where the unknown quantity is found in two terms, and it's index in one is twice as great as in the other, may be resolved in the same manner.

Ex. 5.

Let z + 4z^1/2 = 2l.

z + 4z^1/2 + 4 = 21 + 4 = 25

z^1/2 + 2 = ± 5

z^1/2 = ± 5 - 2 = 3, or -7.

therefore z = 9, or 49. (See Art. 149).

Ex. 6.

Let x^{- 1} + x^{- 1/2} = 6

x^{- 1}+ x^-1/2 + 1/4 = 6 + 1/4 = 25/4

x^{- 1/2} + 1/2 = ± 5/2

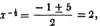or - 3

and x^{- 1/2} = 1/2, or - 1/3.

F 2

[page] 84

Ex. 7.

Let y⁴ - 6y² - 27 = 0.

y⁴ - 6y² = 27

y⁴ - 6y² + 9 = 27 + 9 = 36

y² - 3 = ± 6

y² = 3 ± 6 = 9, or -3

y = ± 3, or ± √-3.

Ex. 8.

Let y⁶ + ry³ + q³/27 = 0.

y⁶ + ry³ = - q³/27

y⁶ + ry³ + r²/4 = r²/4 - q³/27

y³ + r/2 = ± √r²/4 - q³/27.

y³ = - r/2 ± √r²/4 - q³/27.

.

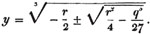(151.) When there are more equations and unknown quantities than one, a single equation, involving only one of the unknown quantities, may sometimes be obtained by the rules laid down for the solution of simple equations; and one of the unknown quantities being discovered, the others may be obtained by substituting it's value in the preceding equations.

Ex. 9.

Let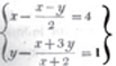 To find x and y.

[page] 85

From the first equation, 2x - x + y = 8

or x + y = 8

and x = 8 - y

from the 2^d equation, xy + 2y - x - 3y = x + 2

or xy - 2x - y = 2

by substitution,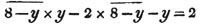

8y - y² - l6 + 2y - y = 2

9y - y² = 16 + 2 = 18

y² - 9y = - 18

y² - 9y + 81/4 = 81/4 - 18 = 9/4

y - 9/2 = ± 3/2

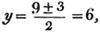, or 3

x = 8 - y = 2, or 5.

The solution will often be rendered more simple by particular artifices, the proper application of which is best learned by experience.

Ex. 10.

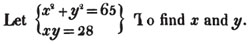To find ^x and ^y.

From the second equation, 2xy = 56

and adding this to the first, x² + 2xy + y² = 121

subtracting it from the same, x² - 2xy + y² = 9

by extracting the sq. roots, x + y = ± 11

and x - y = ± 3

therefore, 2x = ± 14

x = 7, or - 7

and y = 4, or - 4.

(152.) It may sometimes be of use to substitute for

[page] 86

one of the unknown quantities, the product of the other and a third unknown quantity*.

Ex. 11.

* This substitution may be successfully applied whenever the sum of the dimensions of the unknown quantities, in every term of each equation, is the same.

[page] 87

(153.) The operation may sometimes be shortened by substituting for the unknown quantities, the sum and difference of two others*.

Ex. 12.

Let 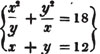To find x and y.

Assume x = z + v

and y = z - v

then x + y = 2z = 12

or z = 6

hence, x = 6 + v

and y = 6 - v

also, since x²/y + y²/x = 18

x³ + y³ = 18xy

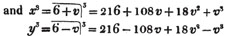
therefore, x³ + y³ = 432 + 36v²

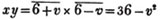
18xy = 648 - 18v²

but x³ + y³ = 18xy

therefore, 432 + 36v² = 648 - 18v²

54v² = 216

v² = 216/54 = 4

v = ± 2

x = 6 ± 2 = 8 or 4

y = 6 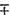2 = 4 or 8 †.

* This artifice may be used, when the unknown quantities in each equation are similarly involved.

† Other methods are given by Dr. Waring, Med. Alg. Cap. 4.

[page] 88

PROBLEMS PRODUCING QUADRATIC EQUATIONS.

PROB. 1.

(154.) A person bought a certain number of oxen for 80 guineas, and if he had bought 4 more for the same sum, they would have cost a guinea a piece less; required the number of oxen and price of each.

Let x be the number,

then 80/x is the price of each,

and 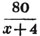the price of each on the 2^d supposition,

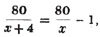by the question,

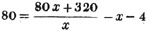
80x = 80x + 320 - x² - 4x

x² + 4x = 320

x² + 4x + 4 = 324

x + 2 = ± 18

x = ± 18 - 2 = 16 or - 20.

80/x = 80/16 = 5 guineas, the price of each.

In this, and in many other cases, especially in the solution of philosophical questions, we deduce, from the algebraical process, answers which do not correspond with the conditions. The reason seems to be, that the algebraical expression is more general than the common language, and the equation which is a proper

[page] 89

representation of the conditions, will also express other conditions, and answer other suppositions. In the foregoing instance, x may either represent a positive or a negative quantity, and cannot in the operation represent a positive quantity alone (Art. 149); and the equation 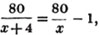when x is negative, or represents the diminution of stock, will be a proper expression for the solution of the following problem: A person sells a certain number of oxen for 80 guineas; and, had he sold 4 fewer for the same sum, he would have received a guinea a piece more for them; required the number sold.

PROB. 2.

(155.) To divide a line of 20 inches into two such parts, that the rectangle under the whole and one part, may be equal to the square of the other.

Let x be the greater part, then will 20 - x be the less, and 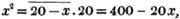by the question,

x² + 20x = 400

x² + 20x + 100 = 400 + 100 = 500

x + 10 = ± √500

x = + √500 - 10, or - √500 - 10.

The observation contained in the preceding article may be applied here; and it is to be remarked, that the negative values thus deduced are not insignificant, or useless. Here the negative value shews, that if the line be produced √500+10 inches, the square of the part produced is equal to the rectangle under the line given, and the line made up of the whole and part produced.

[page] 90

PROB. 3.

(156.) To find two numbers, whose sum, product, and the sum of whose squares, are equal to each other.

Let x + y and x - y be the numbers,

their sum is 2x

their product x² - y²

the sum of their squares 2x² + 2y²

and by the question 2x = 2x² + 2y²

or x = x² + y²

also, 2x = x² - y²

therefore, 3x = 2x²

x = 3/2

2x = x² - y²

or 3 = 9/4 - y²

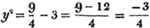
y = ± √- 3/2

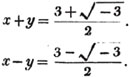
Since the square of every quantity is positive, a negative quantity has no square root; the conclusion therefore shews that there are no such numbers as the question supposes*.

* Collections of problems, producing simple and quadratic equations, may be seen in Bland's Algebra; Simpson's Exercises; and Dodson's Mathematical Repository, Vol. 1.

[page] 91

ON RATIOS.

(157.) Ratio is the relation which one quantity bears to another in respect of magnitude, the comparison being made by considering what multiple, part, or parts, one is of the other.

Thus, in comparing 6 with 3, we observe that it has a certain magnitude with respect to 3, which it contains twice; again, in comparing it with 2, we see that it has a different relative magnitude, for it contains 2 three times, or it is greater when compared with 2 than it is when compared with 3. The ratio of a to b is usually expressed by two points placed between them, thus, a : b; and the former is called the antecedent of the ratio, the latter the consequent.

(158.) COR. 1. When one antecedent is the same multiple, part, or parts, of it's consequent, that another antecedent is of it's consequent, the ratios are equal. Thus, the ratio of 4 : 6 is equal to the ratio of 2 : 3, i. e. 4 has the same magnitude when compared with 6, that 2 has when compared with 3, since 4/6 = 2/3; the ratio of a : b is equal to the ratio of c : d, if a/b = c/d, because a/b and c/d, represent the multiple, part, or parts, that a is of b, and c of d.

(159.) COR. 2. If the terms of a ratio be multiplied or divided by the same quantity, the ratio is not altered.

For a/b = ma/mb (Art. 89)

[page] 92

(160.) COR. 3. That ratio is greater than another, whose antecedent is the greater multiple, part, or parts, of it's consequent. Thus, the ratio of 7 : 4 is greater than the ratio of 8 : 5; because 7/4, or 35/20 is greater than 8/5, or 32/20. These conclusions follow immediately from our idea of ratio.

(161.) A ratio is called a ratio of greater inequality, of less inequality, or of equality, according as the antecedent is greater, less than, or equal to, the consequent.

(162.) A ratio of greater inequality is diminished, and of less inequality increased, by adding any quantity to both it's terms.

If to the terms of the ratio 7 : 4, 1 be added, it becomes the ratio of 8 : 5, which is less than the former, (Art. l60). And in general, let x be added to the terms of the ratio a : b, and it becomes a + x : b + x, which is greater or less than the former, according as 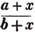is greater or less than a/b; or, by reducing them to a common denominator, as 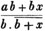is greater or less than 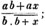that is, as b is greater or less than a.

(163.) Hence, a ratio of greater inequality is increased, and of less inequality diminished, by taking from the terms a quantity less than either of them.

(164.) If the antecedents of any ratios be multiplied together, and also the consequents, a new ratio results, which is said to be compounded of the former.

[page] 93

Thus, ac : bd is said to be compounded of the two a : b and c : d. It is also sometimes called the sum of the ratios; and when the ratio a : b is compounded with itself, the resulting ratio, a² : b², is called the double of the ratio of a : b, and if three of these ratios be compounded together, the result a³ : b³, is called the triple of the first, &c. Also, the ratio of a : b is said to be one third of the ratio of a³ : b³; and a^1/m : b^1/m is said to be an m^th part of the ratio of a : b.

(165.) Let the first ratio be a : 1; then a² : 1, a³ : 1, …. aⁿ : 1, are twice, three times, … n times the first ratio; where n, the index of a, shews what multiple, or part, of the ratio aⁿ : 1, the first ratio a : 1, is. On this account, the indices 1, 2, 3, … n, are called measures of the ratios a¹ : 1, a² : 1, a³ : 1, …. aⁿ : 1.

(166.) If the consequent of the preceding ratio be the antecedent of the succeeding one, and any number of such ratios be taken, the ratio which arises from their composition, is that of the first antecedent to the last consequent.

Let a : b, b : c, c : d, &c. be the ratios, the compound ratio is a x b x c : b x c x d, (Art. 164.); or, dividing by b x c (Art. 159), a : d.

(167.) A ratio of greater inequality, compounded with another, increases it; and a ratio of less inequality diminishes it.

Let the ratio of x : y be compounded with the ratio of a : b, and the resulting ratio ax : by is greater or less than the ratio a : b, according as ax/by is greater or

[page] 94

less than a/b (Art. 160); i. e. according as x is greater or less than y.

(168.) If the difference between the antecedent and consequent of a ratio be small when compared with either of them, the double of the ratio, or the ratio of their squares, is nearly obtained by doubling this difference.

Let a + x : a be the proposed ratio, where x is small when compared with a; then a² + 2ax + x²: a² is the ratio of the squares of the antecedent and consequent; but since x is small when compared with a, x² or x x x is small when compared with 2a x x, and much smaller than a x a; therefore, a² + 2ax : a², or a + 2x : a (Art. 159), nearly express the ratio of a² + 2ax + x² : a².

Thus, the ratio of the square of 1001 to the square of 1000 is nearly 1002 : 1000; the real ratio is 1002.001 : 1000, in which the antecedent differs from its approximate value, only by one thousandth part of an unit.

(169.) COR. Hence, the ratio of the square root of a + 2x to the square root of a is the ratio a + x : a, nearly; that is, if the difference of two quantities be small with respect to either of them, the ratio of their square roots is nearly obtained by halving their difference.

(170.) In the same manner, a + 3x : a; a + 4x : a; a + mx : a; are nearly equal to the ratios 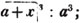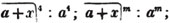if mx be small when compared with a.

[page] 95

ON PROPORTION.

(171.) Four quantities are said to be proportionals, when the first is the same multiple, part, or parts, of the second, that the third is of the fourth. That is, when a/b = c/d, the four quantities a, b, c, d, are called proportionals. This is usually expressed by saying, a is to b as c to d; and thus represented, a : b :: c : d.

The terms a and d are called the extremes, and b and c the means.

(172.) When four quantities are proportionals, the product of the extremes is equal to the product of the means.

Let a, b, c, d, be the four quantities; then, since they are proportionals, a/b = c/d (Art. 171); and by multiplying both sides by bd, ad = bc.

(173.) COR. 1. If the first be to the second as the second to the third, the product of the extremes is equal to the square of the mean.

(174.) COR. 2. Any three terms in a proportion being given, the fourth may be determined from the equation ad = bc.

(175.) If the product of two quantities be equal to the product of two others, the four are proportionals, making the terms of one product the means, and the terms of the other, the extremes.

Let xy = ab, then dividing by ay, x/a = b/y, or x : a :: b : y. (Art. 171).

[page] 96

(176.) If a : b :: c : d, and c : d :: e : f, then will a : b :: e : f.

Because a/b = c/d, and c/d = e/f; therefore, a/b = e/f; or a : b :: e : f.

(177.) If four quantities be proportionals, they are also proportionals when taken inversely.

If a : b :: c : d, then b : a :: d : c. For a/b = c/d, and dividing unity by each of these equal quantities, or taking their reciprocals, b/a = d/c; that is, b : a :: d : c.

(178.) If four quantities be proportionals, they are proportionals when taken alternately.

If a : b :: c : d, then a : c :: b : d.

Because the quantities are proportionals, a/b = c/d and multiplying by b/c, a/c = b/d, or a : c :: b : d.

Unless the four quantities are of the same kind, the alternation cannot take place, because this operation supposes the first to be some multiple, part, or parts, of the third.

One line may have to another line the same ratio that one weight has to another weight, but a line has no relation, in respect of magnitude to a weight. In cases of this kind, if the four quantities be represented by numbers, or other quantities which are similar, the alternation may take place, and the conclusions drawn from it will be just.

[page] 97

(179.) When four quantities are proportionals, the first together with the second, is to the second, as the third together with the fourth, is to the fourth.

Let a : b :: c : d, then

componendo, a + b : b :: c + d : d.

Because a/b = c/d, by adding unity to each side, a/b + 1 = c/d + 1; that is, 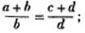; or, a + b : b :: c + d : d.

(180.) Also, dividendo, the excess of the first above the second, is to the second, as the excess of the third above the fourth, is to the fourth.

Because a/b = c/d, by subtracting unity from each side, a/b - 1 = c/d - 1; that is, 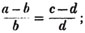; or, a - b : b :: c - d : d.

(181.) Again, convertendo, thefirst is to it's excess above the second, as the third to it's excess above the fourth.

By the last article, 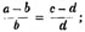and b/a=d/c (Art.177); therefore, 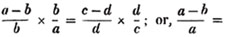,,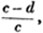 that is, a - b : a :: c - d : c; and inversely, a : a - b :: c : c - d.

(182.) When four quantities are proportionals, the sum of the first and second is to their difference, as the sum of the third and fourth, to their difference.

Let a : b :: c : d; then, a + b : a - b :: c + d: c - d.

G

[page] 98

By Art. 179, 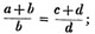; amd by Art. 180, 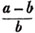; ;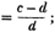therefore, 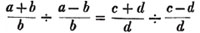;(Art.70); 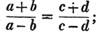;that is, a + b : a - b :: c + d : c - d.

(183.) When any number of quantities are proportionals, as one antecedent is to it's consequent, so is the sum of all the antecedents, to the sum of all the consequents.

Let a : b :: c : d :: e : f, &c.
then a : b :: a + c + e : b + d + f.
Because a/b = c/d, ad = bc; in the same manner, af = be;
also, ab = ba; hence, ab + ad + af = ba + bc + be, or, 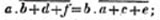; and by Art. 175, a : b :: a + c + e : b + d + f.

(184.) When four quantities are proportionals, if the first and second be multiplied, or divided, by any quantity, as also the third and fourth, the resulting quantities will be proportionals.

Let a : b :: c : d, then will ma : mb :: c/n : d/n.

For a/b = c/d; therefore, 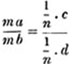(Art. 89);
or, ma : mb :: c/n : d/n.

(185.) If the first and third be multiplied, or divided, by any quantity, and also the second and fourth, the resulting quantities will be proportionals.

[page] 99

For a/b = c/d; therefore ma/b = mc/d; and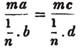
(Art. 69) or, ma : b/n :: mc : d/n.

(186.) COR. Hence, in any proportion, if instead of the second and fourth terms, quantities proportional to them be substituted, we have still a proportion. For b/n and d/n are in the same proportion with b and d (Art. 184.)

(187.) In two ranks of proportionals, if the corresponding terms be multiplied together, the products will be proportionals.

Let a : b :: c : d
and e : f :: g : h
then will ae : bf :: eg : dh.

Because a/b = c/d and e/f = g/h; therefore, ae/bf = eg/dh; or, ae : bf :: eg : dh.

This is called compounding the proportions.

The proposition is true if applied to any number of proportions.

(188.) If four quantities be proportionals, the like powers, or roots of these quantities, will be proportionals.

Let a : b :: c : d, then a/b = c/d, and aⁿ/bⁿ = cⁿ/dⁿ; or, aⁿ : bⁿ :: cⁿ : dⁿ; where n is whole or fractional.

(189.) If two numbers, a and b, be prime to each other, they are the least in that proportion.

G 2

[page] 100

If possible, let a/b = c/d, where a and b are prime to each other, and respectively greater than c and d. If the latter numbers be not prime to each other, divide them by their greatest common measure. Then divide a by b, and c by d, as in Art. go; thus,

and because a/b = c/d the first quotients m, m, are equal; again, since a/b = m + x/b, and c/d = m + r/d, we have x/b = r/d, or b/x = d/r; also, because b is greater than d, x is greater than r. In the same manner, x/y = r/s, and y is greater than s, &c. thus the remainder in the latter division will become unity, sooner than the remainder in the former. Let s = 1; then x/y = r, and y, which is greater than unity, will measure a and b (Art. 92), which is contrary to the supposition.

COR. Hence, if /ab = c/d, and a and b be prime to each other, c and d are equimultiples of a and b.

(190.) If a and b be each of them prime to c, ab is prime to c.

If not, let ab = mr, and c = ms; then since a and b are prime to c, they are respectively prime to ms, and therefore to m; and because ab = mr, we have a/m = r/b;

[page] 101

therefore b is a multiple of m (Art. I89, Cor.), which is absurd.

COR. 1. If b be equal to a, then a², and c have no common measure: or a²/c is a fraction in it's lowest terms.

COR. 2. In the same manner, a³/c, a⁴/c, &c. are fractions in their lowest terms.

COR. 3. If a, b, and c, be each of them prime to d, e, and f, abc is prime to def.

For, if a be prime to d and e, it is prime to de, and if it be prime to de and f, it is prime to def. In the same manner, b and c are prime to def, consequently, abc is prime to def.

COR. 4. If a be prime to b, a² is prime to b², and a³ to b³, &c.

SCHOLIUM.

(191.) In the definition of Proportion, it is supposed that one quantity is some determinate multiple, part, or parts of another; or that the fraction arising from the division of one by the other, (which expresses the multiple, part, or parts, that the former is of the latter), is a determinate fraction. This will be the case, whenever the two quantities have any common measure whatever.

Let x be a common measure of a and b, and let a = mx, b = nx; then a/b = mx/nx = m/n, where m and n are whole numbers.

But it sometimes happens that the quantities are

[page] 102

incommensurable, or adroit of no common measure whatever, as when one represents the circumference of a circle and the other it's diameter; in such cases, the value of a/b cannot be exactly expressed by any fraction, m/n, whose numerator and denominator are whole numbers; yet a fraction of this kind may be found, which will express it's value to any required degree of accuracy.

Suppose x to be a measure of b, and let b=nx; also let a be greater than mx but less than 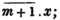; then a/b is greater than m/n but less than 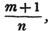, or the difference between m/n and a/b is less than 1/n; and as x is diminished, since nx=b, n is increased, and 1/n diminished; therefore by diminishing x, the difference between m/n and a/b may be made less than any that can be assigned.

If a and b as well as c and d be incommensurable, and if when a/b lies between m/n and 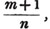, c/d lie also between and m/n and 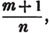, however the magnitudes m and n are increased, a/b is equal to c/d. If they are not equal, they musthave some assignable difference, and because each of them lies between m/n and 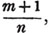, this difference

[page] 103

is less than 1/n; but since n may, by the supposition, be increased without limit, 1/n may be diminished without limit, that is, it may become less than any assignable magnitude; therefore, a/b and c/d have no assignable difference; that is, a/b is equal to c/d; and all the preceding propositions, respecting proportionals, are true of the four magnitudes, a, b, c, d.

ON VARIABLE QUANTITIES.

(192.) In the investigation of the relation which varying and dependent quantities bear to each other, the conclusions are more readily obtained, by expressing only two terms in each proportion, than by retaining the four.

But though, in considering the variation of such quantities, two terms only are expressed, it will be necessary for the Learner to keep constantly in mind that four are supposed; and that the operations, by which our conclusions are in this case obtained, are in reality the operations of proportionals.

(193) DEF. 1. One quantity is said to vary directly as another, when the two quantities depend wholly upon each other, and in such a manner, that if one be changed, the other is changed in the same proportion.

Let A and B be mutually dependent upon each other, in such a way, that if A be changed to any other value a, B must be changed to auother value b,

[page] 104

such, that A : a :: B : b, then A is said to vary directly as B.

Ex. If the altitude of a triangle be invariable, the area varies as the base. For if the base be increased, or diminished, the area is increased or diminished in the same proportion*.

(194.) DEF. 2. One quantity is said to vary inversely as another, when the former cannot be changed in any manner, but the reciprocal of the latter is changed in the same proportion.

A varies inversely as B, (A∝1/B if, when A is changed to a, B be changed to b, in such a manner that A : a :: 1/B : 1/b; or A : a :: b : B.

Ex. If the area of a triangle be given, the base varies inversely as the perpendicular altitude.

Let A and a represent the altitudes, B and b, the bases, of two equal triangles; then 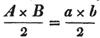(the area of a triangle being the half of the rectangle under the base and perpendicular), or A x B = a x b; therefore (Art. 175). A : a :: b : B :: 1/B : 1/b.

(195.) DEF. 3. One quantity is said to vary as two others jointly, if, when the former is changed in any manner, the product of the other two be changed in the same proportion.

Thus, A varies as B and C jointly, (A∝BC), when A cannot be changed to a, but the product BC must be changed to bc, such, that A : a :: BC : bc.

* The sign ∝ placed between two quantities signifies that they vary as eaxh other.

[page] 105

Ex. The area of a triangle varies as it's base and perpendicular altitude jointly. Let A, B, P, represent the area, base, and perpendicular altitude of'one triangle; a, b, p, those of another; then BP = 2A, and bp = 2a; therefore A/a = BP/bp, or A : a :: BP : bp.

(196.) DEF. 4. One quantity is said to vary directly as a second and inversely as a third, when the first cannot be changed in any manner, but the second multiplied by the reciprocal of the third, is changed in the same proportion.

A varies directly as B, and inversely as C, (A∝B/C), when A : a : B/C : b/c; A, B, C, and a, b, c, being corresponding values of the three quantities.

Ex. The base of a triangle varies as the area directly and the perpendicular altitude inversely. The notation in the last Article being retained, BP/bp = A/a; and multiplying both sides by p/P we have B/b = Ap/aP = A/P ÷ a/p; therefore, B : b :: A/P : a/p.

In the following articles, A, B, C, &c. represent corresponding values of any quantities, and a, b, c, &c. any other corresponding values of the same quantities.

(197.) If one quantity vary as a second, and that second as a third, the first varies as the third.

Let A : a :: B : b, and B : b :: C : c, then, (Art. 176), A : a :: C : c. That is, A∝C. In the same manner, if A∝B and B∝1/C, then Aα1/C.

[page] 106

(198.) If two quantities vary respectively as a third, their sum, or difference, or the square root of their product, will vary as the third.

Let A∝C and B∝C, then 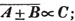; also, √AB∝C. By the supposition, A : a :: C: c:: B: b; therefore A : a :: B : b; alternately, A : B :: a : b; and by composition or division, A ± B : B :: a ± b : b; alt. A ± B : a ± b :: B : b :: C: c; that is, .

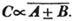Again, A : a :: C: c
and B : b :: C : c.
therefore, AB : ab C² : c² (Art. 187)
and √AB : √ab :: C : c (Art. 188); that is, Cα√AB.

(199.) If one quantity vary as another, it will also vary as any multiple, or part, of the other.

Let A∝B, and m be any constant quantity, then because A: a:: B : b, A : a :: mB: mb, or A : a :: B/m: b/m (Art. 184); that is, A∝mB or ∝ B/m.

(200.) COR. 1. If A vary as B, A is equal to B multiplied by some invariable quantity. For A : a :: mB : mb, altern. A : mB :: a : mb; if therefore m be so assumed that A = mB, then in all cases, a = mb.

(201.) COR. 2. If we know any corresponding values of A and B, the constant quantity m may be found.

Let a and b be the two values known, then m = a/b; and in general, A = a/b x B.

[page] 107

Ex. Let S ∝ T², and when T = 1 suppose S = 16, then S = 16 T².

(202.) If one quantity vary as another, any power or root of the former will vary as the same power or root of the latter.

Let A vary as B, then A : a :: B : b, and by Art. 188, Aⁿ : aⁿ :: Bⁿ : b^n; that is, Aⁿ∝Bⁿ, where n is whole or fractional.

(203.) If one quantity vary as another, and each of them be multiplied or divided by any quantity, variable or invariable, the products or quotients will vary as each other.

Let A vary as B, and let T be any other quantity. Then, by the supposition, A : a :: B : b; therefore AT : at :: BT : bt, and A/T :: a/t :: B/T : b/t (Art. 185).

(204.) COR. If A∝B, dividing both by B, A/B ∝ B/B ∝ that is, A/B is constant.

(205.) If one quantity vary as two others jointly, either of the latter varies as the first directly and the other inversely.

Let V∝FT, then by Art. 203,
F∝V/T or T∝V/F.

(206.) COR. If the product of two quantities be invariable, those quantities vary inversely as each other.

Let B x P be constant, or B x P∝1; by division, B∝1/P.

[page] 108

(207.) If four quantities be always proportionals, and one or two of them be invariable, we may find how the others vary.

Ex. Let p, q, r, s, be always proportionals, and let p be invariable, then s∝qr. Because ps = qr (Art. 172), psαqr; and since p is constant, s∝qr, (Art. 199).

(208.) If one quantity vary as a second, and a third as a fourth, the product of the first and third will vary as the product of the second and fourth.

Let A∝B and C∝D, then AC∝BD.

Because A : a :: B : b
and C : c :: D : d
AC : ac :: BD : bd (Art. 187)
that is, AC∝BD.

(209.) When the increase or decrease of one quantity depends upon the increase or decrease of two others, and it appears that if either of these latter be invariable, the first varies as the other; when they both vary, the first is as their product.

Let S∝V when T is given,
and S∝T when V is given;

when neither T nor V is given, S∝TV. The variation of S depends upon the variations of the two quantities T and V; let the changes take place separately, and whilst T is changed to t, let S be changed to S¹; then by the supposition, S : S¹ :: T : t; but this value S¹ will again be changed to s, by the variation of V, and in the same proportion that V is changed; that is, S¹ : s :: V: v, and by compounding this with the last proportion, S¹S : S¹s :: TV : tv; or, S : s :: TV: tv (Art. 184).

[page] 109

(210.) In the same manner, if there be any number of magnitudes P, Q, R, S, each of which vanes as another, V, when the rest are constant; when they are all changed, V varies as their product.

ON ARITHMETICAL PROGRESSION.

(211.) Quantities are said to be in arithmetical progression, when they increase or decrease by a common difference.

Thus, 1, 3, 5, 7, 9, &c. a, a +1, a + 2b, a + 3b, &c. a, a - b, a - 2b, a - 3b, &c. are in arithmetical progression.

Hence it is manifest, that if a be the first term and a + b the second, a + 2b is the third, a + 3b the fourth, &c. and 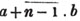.b the n^th term.

(212.) The sum of a series of quantities in arithmetical progression is found by multiplying the sum of the Jirst and last terms by half the number of terms.

Let a be the first term, b the common difference, n the number of terms, and s the sum of the series: Then,

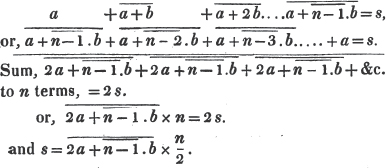
[page] 110

(213.) COR. Any three of the quantities s, a, n, b, being given, the fourth may be found from the equation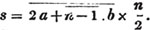

Ex. 1.

To find the sum of 14 terms of the series 1, 3, 5, 7, &c.

Here a = 1, 6 = 2, n = 14; therefore, 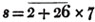= 196.

Ex. 2.

Required the sum of 9 terms of the series 11, 9, 7, 5, &c.

In this case a = 11, b = -2, n = 9; therefore, s =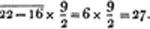

Ex. 3.

If the first term of an arithmetical progression be 14, and the sum of 8 terms be 28, what is the common difference?

Since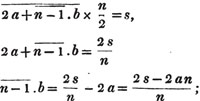

therefore, 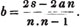In the case proposed, s = 28, a = 14, n = 8 therefore, 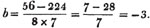. Hence, the series is 14, 11, 8, 5, &c.

[page] 111

ON GEOMETRICAL PROGRESSION.

(214.) Quantities are said to be in geometrical progression, or continual proportion, when the first is to the second, as the second to the third, and as the third to the fourth, &c. that is, when every succeeding term is a certain multiple, or part of the preceding term.

If a be the first term, and ar the second, the series will be a, ar, ar², ar³, ar³, &c.

For a : ar :: ar : ar² :: ar² : ar³, &c.

(215.) The constant multiplier is called the common ratio, and it may be found by dividing the second term by the first, or any other term by that which precedes it.

(216.) If quantities be in geometrical progression, their differences are in geometrical progression.

Let a, ar,ar², ar³, ar⁴, &c. be the quantities; their differences, ar - a, ar² - ar, ar⁴ - ar³, &c. form a geometrical progression, whose first term is ar - a, and common ratio r.

(217.) Quantities in geometrical progression are proportional to their differences.

For a : ar :: ar - a : ar² - ar :: ar² - ar : ar³ - ar², &c.

(218.) In any geometrical progression, the first term is to the third, as the square of the first to the square of the second.

Let a, ar, ar² &c. be the progression; then a : ar² :: a² : a²r².

[page] 112

Hence it appears, that the duplicate ratio of two quantities (Euc. Def. 10. 5), is the ratio of their squares.

(219.) In the same manner it may be shewn, that the first term is to the term, as the first raised to the n^th power, to the second raised to the same power.

(220.) If any terms be taken at equal intervals in a geometrical progression, they will be in geometrical progression.

Let a, ar … arⁿ ….. ar²ⁿ ….. ar³ⁿ ….. &c. be the progression, then a, ar, arⁿ, ar²ⁿ, ar³ⁿ &c. are at the interval of n terms, and form a geometrical progression, whose common ratio is rⁿ.

(221.) If the two extremes, and the number of terms in a geometrical progression be given, the means may be found.

Let a and b be the extremes, n the number of terms, and r the common ratio; then the progression is a, ar, ar², ar³ ….. ar^n-1;; and since b is the last term, ar^n-l = b, and and r being thus known, the terms of the progression ar, ar³, ar³, &c. are known.

(222.) To find the sum of a series of quantities in geometrical progression, subtract thefirst term from the product of the last term and common ratio, and divide the remainder by the difference between the common ratio and unity.

Let a be the first term, r the common ratio, n the

[page] 113

number of terms, y the last term, and s the sum of the series:

Then a + ar + ar² …. + ar^n-2 + ar^n-1 = s; and multiplying both sides by r,

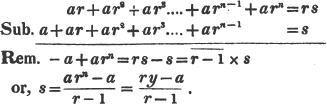
(223) COR. 1. Prom the equation 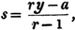, any three of the quantities, s, r, y, a, being given, the fourth may be found.

(224.) COR. 2. When r is a proper fraction, as n increases, the value of rⁿ, or of arⁿ, decreases, and when n is increased without limit, arⁿ becomes less, with respect to a, than any magnitude that can be assigned; and therefore .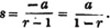

This quantity 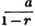which we call the sum of the series, is the limit to which the sum of the terms approaches, but never actually attains; it is however the true representative of the series continued sinefine; for this series arises from the division of a by 1 - r; and therefore 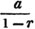may, without error, be substituted for it.

Ex. 1.

To find the sum of 20 terms of the series, 1, 2, 4, 8, &c.

H

[page] 114

Here a = 1, r = 2, n = 20; therefore,

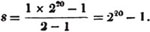
Ex. 2.

Required the sum of 12 terms of the series 64, l6, 4, &c.

Here a = 64, r 1/4, n = 12; therefore,

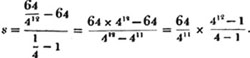
Ex. 3.

Required the sum of 12 terms of the series 1, -3, 9, -27, &c.

In this case, a = 1, r = -3, n = 12;

therefore,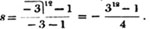

Ex. 4.

To find the sum of the series 1 - 1/2 + 1/4 - 1/8 &c. in infinitum.

Here a = 1, r = 1/2; therefore (Art. 224),

.

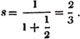(225.) Recurring decimals are quantities in geometrical progression, where 1/10, 1/100, 1/1000, &c. is the common ratio, according as one, two, three, &c.

[page] 115

figures recur; and the vulgar fraction, corresponding to such a decimal, is found by summing the series.

Ex. 5.

Required the vulgar fraction corresponding to the decimal.123123123 &c.

Let.123123123 &c. = s; then, as in Art. 222, multiply both sides by 1000; and 123.123123123 &c. = 1000s, and by subtracting the former equation from the latter, 123 = 999s; therefore s = 123/999 = 41/333.

ON PERMUTATIONS AND COMBINATIONS.

(226.) The different orders in which any quantities can be arranged, are called their permutations.

Thus, the permutations of a, b, c, taken two and two together, are ab, ba, ac, ca, bc, cb.

(227.) The combinations of quantities are the different collections that can be formed out of them, without regarding the order in which the quantities are placed.

Thus, ab, ac, bc, are the combinations of the quantities a, b, c, taken two and two; ab and ba, though different permutations, forming the same combination.

(228.) The number of permutations that can be formed out of n quantities, taken two and two together, is 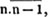, taken three and three together, is .

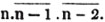In n things, a, b, c, d, &c. a may be placed before each of the rest, and thus form permutations; in

H 2

[page] 116

the same manner, there are 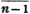permutations in which b stands first, and so of the rest; therefore there are, upon the whole, 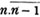permutations of this kind ab, ba, ac, ca, &c.

Again, of n - 1 things b, c, d, &c. taken two and two together, there are 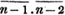permutations, by the former part of the article, and by prefixing a to each of these, there are 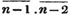permutations, taken three and three, in which a stands first; the same may be said of b, c, d, &c. therefore there are, upon the whole, 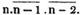such permutations.

(229.) COR. By following the same method, it appears, that in n things, if r of them be always taken together, there are 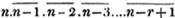permutations.

(230.) The number of combinations that can be formed out of n things, taken two and two together, is 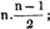taken three and three together, the number in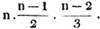

The number of permutations in the first case is 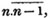but each combination ab, admits of two permutations, ab, ba; therefore there are twice as many permutations as combinations, or the number of combinations is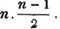

Again, there are 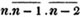permutations in n things, taken three and three together; and each combination of three things admits of 3.2.1 permutations (Art. 228); therefore there are 3.2.1 times as

[page] 117

many permutations as combinations, and consequently the number of combinations is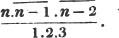

(231.) COR. In the same manner it appears, that the number of combinations, in n things, each of which contains r of them, is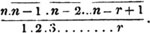

ON THE BINOMIAL THEOREM.

(232.) The method of raising a binomial to any power, by repeated multiplication, has before been laid down (Art. 117). The same thing may be done much more expeditiously by the following general rule, which is called the Binomial Theorem.

Let x + a be the given binomial; and it's n^th power is 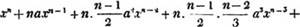&c. Where the index of x, beginning from n is diminished by unity, and the index of a, beginning from 0, is increased by unity, in every succeeding term. Also, the coefficient of each term is found by multiplying the coefficient of the preceding term by the index of x in that term, and dividing by the index of a increased by unity.

.

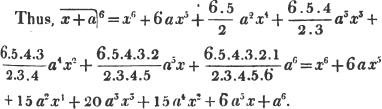[page] 118

To investigate this theorem, suppose n quantities, x + a, x + b, x + c, &c. multiplied together; it is manifest that the first term of the product will be xⁿ, and that, x^n-1, x^n-2 &c. the other powers of x, will all be found in the remaining terms, with different combinations of a, b, c, d, &c.

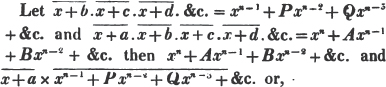
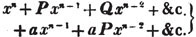are the same series;

therefore A = P + a, b = Q + aP, &c. that is, by introducing one factor, x = a, into the product, the coefficient of the second term is increased by a, and by introducing x = b into the product, that coefficient is increased by b, &c. therefore the whole value of A is a + b + c + d + &c. Again, by the introduction of one factor, x + a, the coefficient of the third term, Q, is increased by aP, i. e. by a multiplied by the preceding value of A, or by and the same may be said with respect to the introduction of every other factor; therefore upon the whole,

.

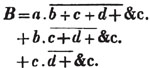In the same manner,

.

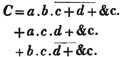and so on; that is, A is the sum of the quantities a,

[page] 119

b, c, &c. B is the sum of the products of every two; C is the sum of the products of every three, &c. &c.

Let a = b = c = d= &c. then A, or a + b + c + d + &c. = nz; B = ab + ac + bc + &c. = a² x the number of combinations fo a, b, c, d, &c. taken two and two, 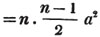. (Art. 230); in the same manner it appears, that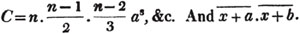

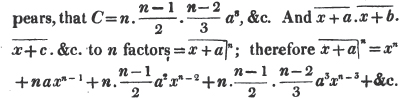
This proof applies only to those cases in which n is a whole positive number; but the rule holds when the index is fractional, or negative.

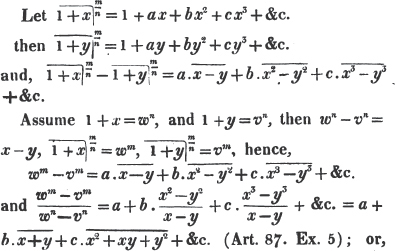dividing the numerator and denominator of the fraction 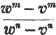by their common measure w-v, we have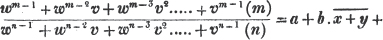

[page] 120

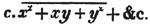. Now let x = y, then w = v, and the equation becomes .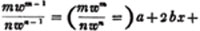

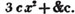And multiplying by wⁿ = 1 + x,

.

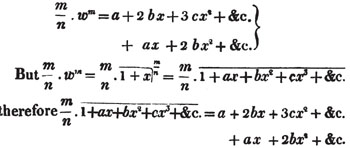And since these must form the same series, m/n = a; the same manner, the proposition may be proved when

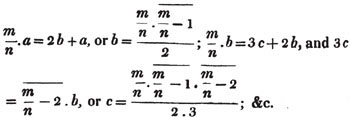Nearly in the same manner, the proposition may be proved when m/n is negative.*

* See Encyclop±dia Britan. vol. I. page 651.
It may not be improper to state, briefly, the nature of the proof alluded to in former Editions of this work, as the principle is of extensive application.
It is usually taken for granted, and may without much difficulty be proved, that whether r is positive or negative, whole or fractional, 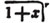may properly be expressed by 1 + ax + bx² + cx³ + &c. where a, b, c, &c. are definite magnitudes, not dependent on the value of x. Let 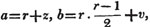, then, as appears from what has been proved before (p.118), when r is any whole positive number a = r, and z=0 when r is 1, 2, 3, &c. or z contains the factors r - 1, r - 2, r - 3, &c. in inf. (Art. 269); hence, . in inf., which cannot be expressed in finite terms; consequentley r + z cannot be expressed in finite terms unless Q, that is, unless z = 0; and since we know that a, or r + z may be expressed in infinite terms, it fallows that z = 0, and that a = r. In the same manner it appears that .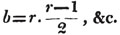

[page] 121

Ex. 1.

.

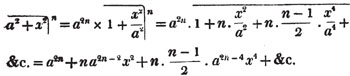Ex. 2.

.

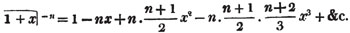Ex. 3.

.

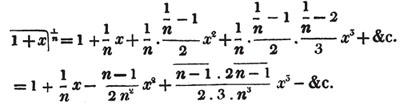Ex. 4.

.

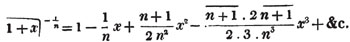(233.) COR. 1. If either term of the binomial be negative, it's odd powers will be negative (Art. 114);

[page] 122

and consequently the signs of the terms, in which those odd powers are found, will be changed.

Ex. 5.

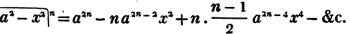
(234.) COR. 2. If the index of the power, to which a binomial is to be raised, be a whole positive number, the series will terminate, because the coefficient 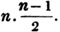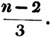will become nothing, when it is continued to 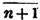factors. In all other cases the number of terms will be indefinite.

(235.) COR. 3. When the index is a whole positive number, the coefficients of the terms taken backward, from the end of the series, are respectively equal to the coefficients of the corresponding terms taken forward, from the beginning.

Thus, if a + x be raised to the 8^th power, the coefficients are 1, 8, 28, 56, 70, 56, 28, 8, 1.

In general, the coefficient of the 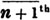term is 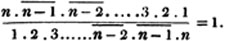The coefficient of the nth term is 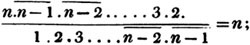= n; of the term,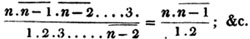

(236.) COR. 4. The sum of the coefficients 1 + n + 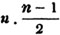&c. is 2 ⁿ.

[page] 123

For if x=a=1, then 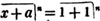= 2ⁿ = 1 + n + 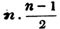+ &c.

(237.) COR. 5. Since

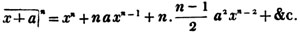and 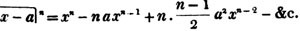by addition, 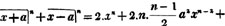&c. or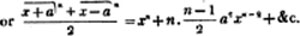

By subtractingone series from the other,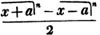

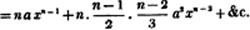
(238.) The trinomial a + b + c may be raised to any power by considering two terms as one factor, and proceeding as before.

Thus,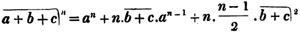

a^{n - 2} + &c. and the powers of b + c may be determined by the binomial theorem.

The theorem by which a multinomial may be raised to any power, is given by Mr. Demoivre, Analyt. page 87.

ON SURDS.

(239.) A quantity may be reduced to the form of a given surd, by raising it to the power whose root the sUrd expresses, and affixing the radical sign.

[page] 124

Thus, 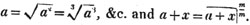. In the same manner, the form of any surd may be altered; thus, 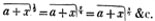. The quantities are here raised to certain powers, and the roots of those powers are again taken, therefore the values of the quantities are not altered.

(240.) If two surds have the same index, their product is found by taking the product of the quantities under the signs, and retaining the common index.

Thus, 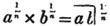(Art. 124); √ 2 x √ 3 = √ 6;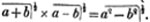

(241.) If the surds have coefficients, the product of these coefficients must be prefixed.

Thus, a√x×b √y = ab √xy

(242.) We must observe, that √-a², x √-a², or the square of √-a², is a² because it is that quantity whose square root is √-a².

COR. Hence, 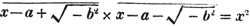- 2ax + a² + b².

(243.) If the indices of two surds have a common denominator, let the quantities be raised to the powers expressed by their respective numerators, and their product may be found as before.

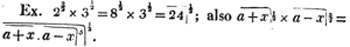
(244.) If the indices have not a common denominator, they may be transformed to others of the same

[page] 125

value, with a common denominator, and their product found as in Art. 243.

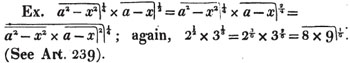
(245.) If two surds have the same rational quantity under the radical signs, their product isfouiid by making the sum of the indices the index of that quantity.

Thus, a^1/n x a^1/m = a^m/mn x a^n/mn (Art. 239), = a^m+n/mn; (See Art. 124.)

Ex. 2.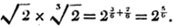

(246.) If the indices of two quantities have a common denominator, the quotient of one divided by the other is obtained by raising them, respectively, to the powers expressed by the numerators of their indices, and extracting that root of the quotient which is expressed by the common denominator.

For,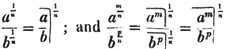

Ex.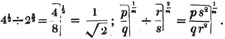

(247.) If the indices have not a common denominator, reduce them to others of the same value, with a common denominator, and proceed as before.

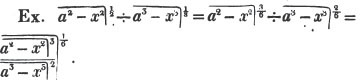
[page] 126

(248.) If two surds have the same rational quantity under the radical signs, their quotient is obtained by making the difference of the indices, the index of that quantity.

Thus, a^1/n divided by a^1/m, or a^m/mn divided by a^n/mn (Art. 239), that is 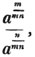is equal to 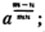because these quantities, raised to thepower mn, produce equal results a^m/aⁿ and a^m-n.

Ex. 2. 2^1/2 ÷ 2^1/3 = 2 ^3/6 ÷ 2^2/6 = 2^1/6.

(249.) The coefficient of a surd may be introduced under the radical sign, by first reducing it to the form of the surd, and then multiplying as in Art. 240.

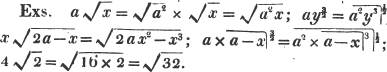
(250.) Conversely, any quantity may be made the coefficient of a surd, if every part under the sign be divided by this quantity raised to the power whose root the sign expresses.

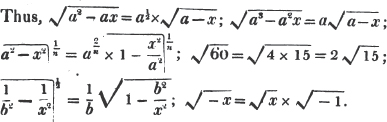
[page] 127

(251.)When surds have the same irrational part, their sum or difference is found by affixing the sum or difference of their coefficients to that irrational part.

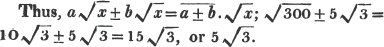
(252.) The square root of a quantity cannot be partly rational and partly a quadratic surd.

If possible, let = √n = a + √m; then by squaring both sides, n = a² + 2a√m + m, and 2a√m = n - a² - m; therefore, 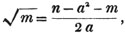a rational quantity, which is contrary to the supposition.

(253.) In any equation x + √y = a + √b, consisting of rational quantities and quadratic surds, the rational parts on each side are equal, and also the irrational parts.

If x be not equal to a, let x = a + m; then a + m + √y = a +√b, or m + √y = √b; that is, √b is partly rational and partly a quadratic surd, which is impossible (Art. 252); therefore x = a, and consequently √y = √b.

(254.) If two quadratic surds √x and √y cannot be reduced to others which have the same irrational part, their product is irrational.

If possible, let √xy = rx, where r is a whole number or a fraction. Then xy = r²x², and y = r²x, therefore √y = r √x, that is, √y and √x may be so reduced as to have the same irrational part, which is contrary to the supposition.

[page] 128

(255.) One quadratic surd, √x, cannot be made up of two others, √m and √n, which have not the same irrational part.

If possible, let √x = √m + √n; then by squaring both sides, x = m + n + 2√mn, and x - m - n = 2√mn, a rational quantity equal to an irrational one (Art. 254); which is absurd.

(256.) Let 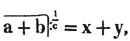where c is an even number, a a rational quantity, b a quadratic surd, x and y one or both of them, quadratic surds, then 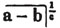= x - y.

By involution, 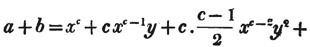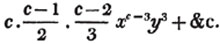and since c is even, the odd terms of the series are rational, and the even terms irrational; therefore 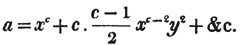and 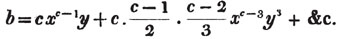(Art. 253); hence, 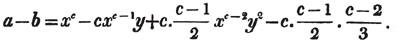x^{c - 3}y³ + &c. and consequently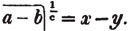

(257.) If c be an odd number,a and b, one or both quadratic surds, and x and y involve the same surds that a and b do, respectively, and also 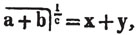then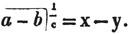

[page] 129

By involution, 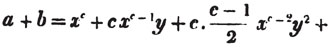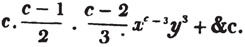where the odd terms involve the same surd that x does, because c is an odd number, and the even terms, the same surd that y does; and since no part of a can consist of y, or it's parts (Art. 255), 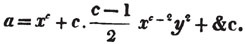and b = 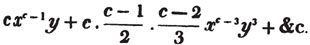hence, a - b = x^c - 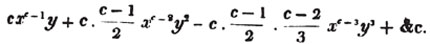therefore, by evolution,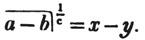

(258.)The square root of a binomial, one of whose factors is a quadratic surd, and the other rational, may sometimes be expressed by a binomial, one or both of whose factors are quadratic surds.

Let a + √b the given binomial, and suppose 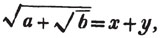where x and y are one or both quadratic surds;

then 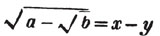(Art. 256),

by multiplication 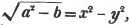also, by squaring both sides of the first equation,

a + √b = x² + 2xy +y²

and a = x² + y² (Art. 253),

by addition,

I

[page] 130

by subtraction, a - √² - b = 2y², and the root x + y =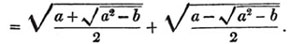

From this conclusion it appears, that the square root of a + √b can only be expressed by a binomial of the form x + y, one or both of which are quadratic surds, when a² - b is a perfect square.

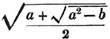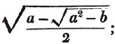The values of x and y are ± and ± there are therefore four different values of x + y; two of which were introduced in the operation (See Art. 149), and will not answer the conditions of the question.

Ex.1.

Required the square root of 3 + 2 √2.

In this case, a = 3, √b = 2 √2, and a² - b = 9 - 8 = 1; hence,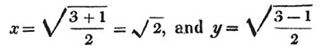

= 1; therefore, x + y = √2 + 1.

Ex. 2.

Required the square root of 7 - 2 √10.

Here, a = 7, √b = 2 √10, a² - b = 9; hence, 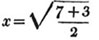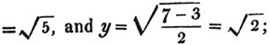therefore, x - y = √5 - √2, the root required.

[page] 131

Ex. 3.

Required the square root of 4√- 5 - 1.

Here, a = - 1, √ b = 4 √ - 5, a² - b = 81; hence, x = 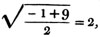and y = √- 10/2 = √ - 5; and the root required is 2 + √- 5.

(259.) The c^th root of a binomial, one or both of whose factors are possible quadratic surds, may sometimes be expressed by a binomial of that description.

Let A + B be the given binomial surd, in which both terms are possible; the quantities under the radical signs whole numbers; and A is greater than B.

Let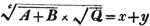

then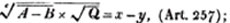

by mult.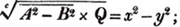 let Q be so assumed that 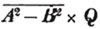may be a perfect c^th power = n^c, then x² - y² = n.

Again, by squaring both sides of the two first equations, we have

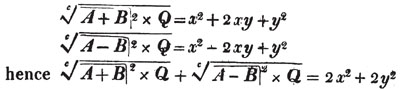which is always a whole number When the root is a binomial surd; take therefore s and t the nearest integer values of 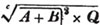and 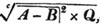one of which is greater, and the other less than the true value of the corresponding quantity; then since the sum of these surds is an integer, the fractional parts must destroy each other, and 2x² + 2y² = s + t, exactly,

I 2

[page] 132

when the root of the proposed quantity can be obtained. We have therefore these two equations

x² - y² = n

x² + y ² =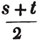

therefore,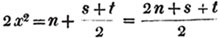

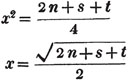
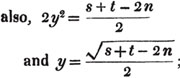
therefore, if the root of the binomial 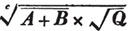be of the form x + y it is 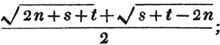and the c^th root of A + B is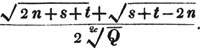

Ex. 1.

Required the cube root of 10 + √108.

In this case, A = √108, B = 10; A² - B² = 8, and 8Q = n³; if therefore Q = l, n = 2. Also,

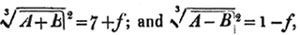where f is some fraction less than unity; therefore s = 7, t = 1; and

If therefore the cube root of 10 + √108 can be expressed in the proposed form, it is √3 + 1; which, on trial, is found to succeed.

[page] 133

Let the cube root of 11 + 5 √7 be required.

Here, A= 5 √7 B=11, A² - B² = 54; therefore 54Q = n³, and if Q = 4, n³ = 2l6, and n = 6.

Also 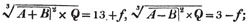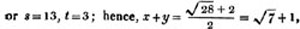and the quantity, to be tried for the root, is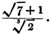

Ex. 3.

To find the cube root of 2√7 + 3√3.

Here, A = 2√7, B = 3√3, A² - B² = 1; hence Q = 1, and n = 1. 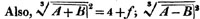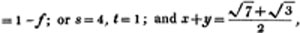the quantity to be tried for the root, which is found to succeed.

(260.) In the same manner, the c^th root of A - B is 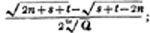in which expression, when A is less than B, n is negative.

(261.) In the operation, it is required to find a number Q, such, that 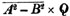may be a perfect cth power; this will be the case, if Q be taken equal to 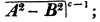but to find a less number which will answer this condition, let A² - B² be divisible by a, a, …..(m); b, b, ….. (n); d, d, ….. (r); &c. in succession, that is, let A² - B² = a^m bⁿd^r &c. also let

[page] 134

Q = a^xb^yd^z &c. then 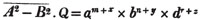. which is a perfect c^th power, if x, y, z, &c. be so assumed that m + x, n + y, r + z, are respectively equal to c, or some multiple of c. Thus, to find a number which multiplied by 180 will produce a perfect cube, divide 180 as often as possible by 2, 3, 5, &c. and it appears that 2.2.3.3.5 = 180; if, therefore, it be multiplied by 2.3.5.5, it becomes 2³.3³.5³, or a perfect cube.

If the index of the root to be extracted be an even number, the square root may be found by Art. 258, when it can be expressed by a binomial of the same description; and if half the index be an even number, the square root may again be taken, and so on, until the root remaining to be extracted is expressed by an odd number.

If A and B be divided by their greatest common measure, either integer or quadratic surd, in all cases where the c^th root can be obtained by this method, Q will either be unity, or some power of 2, less than 2^c. See Dr. Waring's Med. Alg. p. 287.

THE END OF PART I.

[page 135]

THE

ELEMENTS OF ALGEBRA.

PART II.

ON THE NATURE OF EQUATIONS.

(262.) Any equation, involving the powers of one unknown quantity, may be reduced to the form xⁿ - px^{n - 1} + qx^{n - 2}- &c. = 0; where the whole expression is made equal to nothing, the terms are arranged according to the dimensions of the unknown quantity, the coefficient of the highest dimension is unity, and the coefficients, p, q, r, &c. are affected with their proper signs.

An equation, where the index of the highest power of the unknown quantity is n, is said to be of n dimensions; and in speaking simply of an equation of n dimensions, we understand one reduced to the above form, unless the contrary be expressed.

(263.) Any quantity, xⁿ - px^{n - 1} + qx^{n - 2} … + Px - Q, may be supposed to arise from the multiplication of 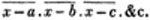. continued to n factors.

[page] 136

For, by actually multiplying the factors together, we obtain a quantity of n dimensions, similar to the proposed quantity, xⁿ - px^{n - 1} + qx^{n - 2} - &c. and if a, b, c, &c. can be so assumed that the coefficients of the corresponding terms in the two quantities become equal, the whole expressions coincide. And these coefficients may be made equal, because we shall have n equations, to determine the n quantities a, b, c, d, &c. (See Art. 145). If then the quantities, a, b, c, d, &c. be properly assumed, the equation, xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0, is the same with .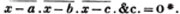

We cannot suppose xⁿ - px^{n - 1} + qx^{n - 2} - &c. to be made up of more, or fewer, then n simple factors; because, on either supposition, the result would not be of the same number of dimensions with the proposed quantity.

(264.) The quantities a, b, c, d, &c. are called roots of the equation, or values of x; because, if any one of them be substituted for x, the whole expression becomes nothing, which is the only condition proposed by the equation.

* This proof, which is usually given, is imperfect; for if the n equations be reduced to one, containing only one of the quantities, a, this equation is aⁿ - pa^{n - 2}-1 + qa^{n - 2} - &c. = 0, which exactly coincides with the proposed equation; in supposing therefore that a can be found, we take for granted the proposition to be proved. The subject has exercised the skill of the most eminent algebraical writers, but their reasonings upon it are of too abstruse a nature to be introduced in this place: The Learner must, at present, take for granted, that an equation may be made up of as many simple factors as it has dimensions; and when he is farther advanced in the subject, he may consult Dr. Waring's Algebra, page 272; Phil. Trans. 1798, page 369; and' Demonstratio Nova; C. F. Gauss, Helmstadt, 1799.

[page] 137

(265.) If the signs of the terms of an equation be all positive, it cannot have a positive root; and if the signs be alternately positive and negative, it cannot have a negative root.

If xⁿ + px^{n - 1} + qx^{n - 2} + &c. = 0, and any positive quantity, a, be substituted for x, the result is positive; consequently a is not a root of the equation.

If xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0, and a negative quantity, - a, be substituted for x, when n is an odd number the result is negative, and when n is an even number the result is positive; therefore - a cannot in either case, be a root of the equation.

(266.) Every equation has as many roots as it has dimensions.

If xⁿ - px^{n - 1} + qx^{n - 2} + &c. = 0, or 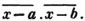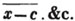to n factors = 0; there are n quantities, a, b, c, &c. each of which, when substituted for x, makes the whole = 0, because in each case one of the factors becomes = 0; but any quantity different from these, as e, when substituted for x, gives the product 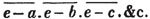which does not vanish, because none of the factors vanish; that is, e will not answer the condition which the equation requires.

(267.) When one of the roots, a, is obtained, the equation 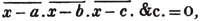, or xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0, is divisible by x - a, without a remainder, and is thus reducible to 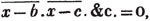an equation one dimension lower, whose roots are b, c, &c.

Ex. One root of the equation y³ + 1 = 0, is - 1, or y + 1 = 0, and the equation may be depressed to a quadratic, in the following manner:

[page] 138

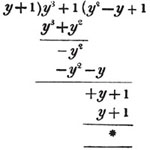
Hence, the other two roots are the roots of the quadratic y² - y + 1 = 0.

If two roots, a and b, be obtained, the equation is divisible by 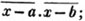; and thus it may be reduced two dimensions lower.

Ex. Two roots of the equation x⁶ - 1 = 0, are + 1 and - 1, or x - 1 = 0, and x + 1 = 0; therefore it may be depressed to a biquadratic by dividing by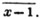

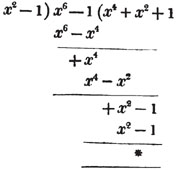
Hence, the equation x⁴ + x² + 1 = 0, contains the other four roots of the proposed equation.

(268.) Conversely, if the equation be divisible by x - a, without remainder, a is a root; if by

[page] 139

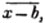a and b are both roots; &c. Let Q be the quotient arising from the division, then the equation is 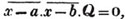, in which, if a or b be substituted for x, the whole vanishes.

(269.) COR. 1. If a, b, c, &c. be the roots of an equation, that equation is 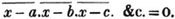. Thus, the equation whose roots are 1, 2, 3, 4, is 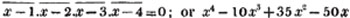+ 24 = 0. The equation whose roots are 1, 2, and 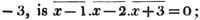or, x³ - 7x + 6 = 0.

(270.) COR. 2. If the last term of an equation vanish, it is of the form xⁿ - px^{n - 1} + qx^{n - 2} …… Px = 0, which is divisible by x, or x - 0, without remainder; therefore, 0 is one of it's roots; if the two last terms vanish, it is divisible by x², without remainder, or by 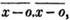, that is, two of it's roots are 0; &c.

(271.) The coefficient of the second term of an equation, with it's proper sign, is the sum of the roots with their signs changed; the coefficient of the third term, is the sum of the products of every two roots with their signs changed; the coefficient of the fourth term, is the sum of the products of every three roots, with their signs changed, &c. and the last term is the product of all the roots, with their signs changed.

Let a, b, c, &c. be the roots of the equation; then 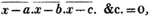, is that equation; and by Art. 232, it appears, that when these factors are multiplied together, the coefficient of the second term is the sum of the quantities - a, - b, - c, &c. the coefficient of the third term, the sum of the products of every two, &c. and the last term, which

[page] 140

does not contain x, is the product of all those quantities.

(272.) COR. 1. If the roots be all positive, the signs of the terms will be alternately + and -. For the product of an odd number of negative quantities is negative, and of an even number, positive. But if the roots be all negative, the signs of all the terms will be positive, because the equation arises from the multiplication of the positive quantities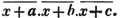

(273.) COR. 2. Let the roots of the equation xⁿ - px^{n - 1} … + Px² - Qx + R = 0, be a, b, c, d, &c. then R = abcd (n); Q = bcd (n -1) + acd (n - 1) + abd (n - 1) + &c. and Q/R = 1/a + 1/b + 1/c + &c. that is, the coefficient of the last term but one, divided by the last term, is the sum of the reciprocals of the roots. In the same manner, P/R = 1/ab + 1/ac + 1/ad + 1/bc + 1/bd + 1/cd + &c.

(274.) Any equation, it has been observed, may be conceived to arise from the multiplication of the simple factors 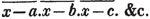or by taking two or more of these together, it may be supposed to arise from the multiplication of quadratic, cubic, &c. factors, if the dimensions of these factors, together, make up the dimensions of the proposed equation.

Thus, a cubic equation may be supposed to be the product of three simple factors, 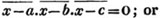of a quadratic and a simple factor, .

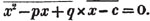[page] 141

(275.) If the coefficients, in any equation, be whole numbers, the equation cannot have a fractional root.

If possible, let a/b, a fraction in it's lowest terms, be a root of the equation xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0; then 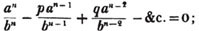or aⁿ/b = pa^{n - 1} - qa^{n - 2}b + &c. that is, aⁿ/b, a fraction in it's lowest terms (Art 190. Cor. 2), is equal to a whole number, which is absurd; therefore a/b is not a root of the equation.

(276.) The roots a, b, c, &c. of an equation are impossible, when, as is frequently the case, they involve the square root of a negative quantity.

(277.) Impossible roots enter equations by pairs.

If a + √- b² be a root of the equation xⁿ - px^{n - 1} + &c. = 0, then a - √- b² is also a root.

In the equation, for x substitute a + √- b², and the result will consist of two parts, possible quantities, which involve the powers of a and the even powers of √- b², and impossible quantities which involve the odd powers of √- b²; call the sum of the possible quantities A, and of the impossible B, then A + B is the whole result. Let now, a - √- b², be substituted for x, and the possible part of the result will be the same as before, and the impossible part which arises

[page] 142

from the odd powers of - √- b², will only differ from the former impossible part in it's sign; therefore the result is A - B; and since by the supposition a + √- b² is a root of the equation, A + B = 0; in which, as no part of A can be destroyed by B, A = 0 and B = 0; therefore A - B = 0, that is, the result, arising from the substitution of a - √- b² for x, is nothing; or a - √- b² is a root of the equation.

The truth of the proposition is also manifest from this consideration, that if x² - mx + n = 0 be a quadratic factor of the equation (Art. 274), two values of x are 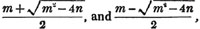which are either both possible, or both impossible.

(278.) COR. 1. Hence it follows, that an equation of an odd number of dimensions, must have, at least one possible root; unless some of the coefficients are impossible, in which case the equation may have an odd number of impossible roots.

(279.) COR. 2. By the same mode of reasoning it appears that, when the coefficients are rational, surd roots of the form ± √b, or a ± √b enter equations by pairs.

ON THE TRANSFORMATION OF EQUATIONS.

(280.) If the signs of all the terms in an equation be changed, it's roots are not altered.

[page] 143

Let 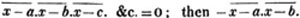vanishes when a, b, c, &c. are substituted for x.

(281.) If the signs of the alternate terms, beginning with the second, be changed, the signs of all the roots are changed.

Let xⁿ - px^{n - 1} - qx^{n - 2} + &c. = 0, be an equation whose roots are a, b, - c, &c. for x substitute - y, and, when n is an even number, yⁿ + py^{n - 1} - qy^{n - 2} - &c. = 0; but when n is an odd number, - yⁿ - py^{n - 1} + qy^{n - 2} + &c. = 0, or changing all the signs (Art. 280), yⁿ + py^{n - 1} - qy^{n - 2} - &c.= 0, as before; and since x = - y, or y = - x, the values of y are - a, - b, + c, &c.

Ex. Let it be required to change the signs of the roots of the equation x³ - qx + r = 0.

This equation with all it's terms is x³ + 0 - qx + r = 0; and changing the signs of the alternate terms, we have x³ - 0 - qx - r = 0, or x³ - qx - r = 0, an equation whose roots differ from the roots of the former, only in their signs.

(282.) To transform an equation into one whose roots are greater, or less than the corresponding roots of the original equation, by any given quantity.

Let the roots of the equation x³ - px² + qx - r = 0, be a, b, c; to transform it into one whose roots are a + e, b + e, c + e.

Assume x + e = y, or x = y - e; then,

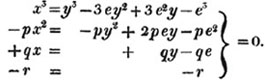
[page] 144

In this last equation, since y = x + e, the values of y are a + e, b + e, c + e.

If y + e be substituted for x, the values of y in the resulting equation will be a - e, b - e, c - e.

(283.) In general, let the roots of the equation xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0, be a, b, c, &c. Assume y = x - e, or x = y + e, and by substitution,

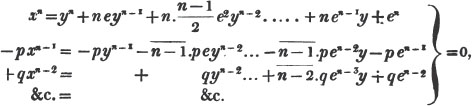
and since y = x - e, the values of y, in this equation, are a - e, b - e, c - e, &c.

We may observe, that the last term of the transformed equation, eⁿ - pe^{n - 1} + qe^{n - 2} - &c. is the original quantity, with e in the place of x; the coefficient of the last term but one, with it's proper sign, is obtained by multiplying every term of eⁿ - pe^{n - 1} + qe^{n - 2} - &c. by the index of e in that term, and diminishing the index by unity; the coefficient of the last term but two, 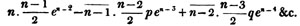is obtained in the same manner from the coefficient of the last term but one, dividing every term by 2; &c.

(284.) One use of this transformation is, to take away any term out of an equation. Thus, to transform an equation into one which shall want the second term, e must be so assumed that ne - p = 0, or e = p/n (where p is the coefficient of the second term

[page] 145

with it's sign changed, and n the index of the highest power of the unknown quantity); and if the roots of the transformed equation can be found, the roots of the original equation may also be found, because x = y + p/n.

Ex. 1. Let x² - px + q = 0 be the proposed equation. Assume x = y + p/2, then

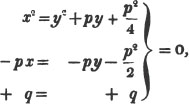
or y² - p²/4 + q = 0; hence, y² = p²/4 - q, and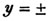

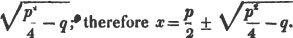
Ex. 2. To transform the equation x³ - 9x² + 7x + 12 = 0 into one which shall want the second term.

Assume x = y + 3, then

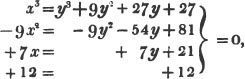
that is, y³ - 20y - 21 = 0; and if the values of y be a, b, c, the values of x are a + 3, b + 3, and c + 3.

(285.) To take away the third term of the equation, e must be so assumed, that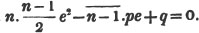

K

[page] 146

In this case we shall have a quadratic to solve; and in general, to take out the m^th term, by this method, it will be necessary to solve an equation of m - 1 dimensions.

Ex. To transform the equation x³ - 6x² + 9x - 1 = 0 into one which shall want the third term.

Here n = 3, p = 6 and q = 9; therefore 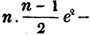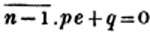becomes 3e² - 12e + 9 = 0, or e² - 4e + 3 = 0, in which the values of e are 1 and 3. Let x = y + 3, then

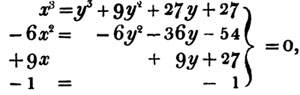
that is, y³ + 3y² - 1 = 0. In the same manner, if x = y + 1, the transformed equation will want the third term.

(286.) To transform an equation into one whose roots are the roots of the original equation multiplied by any given quantity.

Let the roots of the equation xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0, be a, b, c, &c. to transform it into one whose roots are ma, mb, mc, &c.

Assume y = mx, or x = y/m; then substitute this value for x, and the equation becomes 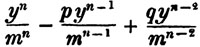- &c. = 0, or multiplying by mⁿ, yⁿ - mpy^{n - 1} + m²qy² - &c. = 0, an equation whose roots are ma, mb, mc, &c.

[page] 147

This equation differs from the former, only in having the successive terms multiplied by 1, m, m², m³, &c.

(287.) COR. 1. By this transformation, an equation may be cleared of fractions; or if the first term be affected with a coefficient, that coefficient may be taken away.

Ex. 1.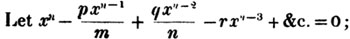 by multiplication, mnxⁿ - npx^n-1 + mqx^n-2 - mnrx^n-3 + &c. =0; transform this equation into one whose roots are mn times as great, and mnyⁿ- mn²py^n-1 + m³n²qy^n-2 - m⁴n⁴ry^n-3 + &c. = 0, or yⁿ - npy^{n - 1} + m²nqy^{n - 2} - m³n³ry^{n - 3} + &c. = 0, an equation of the usual form.

Ex. 2. Let it be required to transform the equation 3y³ - qy + r = 0 into one in which the coefficient of the highest term is unity.

The equation with all it's terms is 3y³ + 0 - qy + r = 0; transform it into one whose roots are three times as great, by substituting z/3 fory; then 3z³ + 3 × 0 - 9qz + 27r = 0, or z³ - 3qz + 9r = 0, an equation of the required form.

(288.) COR. 2. In any equation, if the coefficients of the second, third, fourth terms, &c. be divisible, respectively, by m, m², m³, &c. the roots have a common measure, m.

(289.) COR. 3. An equation may be transformed into one whose roots are 1/m parts of the roots of the

K 2

[page] 148

former, by dividing the second, third, fourth, &c. terms by m, m², m³, &c. respectively.

(290.) To transform an equation into one whose roots are the reciprocals of the roots of the given equation.

Let the roots of the equation xⁿ - px^n-1 + qx^n-2 …. - Px + Q=0, be a, b, c, &c. to transform it into one whose roots are 1/a, 1/b, 1/c, &c.

Assume y = 1/x or x = 1/y; then, by substitution, 1/yⁿ - p/y^{n - 1} + q/y^{n - 2} …. - P/y + Q = 0, and multiplying by yⁿ, 1 - py + qy² … Py^{n - 1} + qyⁿ = 0; that is Qyⁿ - Py^n-1 …. + qy² - py + 1 = 0; and since y = 1/x, the values of y are1/a, 1/b, 1/c, &c.

(291.) COR. l. If any term in the given equation be wanting, the corresponding term will be wanting in the transformed equation; thus, if the original equation want the second term, the transformed equation will want the last term but one, &c. because the coefficients in the transformed equation are the coefficients in the original equation, in an inverted order.

(292.) COR. 2. If the coefficients of the terms, taken from the beginning of an equation, be the same with the coefficients of the corresponding terms, taken from the end, with the same signs, the transformed will coincide with the original equation, and their roots will therefore be the same.

[page] 149

Let a, b, c, roots of the equation xⁿ - px^n-1 + qx^n-2 …. + qx² - px + 1= 0; the transformed equation will be yⁿ - py^n-1 + qy^n-2 …. + qy² - py + 1 = 0, and a, b, c, must also be roots of this equation; but the roots of this equation are the reciprocals of the roots of the original equation, therefore 1/a, 1/b, 1/c, are also roots of the original equation.

Ex. The roots of the equations, x⁴ - px³ + qx² - px + 1=0; x⁴ + qx² +1=0; and x⁴ + l=0, are of the form a, b, 1/a, 1/b.

(293.) COR. 3. If the equation be of an odd number of dimensions, or if the middle term of an equation of an even number of dimensions be wanting, the same thing will hold when the signs of the corresponding terms, taken from the beginning and end, are different.

Ex. The roots of the equation x³ - px² + px - 1=0, are of the form 1, a, 1/a. For in this case, if the signs of all the terms of the transformed equation be changed, it will coincide with the original equation; and by changing the signs of all the terms, we do not alter the roots. (Art. 280).

(294.) The equations described in the two last corollaries are called recurring equations.

(295.) COR. 4. One root of a recurring equation of an odd number of dimensions, will be +1, or - 1,

[page] 150

according as the sign of the last term is - or +; and the rest will be of the form a, 1/a, b, 1/b; &c.

For if + 1, in the former case, and -1, in the latter, be substituted for the unknown quantity, the whole vanishes; thus, if x⁵ - px⁴ + qx³ - qx³ + px - 1 = 0, and for x we substitute +1, it becomes 1- p + q - q+p - 1= 0; and it appears from Art. 290, that if a, b, c, &c. be roots of the equation, 1/a, 1/b, 1/c, &c. are also roots.

(296.) To transform an equation into one whose roots are the squares of the roots of the given equation.

Let xⁿ - px^n-1 + qx^n-2 - rx^n-3 + sx ^n-4 - &c. = 0; by transposition, xⁿ+qx^{n - 2} + sx^{n - 4} + &c. = px^{n - 1} + rx^{n - 3} +&c.; and by squaring both sides, x²ⁿ + 2qx^2n-2 + 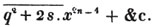= p²x^2n-2 + 2prx^2n-4 + &c. and again by transp. 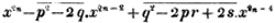- &c. = 0; assume y = x², then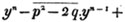 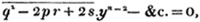in which equation, the values of y are the squares of the values of x.

(297.) COR. If the roots of the original equation be a, b, c, &c. then p² - 2q = a² + b² + c² + &c. q² - 2pr + 2s = a²b² + a²c² + &c. (Art. 271).

Other transformations may be seen in Dr. Waring's Meditationes Algebraicœ, Prob. 5; and indeed, whoever would fully understand the nature of equations, must have recourse to that Work.

[page] 151

ON THE LIMITS OF THE ROOTS OF EQUATIONS.

(298.) If a, b, c, - d, &c. be the roots of an equation, taken in order, that is, a greater than b, b greater than c, &c.* the equation is 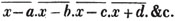= 0; in which, if a quantity greater than a be substituted for x, as every factor is, on this supposition, positive, the result will be positive; if a quantity less than a, but greater than b, be substituted, the result will be negative, because the first factor will be negative and the rest positive. If a quantity between b and c be substituted, the result will again be positive, because the two first factors are negative and the rest positive, and so on. Thus, quantities which are limits to the roots of an equation, or between which the roots lie, if substituted, successively, for the unknown quantity, give results alternately positive and negative.

(299.) Conversely, if two magnitudes, when substituted for the unknown quantity, give results affected with different signs, an odd number of roots must lie between them; and if a series of magnitudes, taken in order, can be found, which give as many results, alternately positive and negative, as the equation has dimensions, these must be limits to the roots of the equation; because an odd number of roots lies between

* In this series, the greater d is, the less is -d. And whenever a, b, c, - d, &c. are said to be the roots of an equation taken in order, a is supposed to be the greatest. Also, in speaking of the limits of the roots of an equation, we understand the limits of the possible roots.

[page] 152

each two succeeding terms of the series, and there are as many terms as the equation has dimensions; therefore this odd number cannot exceed unity.

(300.) If the results arising from the substitution of two magnitudes, for the unknown quantity, be both positive or both negative, either no root of the equation, or an even number of roots, lies between them.

(301.) COR. If m, and every quantity greater than m, when substituted for the unknown quantity, give positive results, m is greater than the greatest root of the equation.

(302.) To Jind a limit greater than the greatest root of an equation.

Let the roots of the equation be a, b, c, &c. transform it into one whose roots are a - e, b - e, a - e, &c. and if, by trial, such a value of e be found, that every term of the transformed equation is positive, all it's roots are negative (Art. 265), and consequently e is greater than the greatest root of the proposed equation.

Ex. 1. To find a number greater than the greatest root of the equation x³ - 5x² + 7x - 1 = 0.

Assume x = y + e, and we have

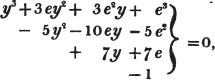
in which equation, if 3 be substituted for e, each of the quantities, e³ - 5 e² + 7^e -1, 3e² - 10e + 7, 3e - 5, is positive, or all the values of y are negative; therefore 3 is greater than the greatest value of x.

[page] 153

Ex. 2. In any cubic equation of this form, x³ - qx + r = 0, Aq is greater than the greatest root.

By transforming the equation, as before,

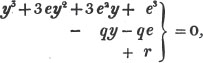
and substituting √q for e, y³ + 3√qy² + 2qy + r = 0, every term of which is positive; therefore √q is greater than the greatest value of x.

(303.) COR. If the signs of the roots be changed, a limit greater than the greatest root of the resulting equation, with it's sign changed, is less than the least root of the proposed equation.

Ex. Required a limit less than the least root of the equation y³ - 3y + 72 = 0.

When the signs of the roots are changed, this equation becomes y³ - 3y - 72 = 0.

Assume y = x + e; then

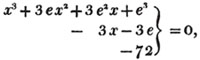
and if 5 be substituted for e, every term becomes positive, consequently 5 is greater than the greatest root of the equation y³ -3y - 72 = 0; and - 5 less than the least root of the equation y³ - 3y + 72 = 0.

(304.) The greatest negative coefficient increased by unity, is greater than the greatest root of an equation.

Let xⁿ - px^{n - 1}- qx^{n - 2} - &c. = 0, and if the coefficients be equal, xⁿ - px^{n - 1} - px^{n - 2} - &c. = 0, or x

[page] 154

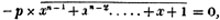that is,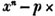 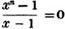(Art. 222). In this equation substitute 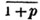for x, and the result is 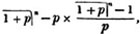or + 1; and if any of the coefficients be positive, or less than p, the sum of the series, to be taken from xⁿ, will be diminished, and the result greater than before. Also, if for x, any quantity, still greater, be substituted, as p + m + 1, the result is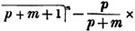 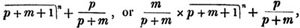a positive quantity; therefore, 1 + p is greater than the greatest root (Art. 301).

(305.) In any equation, xⁿ - px^{n - 1} + qx^{n - 2} - rx^{n - 3} + sx^{n - 4} - &c. = 0, whose roots are possible and positive, 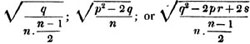is less than the greatest root.

Let a, b, c &c. be the roots, taken in order; then ab + ac + bc + &c. = q, and a² is greater than each of these products, therefore 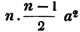(Art. 230), is. greater than q, or a is greater than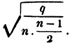

[page] 155

Also, a² + b² + c² + &c. = p² - 2q (Art. 297); and since a is the greatest root, na² is greater than a² + b²+ c² + &c. or p² - 2q; that is, a is greater than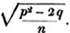

Again, q² - 2pr + 28 = a²b² + a²c² + b²c² + &c. (Art.297), and a⁴ is greater than each of these products, of which there are 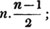therefore 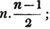a⁴ is greater than a²b²+a²c²+ b²c²+ &c. or q² - 2pr + 2s; that is, a is greater than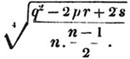

(306.) COR. If the equation have both positive and negative roots, and - d be the least root; then, when d is greater than the greatest root, it is greater than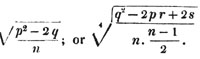

(307.) The roots of the equation 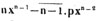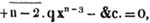are limits between the roots of the equation xⁿ - px^{n -1} +qx^{n - 2} - &c. = 0, when the roots of the latter equation are possible.

Let the roots of this equation, taken in order, be a, b, c, - d, &c. and in it, for x, substitute y + e, then by Art. 283,

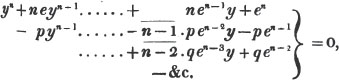
[page] 156

the roots of which equation are a - e, b - e, c - e, - d - e, &c. and the coefficient of the last term but one of any equation of n dimensions, is the sum of the rectangles under n - 1 roots, with their signs changed (Art. 271); therefore,

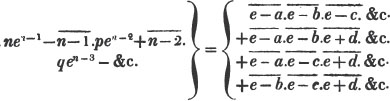
in which, if a, b, c, - d, &c. be successively substituted for e, the results are,

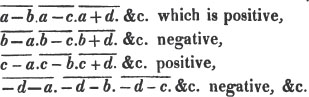
therefore a, b, c, - d are limits to the roots of the equation (Art. 299), or, substituting x for e, to the roots of the equation 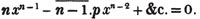Let α, β, γ, &c.be the roots of this equation, taken in order, then a, α, b, β, c, γ, - d, &c. are arranged according to their magnitudes, that is, α, β, γ, &c. lie between the roots of the equation xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0.

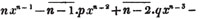
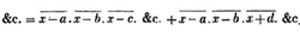
(308.) COR. 1. It appears from the preceding demonstration, that 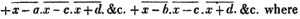a, b, c, - d, &c. are the roots of the equation xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0; also xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 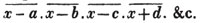therefore,

[page] 157

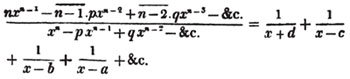
(309.) COR. 2. If the limiting equation to nx^{n -1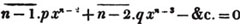} - be taken, it's roots will lie between the first and third, second and fourth, &c. roots of the first equation.

(310.) COR. 3. The original equation has as many positive roots, and as many negative, as the limiting equation, and one more, which will be positive or negative according to the nature of the equation.

(311.) Every equation whose roots are possible, has as many changes of signs from + to -, and from - to +, as it has positive roots; and as many continuations of the same sign, from + to +, and from - to -, as it has negative roots.

Let xⁿ - px ^{n - 1}. … ± Sx² ± Px ± Q = 0, the equation of limits is 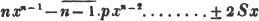± P = 0, which, as far as it goes, has the same signs with the former; and therefore the original equation will have one more change of signs, or one more continuation of the same sign, than the limiting equation, according as the signs of P and Q are different, or the same.

Suppose α, β, γ, &c. to be the roots of the limiting equation; then the roots of the original equation are, by Art. 310, of this form, a, b, c, ± d, &c. therefore, P, with it's proper sign, = n×- α×- β×- γ &c. and Q = - ax - bx - cx 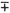dx &c. (Art.271), which pro

[page] 158

ducts will have the same sign when the multiplier d is positive, or the root (- d) negative, and different signs when that root is positive. It appears then, that if the original equation have one more change of signs than the limiting equation, it has one more positive root; and, if it have one more continuation of the same sign, it has one more negative root; therefore if it can be shewn that every equation of n- 1 dimensions, and consequently the equation nx^{n - 1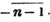} px^{n - 2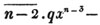} + &c. = 0, which is the limiting equation to xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0, has as many changes of signs as it has positive roots, and as many continuations of the same sign as it has negative roots, the same rule will be true in the equation xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0; or, in other words, if the rule be true of every equation of one order, it is true of every equation of the next superior order. Now in every simple equation x-a = 0, or x + a = 0, the rule is true, therefore it is true in every quadratic x²± px ± q = 0; and if it be true in every quadratic, it is true in every cubic; and so on; that is, the rule is true in all cases.

In the demonstration, each root, ± d, is supposed to be distinct from the rest, and a possible quantity.

Hence, when all the roots are possible, the number of positive roots is exactly known.

Ex. The equation x³ + x² - 14x +8 = 0, has two positive and one negative root; because the signs are +, +, -, +, in which there are two changes, one from + to -, and the other from - to +, and one continuation of the sign +.

(312.) When any coefficient vanishes, it may be considered either as positive or negative, because the value of the whole expression is the same on either supposition.

[page] 159

Ex. If the roots of the equation x³ - qx + r = 0 be possible, two of them are positive and the third is negative; for there are two changes of signs in the equation x³± 0 - qx + r = 0, and one continuation of the same sign.

(313.) If all the roots of an equation be positive, or all negative, and it's terms be multiplied by the terms of any arithmetical progression, the resulting equation will be a limiting equation to the former.

Let the roots of the equation xⁿ - px ^{n - 1} + qx^{n - 2} - &c = 0, taken in order, be a, b, c, &c. and when they are substituted for x in the quantity nx^{n - 1} - n - 1. px^{n - 2}+ &c. let the results be +L, - M, + N, - &c. (Art. 307.); then, when they are substituted in 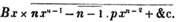the results will be + BaL, - BbM, + BcN, - &c. but when the same quantities are substituted for x in xⁿ - px^{n - 1} -qx^{n - 2} - &c. or in Axⁿ - Apx^{n - 1} + Aqx^{n - 2} - &c. the resultsare nothing; therefore when they are substituted in the sum of Axⁿ - Apx^{n - 1} + Aqx^n-3 - &c. and Bx×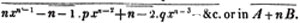 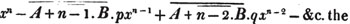results are + BaL, - BbM, + BcN, - &c. therefore (Art. 299), a, b, c, &c. are limits of the roots of the equation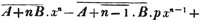 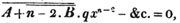which is deduced from the former by multiplying it's terms by the terms of the arithmetical progression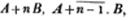

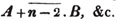
[page] 160

Conversely, the roots of this latter equation are limits to the roots of the former.

If B be negative, or the series an increasing one, the results will be, - BaL, + BbM, - BcN, + &c. therefore a, b, c, &c. are limits to the roots of the equation, 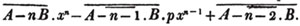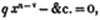as before; only a will be less than it's greatest root.

(314.) If an equation have both, positive and negative roots, and it's terms be multiplied by the terms of an arithmetical progression, an equation arises, whose roots are limits to the roots of the former, with this exception, that either two of it's roots, or none, lie between the positive and negative roots of the original equation, according as a decreasing or increasing progression is used.

Let the roots of the equation xⁿ - px^{n - 1} - qx^{n - 2} - &c. = 0, taken in order, be a, - b, - c, &c. When these values are substituted for x in 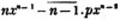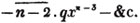the results are, + L, - M, + N, - &c. (Art. 307), therefore when they are substituted in Bx×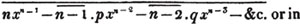 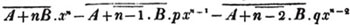the results are +BaL, +BbM, - BcN, +&c. Now the roots of the equation 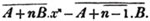P x^n-1 - &c. = 0 are of the same form with the roots of the original equation*, because there is the same number of changes of signs in both; let these roots, taken in order, be α, - β, - γ, &c. and since both

* Here we suppose that the first term is not taken out, and that the signs of the terms of the progression are not changed.

[page] 161

a, and - b, when substituted in the limit, give positive results, either two roots α, and -β, or none, lie between them (Art. 300); and - b, a negative quantity, cannot be greater than α, a positive one; therefore the order of the magnitudes is a, α, - β, -b, - γ - c, &c. that is, when the terms of the equation are multiplied by the terms of the decreasing arithmetical progression, 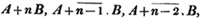two roots, α, and - β, of the limiting equation, lie between the positive and negative roots, a and - b of the original equation.

When B is negative, and nB less than A, or the series an ascending one, it may be proved, as before, that when a, - b, - c, &c. are substituted for x, in 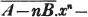the results are - BaL, - BbM, + BcN, - &c. therefore a is less than α, and either two roots -β, and -γ or none, lie between aand - b; there cannot be two, because then - b, and - c, are both less than -γ, and when substituted for x, must give results affected with the same sign; but the results are - BbM, and + BcN; therefore the order of the roots is α, a, - b, -β, - c, γ, - &c. that is, no root of the limiting equation lies between a, and - b. If A be less than nB, the first term of the limiting equation is negative, and the signs of all the terms being changed, to reduce the equation to a proper form (Art. 262), the series becomes a decreasing one.

(315.) In the preceding demonstration, the limiting equation is supposed to contain as many roots as the original equation, and of the same form. If the

L

[page] 162

arithmetical progression begin from nothing, it may be proved in the same manner, that no root of the limit, thus deduced, lies between the positive and negative roots of the proposed equation.

(316.) Ex. Let the proposed equation be x³. The roots of the equation 3x² - q = 0, are limits which lie between it's roots (Art. 307.)

Let the terms of the equation be multiplied by the series, 3, 2, 1, 0, and the limiting equation is 3x³ - qx = 0, whose roots are √q/3, 0, - √q/3, two of which, 0 and - √q/3 lie between the positive and negative roots of the proposed equation (Art. 314).

Let the terms be multiplied by the series 0, -1, -2, -3, the limiting equation thus obtained is 2qx - 3r = 0, whose root 3r/2q lies between the positive roots of the equation X³ - qx + r = 0. (Art. 315).

(317.) To find between which of the roots, of a proposed equation, any given number lies.

Let the roots of the proposed equation be diminished by the given number, and the number of negative roots, in the transformed equation, will shew it's place among the roots of the original equation.

Ex. To find between which of the roots of the equation x³ - 9x² + 23x - 15 = 0, the number 2 lies.

[page] 163

Assume x = y + 2; then,

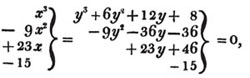
or y³ - 3y² - y + 3 = 0, which has one negative root; and the roots of the proposed equation are all positive; therefore two of them are greater, and one is less than 2.

(318.) In general, the last term., and the coefficients of the other terms of the transformed equation are found by substituting the number, by which the roots are to be diminished, for x, in the quantities,

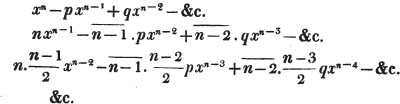
See Art. 283. And by substituting, successively, different numbers for x, the limits of the roots of the proposed equation may be found.

Ex. Let the proposed equation be x⁴ - 2x³ - 5x² + 10x - 3 = 0.

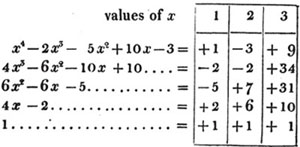
L 2

[page] 164

From the changes of signs in the proposed equation, it appears, that it has one negative and three positive roots; when these roots are diminished by 1, they become two positive and two negative; when diminished by 2, they become one positive and three negative; and when diminished by 3, they all become negative; therefore one root of the proposed equation lies between 0 and 1, one between 1 and 2, and the third between 2 and 3.

By changing the signs of the roots, and proceeding in the same way, we may find, that the negative root lies between - 2, and - 3.

ON THE DEPRESSION AND SOLUTION OF EQUATIONS.

(319.)If an equation contain equal roots, these may he found, and the equation reduced as many dimensions lower as there are equal roots.

Let the roots of the equation xⁿ - px^{n - 1} + qx^{n - 2} - &c. = 0, be a, b, c, &c. then (Art. 307),

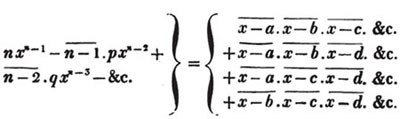
Suppose a = b; then,

[page] 165

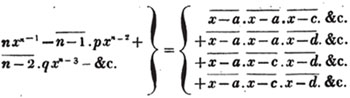
the whole of which is divisible by x - a without remainder, that is, a is a root of the equation nx^{n - 1 -}*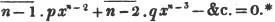

If three roots a, b, c, be equal, 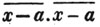will be found in every product, therefore the equation is divisible by 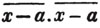without remainder, or two of it's roots are a, a (Art. 268).

In the same manner, if the original equation have m equal roots, the equation 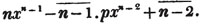qx^{x - 3} - &c. = 0, has m - 1 of those roots.

(320.) Hence it appears, that when there are m equal roots, the two equations have a common measure of the form 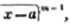which may be obtained in the usual way (Art. 90), and m roots of the original equation may thus be known. Divide this equation by 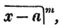and the resulting equation, of n - m dimensions, contains the other roots.

Ex. Let the equation x³ - px² + qx - r = 0, have two equal roots; then 3x² - 2px + q = 0 has one of them; and the two equations have a common measure which is a simple equation; consequently, the quantities 9x³ - 9px² + 9qx - 9r and 3x² - 2px + q, have the same common measure, which is thus found,

* The equation 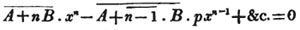has also one of the equal roots. See Art. 313.

[page] 166

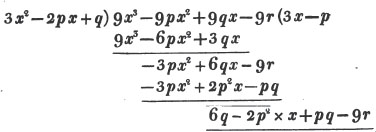
hence, 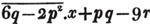is a divisor of the equation x³ - px² + qx - r = 0; that is, 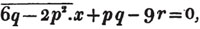and 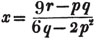(Art. 268).

Thus two roots of the equation are discovered; and since p is the sum of all the roots, the third root is the difference between p and the sum of the two equal roots.

Let the proposed equation be x³ - 4x² + 5x - 2 = 0.

Here, p = 4, q = 5, r = 2; and one of the equal roots is 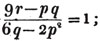and the third root is p - 2 = 4 - 2 = 2.

But it must be observed, that though 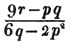should be found, upon trial, to be a root of the proposed cubic, this equation has not two equal roots, unless 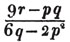be a root of the depressed equation 3x² - 2px + q = 0.

(321.) If the roots of the equation x³ - px² + qx - r = 0, be in arithmetical progression,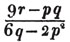 is one of them.

[page] 167

Let the roots be a - b, a, a + b; then p = 3a, q = 3a² -b², and r = a³ - ab²; hence, 9r - pq = - 6 ab², and 6q - 2p² = - 6b²; therefore, 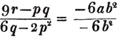= a.

(322.) If two roots of an equation be of the form +a, - a, differing only in their signs, they may be found, and the equation depressed.

Change the signs of the roots, and the resulting equation has two roots +a, - a; thus we have two equations with a common measure, x² - a², which may be found, and the equation depressed, as in the preceding case.

Ex. Required the roots of the equation x⁴ + 3x³ 7x² + 27x - 18 = 0, two of which are of the form + a, - a. By changing the signs of the alternate terms we obtain the equation x⁴ - 3x³ - 7x² + 27x - 18 = 0, which has two roots of the form +a, - a; and the common quadratic divisor, of the two equations, is x² - 9 = 0, hence, x = ± 3. To obtain the other roots, divide x⁴ + 3x³ - 7x² - 27x -18 = 0, by x² - 9 = 0, and the roots of the resulting equation x²+3x + 2 = 0, are the roots sought.

(323.) By this method, when the coefficients are rational, surd roots of the form ± √a may be discovered (See Art. 279)

(324.) When there are two other roots of the same form, the equations will have a common divisor x⁴ - Qx² + R = 0, which contains the four roots, a, - a, b, - b.

[page] 168

If the roots of an equation have any other given relation, they may be found in a similar manner (See Waring's Alg. Cap. 3); but as particular relations of the roots to each other are rarely known, it seems unnecessary to prosecute the subject farther, in this place,

SOLUTIONS OF RECURRING EQUATIONS.

(325.) The roots of a recurring equation of an even number of dimensions, exceeding a quadratic, may be found by the solution of an equation of half the number of dimensions.

Let xⁿ - px ^{n -1} …… - px + 1 = 0; it's roots are of the form a, 1/a, b, 1/b, &c. (Art. 292); or it may be conceived to be made up of quadratic factors, 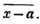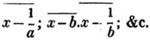i.e. if m = a + 1/a, n = b + 1/b, &c. of the quadratic factors, x² - mx + 1; x² - nx +1; &c. Then by multiplying these together, and equating the coefficients with those of the proposed equation, the values of m, n, &c. may be found. Moreover, for every value of m there are two values of x; therefore the equation for determining the value of m, will rise only to half as many dimensions as x rises in the original equation.

[page] 169

(326.) If the recurring equation be of an odd number of dimensions, +1, or - 1 is a root (Art. 295); and the equation may therefore be reduced to one of the same kind, of an even number of dimensions, by division.

Ex. 1. Let x³ -1 = 0. Unity is one root of this equation, and by dividing x³ - 1 by x - 1, the equation x² + x + 1 = 0 is obtained, which contains the other two roots, and

x² + x + 1 = 0

x² + x + 1/4 = 1/4 - 1 = - 3/4

x + 1/2 = ± √-3/2,

or 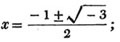that is, the three roots of the equation x³ - 1 = 0, or the three cube roots of 1, are 1,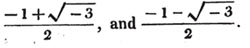

In the same manner, the roots of the equation x³ + 1 = 0 are found to be - 1, 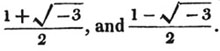This also follows from Art. 281.

Ex. 2. Let x⁴ - 1 = 0. Two roots of this equation are +1, - 1; and by division, 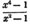= x³ + 1 = 0, an equation which contains the other two roots, + √-1, and -√-1.

[page] 170

Ex.3. Let x⁴ + 1 = 0. Assume 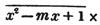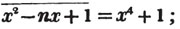= x⁴ + 1; that is,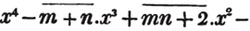 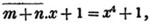and by equating; the coefficients, m + n = 0, and mn + 2 = 0; hence n = - m, and -m² + 2 = 0, or m² = 2, and m = ± √2. Therefore the two quadratics which contain the roots of the biquadratic, are x² + √2.x + 1 = 0, and x² - √2.x + 1 = 0, from the solution of which it appears that the roots are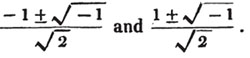

In the same manner may the roots of the equations x⁵ + 1 = 0, and x⁶ + 1 = 0, be found.

THE SOLUTION OF A CUBIC EQUATION BY CARDAN's RULE.

(327.) Let the equation be reduced to the form x³ - qx + r = 0, where q and r may be positive or negative.

Assume x = a + b, then the equation becomes 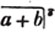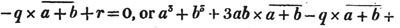r = 0; and since we have two unknown quantities, a and b, and have made only one supposition respecting them, viz. that a + b = x, we are at liberty to make another; let 3ab - q = 0, then the equation becomes a³ + b³ + r = 0; also, since 3ab - q = 0, b = q / 3a, and by

[page] 171

substitution, a³ + q3/27a³ + r = 0, or a⁶ + ra³ + q3/27 = 0, an equation of a quadratic form; and by completing the square, a⁶ + ra³ + r² /4 - q³/27, and a³ + r/2 = ± 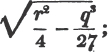therefore 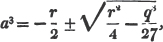and a = 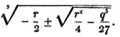Also, since a³ + b³ + r = 0, b³ 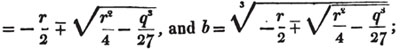and b = 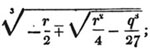therefore x = a + b =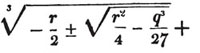

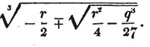
We may observe, that when the sign of √r2/4 - q3/27, in one part of the expression, is positive, it is negative in the other, that is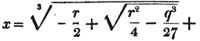

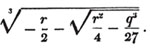
(328.) Since b = q / 3a, the value of x is also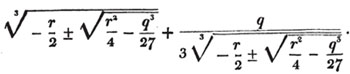

[page] 172

Ex.

Let x³ + 6x - 20 = 0; here q = - 6, r = - 20, 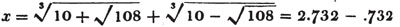= 2*.

(329.) COR. 1. Having obtained one value of x, the equation may be depressed to a quadratic, and the other roots found (Art. 267).

(330.) COR. 2. The possible values of a and b being discovered, the other roots are known without the solution of a quadratic.

The values of the cube roots of a³ are a,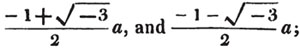 and the values of the cube root of b³, are b, 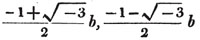(Art. 326). Hence it appears, that there are nine values of a + b, three only of which can answer the conditions ozf the equation, the others having been introduced by involution. These nine values are,

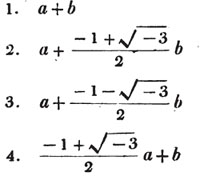
* See Art. 259.

[page] 173

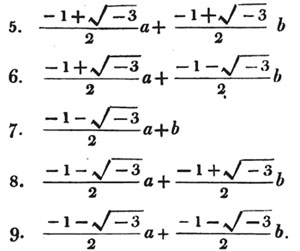
In the operation, we assume 3ab = q, that is, the product of the corresponding values of a and b is supposed to be possible. This consideration excludes the 2^d. 3^d. 4^th. 5^th. 7^th. and 9^th. values of a + b, or x; therefore the three roots of the equation are a + b,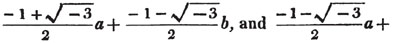

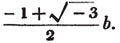
The value of xis also a + q/3a; therefore, if a, α a, β a, be the three roots of a³, the roots of the cubic are a + q/3a; αa + q/3α; βa + q/3β α.

(331.) COR. 3. This solution only extends to those cases in which the cubic has two impossible roots.

For if the roots be m + √3n, m - √3n, and -2 m, then - q (the sum of the products of every two with their signs changed) = - 3m² -3n, and q/3 = m² + n;

[page] 174

also, r (the product of all the roots with their signs changed) = 2m³ - 6mn, and r/2 = m³ - 3mn; and by involution,

r²/4 = m⁶ - 6 m⁴n + 9 m²n²

q³/27 = m⁶ + 3 m⁴n + 3 m²n² + n³

Hence, r²/4 = q³/27 = -9m⁴n + 6m²n²-n³ = 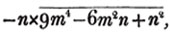and 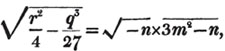a quantity manifestly impossible, unless n be negative, that is, unless two roots of the proposed cubic be impossible.

SOLUTION OF A BIQUADRATIC BY DES CARTES's METHOD.

(332.) Any biquadratic may be reduced to the form x⁴ + qx² + rx + s = 0, by taking away the second term (Art. 284). Suppose this to be made up of the two quadratics, x² + ex + f = 0, and x² - ex + g = 0, where + e and - e are made the coefficients of the second terms, because the second term of the biquadratic is wanting, that is, the sum of it's roots is 0. By multiplying these quadratics together, we have 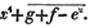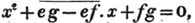which equation is made to coincide with the former, by equating their coefficients, or making g + f = q + e² = q, eg - ef = r, and fg = s; hence, g + f = y + e², also g - f = r/e, and by taking the sum and difference of these equations, 2g = q + e² +

[page] 175

r/e, and 2f = q + e² - r/e; therefore 4fg = q² + 2qe² + e⁴ - r²/e² = 4s, and multiplying by e², and arranging the terms according to the dimensions of e, e⁶ + 2qe⁴ + 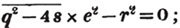or, making y = e², y³ + 2qy² +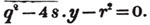

By the solution of this cubic, a value of y, and therefore of √y, or e, is obtained; also f and g, which are respectively equal to 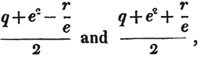are known; the biquadratic is thus resolved into two quadratics, whose roots may be found.

It may be observed, that which ever value of y is used, the same values of x are obtained.

(333.) This solution can only be applied to those cases, in which two roots of the biquadratic are possible and two impossible.

Let the roots be then since e, the coefficient of the second term of one of the reducing quadratics, is the sum of two roots, it's different values are 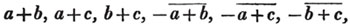and the values of e², or ^y, are 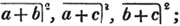all of which being possible, the cubic cannot be solved by any direct method. Suppose the roots of the biquadratic to be a + b√-1, a - b√, - a + c√-1, - a - c√-1; the values of e are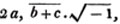 and -2a; and the three values of y are,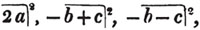

[page] 176

which are all possible, as in the preceding case. But if the roots of the biquadratic be a + b √-1, a - b√-1, - a + c, - a - c, the values of y are 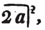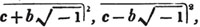two of which are impossible; therefore the cubic may be solved by Cardan's rule.

DR. WARING's SOLUTION.

(334.) Let the proposed biquadratic be x⁴ + 2px³ = qx² + rx + s; now 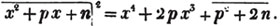x² + 2pnx + n², if therefore 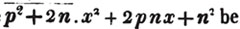be added to both sides of the proposed biquadratic, the first part is a complete square, 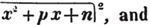the latter part, 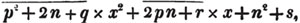is a complete square, if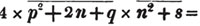 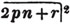(Art. 127), that is, multiplying and arranging the terms according to the dimensions of n, if 8 n³ + 4qn² + 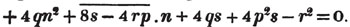From this equation, let a value of n be obtained and substituted in the equation 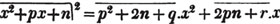+ n² + s; then extracting the square root on both sides,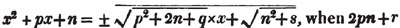 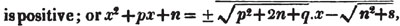when 2pn + r is negative; and from these two quadratics, the four roots of the given biquadratic may be determined.

[page] 177

Ex. Let x⁴ - 6x³ + 5x² + 2x - 10 = 0 be the proposed equation.

By comparing this with the equation x⁴ + 2px³ - qx² - rx - 8 =, we have 2p = - 6, or p = - 3, q = -5, r = -2, s = 10; and 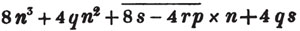+ 4p²s - r² = 0, is 8n³ - 20n² + 56 n +156 = 0, or 2n³ -5n² +14n+ 39 = 0, one of whose roots is -3/2; hence, 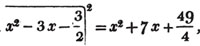and x² - 2x + 2 = 0; roots of these quadratics, - 1, 5, 1 + √-1, 1 - √ -1, are the roots of the proposed biquadratic.

(335.) This solution can only be applied to those cases in which two roots of the biquadratic are possible, and two impossible.

Let the roots be a, b, c, d, then n - √n² + s, the last term of one of the quadratics, to which the equation is reduced, is the product of two of them, as ab; therefore n - ab = √n² + S, and squaring both sides, n² - 2nab + a²b² = n² + s, or - 2nab + a²b² = s = - abcd (Art. 271), and dividing both sides by - ab, 2 n - ab - cd, or 2n = ab + cd, and 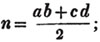the other values of n are 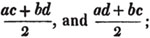therefore; when a, b, c, d, are possible, the values of n are possible. Also, when these quantities are all impossible, the values of n are all possible; in neither case therefore

M

[page] 178

can the value of n be obtained by Cardan's rule; but if two roots of the biquadratic be possible and two roots impossible, two values of n will be impossible, and the cubic may be solved, and consequently the roots of the proposed equation may be found.

THE METHOD OF DIVISORS.

(336.) Since the last term of an equation is the product of all the roots with their signs changed, if any root be a whole number, it may be found amongst the divisors of the last term.

Ex. Suppose x³ - 4x² - 6x + 12 = 0; the divisors of the last term are 1, - 1, 2, - 2, 3, -3, 4, - 4, 6, - 6, 12, -12, and by substituting these successively for x, we find that - 2 is a root of the equation.

(337.) When the last term admits of many divisors, the number of trials may be lessened by finding the limits between which the roots of the equation lie; or by increasing, or diminishing, the roots of the equa-tion, and thus lessening the number of divisors of the last term.

(338.) The number of trials may also be lessened by substituting three or more terms of the arithmetical progression1, 0, -1, &c. for the unknown quantity, and farming the divisors of the results, taken in order, into arithmetical progressions, in which the common difference is unity; as it will only be necessary to try those divisors of the last term of the equation which are found in these progressions,

[page] 179

Let 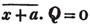be the equation, one factor of which is x+a, and Q the product of the rest; if 1, 0, - 1, be successively substituted for x, the results are respectively divisible by a + 1, a, and a -1; therefore amongst the divisors of the results, formed into arithmetical progressions in which the common difference is unity, is found the decreasing progression a + 1, a, a -1 j and if all the terms corresponding to a, with their signs changed, be substituted in the equation for x, the integral values of x will be discovered.

Ex. Let the proposed equation be x³ - 4x² - 6x + 2 = 0.

SUPP.	Results	Divisors	Progr.
X = 1	3	1, 3	3
X = 0	12	1, 2, 3, 4, 6, 12	2
X = -1	13	1, 13	1

The only decreasing progression that can be formed out of the divisors is 3, 2, 1, therefore if one root of the equation be a whole number, - 2 is that root; and on trial it is found to succeed.

(339.) If the highest power of the unknown quantity be affected with a coefficient, let 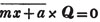be the equation, and substitute 1, 0, - 1, successively for x, then a + m, a, and a - m are divisors of the results, if the equation have a factor of the form mx + a, or a root - a/m. Also m, the common difference, in the arithmetical progression a + m, a, a - m, is a divisor of the coefficient of the first term of the

M 2

[page] 180

equation. In this case therefore, all the decreasing progressions must be taken out of the divisors of the resulting quantities, in which the common difference is unity, or some divisor of the coefficient of the highest term of the equation, and amongst them is the progression a + m, a, a - m; therefore by making trial, successively, of the terms corresponding to a in the progressions thus obtained, the factor mx + a, which divides the equation without remainder, will be found.

Ex. To find a divisor of the equation 8x³ - 26x² + 11x + 10 = 0, if it admit one of the form mx + a.

SUPP.	Res	Divisors	Progress.
X = 1	3	1, 3, -1, - 3	3, 3, - 3
X = 0	10	1, 2, 5, 10, - 1, - 2, - 5, - 10	1, 2, - 5
X = - 1	- 35	1, 5, 7, 35, -1, -5, -7, -35	-1, 1, -7

The decreasing progressions, in which the common difference is a divisor of 8, formed out of the divisors, are 3, 1, -1; 3, 2, 1; and - 3, - 5, -7; therefore the factors to be tried are 2x + 1, x + 2, and 2x - 5, the last of which succeeds, and consequently 2x - 5, = 0 (Art. 268), or x = 5/2.

(340.) If an equation be of four, or more dimensions, though it has no divisor of the form mx + a, it may have one of the form ± mx² ± nx ± r.

To find when this is the case, let 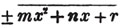×Q = 0 be the equation; and for x substitute successively, p + e, p, p - e, &c. then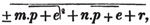 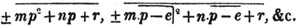are divi-

[page] 181

sors of the resulting quantities; and if they be respectively subtracted from, or added to, 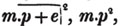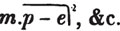the remainders, or sums, are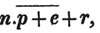 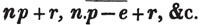which form a decreasing arithmetical progression whose common difference is ne. When p = 0, and e = 1, this progression becomes n + r, r, - n + r &c. and in all cases m is a divisor of the first term of the equation. Let therefore 1, 0, - 1, - 2, &c. be substituted for x in the proposed equation, and let the differences and sums of the divisors of the results, and m, 0, m, 4m, &c. be taken; then if all the arithmetical progressions possible be formed out of these quantities, in order, amongst them will be found the progression n + r, r, - n + r, &c. therefore, by trial, the divisor mx² + nx + r will be discovered, if the equation admit of a quadratic divisor whose coefficients are whole numbers.

Let the proposed equation be 3x⁴ + 4x³ +3x² - 2x + 2 = 0:

[page] 182

SUPP.	Res	Divisors	Sq.	Sum and Differences.	Progress.
X = 1	10	1, 2, 5, 10	3	- 7, - 2, 1, 2, 4, 5, 8, 13	- 2, 1
X = 0	2	1, 2	0	- 2,- 1, 1, 2	2, - 1
X = - 1	6	1, 2, 3, 6	3	- 3, 0, 1, 2, 4, 5, 6, 9	6, - 3
X = - 2	34	1, 2, 17, 34	12	- 22,- 5, 10, 11, 13, 14, 29, 46	10, - 5

From the first progression, n = - 4, r = 2; from the other, n = 2, and r = -1; therefore, since m may either be positive or negative, the divisors to be tried

[page] 183

are ± 3x² - 4x + 2, and ± 3x² + 2x - 1; of which, -3x² + 2x - 1, or 3x² - 2x + 1 succeeds; consequently, the roots of the equation 3x² - 2x + 1 = 0, are two roots of the proposed biquadratic.

THE METHOD OF APPROXIMATION.

(341.) The most useful and general method of discovering the possible roots of numeral equations, is approximation. Find by trial (Art. 318), two numbers, which substituted for the unknown quantity give, one a positive, and the other a negative result; and an odd number of roots lies between these two quantities, that is, one possible root at least, lies between them; then by increasing one of the limits, and diminishing the other, an approximation may be made to the root; substitute this approximate value, increased or diminished by v, for the unknown quantity in the equation, neglect all the powers of v above the first, as being small when compared with the other terms, and a simple equation is obtained for determining v, nearly; thus a nearer approximation is made to the root, and by repeating the operation, the approximation may be made to any required degree of exactness.

Ex. Let the roots of the equation y³ - 3y +1 = 0. be required.

When 1 is substituted for x the result is -1, and when 2 is substituted, the result is + 3, therefore one possible root lies between 1 and 2; try 1.5; and the result is -.125, or the root lies between 1.5 and 2.

[page] 184

Let 1.5 + v = y; then,

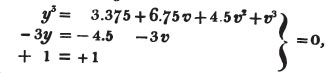
that is, -.125 + 3.75v + 4.5v² + v³ = 0, and neglecting the two last terms, -.125 + 3.75v = 0, or v = .125/3.75 = .033 nearly, and y = 1.5 + v = 1.533. Again, suppose 1.533 + v - y; by proceedingas before, we find .003686437 + 4.050267v = 0, and v = -.003686437/4.050267 = -.0009101 &c. hence, y = 1.532089 nearly. The other roots may be found by the solution of a quadratic (Art. 267).

(342.) The accuracy of the approximation does not depend upon the ratio which the quantity assumed bears to the root, but upon it's being nearer to one root than to any other.

Let the roots of the equation xⁿ - px^{n -1} + qx^{n - 2} - &c = 0, be a + m, a + n, a + r, &c. of which a + m is the least; assume a + v - x, and let P - Qv + Rv² - Sv³ + &c. = 0, be the transformed equation, whose roots are m, n, r, &c. then q/p = 1/m + 1/n + 1/r + &c. (Art. 273), and 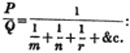In the process we assume P 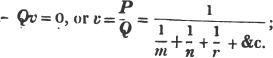and on suppo-

[page] 185

sition that m is much less than n or r, &c. 1/m is much greater than 1/n + 1/r + &c. and P/Q = m nearly; but if m bear a finite ratio to n or r, the approximation will be less accurate, and the less these magnitudes n, r &c. are, the greater error is made in supposing 1/n + 1/r + &c. to vanish, when compared with 1/m.

(343.) When m and n are nearly equal to each other, and much less than r, s, &c. and also both positive or both negative, then 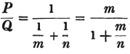, nearly, which is an approximation to m the less of the two; but if one of these quantities be positive and the other negative, 1/m + 1/n may be either positive or negative, and greater, equal to, or less than 1/r + 1/s + &c. and consequently P/Q is not necessarily an approximation to any of the quantities m, n, r, r, &c.

Let P - Qv + Rv² = 0; the roots of this equation will be m and n, nearly. For if m, n, r, s, be the roots of the equation P- Qv+Rv² - Sv³ + &c. = 0, P = mnrs, Q = mns + mnr + mrs + nrs, R = mn + mr + ms + nr + ns + rs, and since m and n are small when compared with r and s, Q = mrs + nrs nearly, and R = rs nearly; therefore the equation P - Qv + Rv² = 0 becomes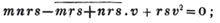

[page] 186

hence, 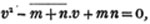whose roots are m and n. By the solution then of this quadratic, a much nearer approximation is made to the root a + m than by the former method, and at the same time, an approximation is also made to the root a + n.

(344.) In the same manner, if t roots be nearly equal, in order to approximate to them, it will be necessary to solve an equation of t dimensions. See Dr. Waring's Med. Algeb. p. 186.

(345). If we have two equations, containing two unknown quantities, we may discover the values of these quantities nearly in the same manner.

Ex.

Let 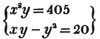To find x and y.

Find, by trial, approximate values of x and y; such are 20 and 1; and let x = 20 + v, y = 1 + z;

then x²y = 400 + 40v + 400 z + v² + 40vz + v²z = 405,

and xy - y² = 19 + v + 18z + vz - z² = 20,

and neglecting those terms in which z or v is of more than one dimension, or in which their product is found, as being small when compared with the rest,

400 + 400v + 400 z = 405

19 + v +18z = 20

40 v + 400z = 5

v + 10z = .125

and v + 18z + 19 = 20

or v + 18z = 1

hence, 8 z = .875

z = .109375

[page] 187

v = .125 - 10z = - .96875

therefore x = 19.03

and y = 1.109.

By making use of the values thus obtained, nearer approximations may be made to x and y.

ON THE REVERSION OF SERIES.

(346.) If two quantities Ax + Bx² + Cx³ + &c. and ax + bx² + cx³ + &c. be always equal, the invariable coefficients of the corresponding terms are equal.

For if these equal quantities be divided by x, we have A + Bx + Cx² + &c. = a + bx + cx² + &c. or when ^x vanishes, A = a, and A and a are invariable, therefore in all cases, A = a; hence also, bx + Cx² + &c. = bx + cx² + &c. or dividing by x, B + Cx + &c. = b + cx + &c. and when x vanishes, B = b, therefore in all cases, B = b. In the same manner, C = c, &c.

(347.) COR. If SA + Bx + Cx² + &c. = 0 in all cases, then A = 0, B = 0, C - 0, &c.

(348.) Approximation may be made to a root of an equation, by assuming for it a series, involving the powers of that quantity in terms of which it is sought, with indeterminate coefficients; this series being substituted for the unknown quantity in the proposed equation, the coefficients may be found by making each term equal to 0, and thus the series, which expresses the value of the unknown quantity, may be determined.

[page] 188

Ex. Let y³ - 3y + x = 0; required the value of y in terms of x.

Lety = ax + bx³ + cx⁵ + dx⁷ + &c. then

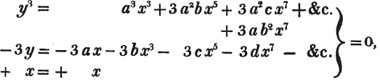
and supposing each term to vanish (Art. 347), - 3a + 1 = 0, or a = 1/3; a³ - 3b = 0, b = a³/3 = 1/3⁴; 3a²b - 3c = 0, and c = a² b = 1/3⁶; &c. therefore

y = x/3 + x³/3⁴+x⁵/3⁶+c. and when x = 1, y = 1/3 + 1/3⁴ + 1/3⁶ + &c. = .347 &c. which is one root of the equation y³ - 3y + 1 = 0.

If for y, the series ax + bx² + cx³ + dx⁴ + &c. had been assumed, the quantities b, d, &c. would have been found = 0; therefore the even terms are unnecessary.

(349.) Cor. The less x is assumed, the faster will this series converge, and the more accurately will y be obtained.

(350.) This method of approximation is similar to the former, in this respect, that the series will have a slow degree of convergency, unless one value of y be much less than any other. If this be not the case, find m, an approximate value of y, by trial, and assume m ± v = y; then, when one value of v is much less than any other, it may be found by this reversion,

[page] 189

and consequently that value of y which is nearest to m, will be known.

In the example Art. 341, y being found nearly 1.5, assume v + 1.5 = y, and the equation is transformed into v³ + 4.5v² + 3.75v - .125 = 0: call this v³ + pv² + qv - x = 0, and take v = ax + bx² + cx³ + dx⁴ + &c. then

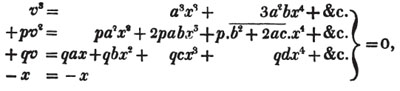
hence, qa -1 = 0, and a = 1/q = 1/3.75 = .26666 &c. qb+ pa² = 0, and b = -pa²/q = -.08533 &c. therefore v = 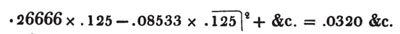and y = v + 1.5 = 1.5320 &c. as before.

(351.) The same method may be used to find y in terms of x, when x and y, and their powers, are combined in any manner in the equation.

Ex. 1. Let x = ay + by² + cy³ + &c. required the value of ^y in terms of ^x.

Assume y = Ax + Bx² + Cx³ + &c.

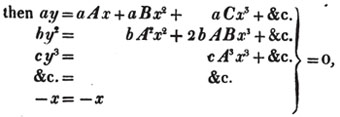
therefore a A -1 = 0, or A = 1/a; a B + b A² = 0, or

[page] 190

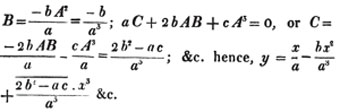
Ex. 2. Let x = y - ay³ + by⁵ - &c. required the value of y in terms of x.

Assume y = Ax + Bx³ + Cx⁵ + &c.

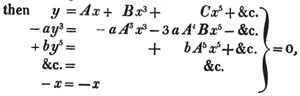
hence, A - 1 = 0, or A = 1; B - a A³ = 0, or B = a; C - 3a A²B + bA⁵ = 0, or 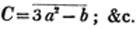therefore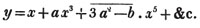

The method of determining the proper series to be assumed in each case, without previous trial, is given by Maclaurin; Alg. Pt. 2. Ch. 10.

ON THE SUMS OF THE POWERS OF THE ROOTS OF AN EQUATION.

(352). Let a, b, c, &c. be the roots of the equation xⁿ - px^{n - 1} + qx^{n - 2} ….. + wx^{n - m} - &c. = 0, and A B, C, ……… P, Q; R, S, the sum of the roots, sum of their squares, cubes, ……… m - 3, m -2, m -1, m, powers respectively; then will A = p, B = p A - 2q, C = p B - qA + 3r, &c. and S = pR- qQ + rP ….

[page] 191

-mw; where + w is the coefficient of the 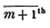term.

It appears by Art. 308 that

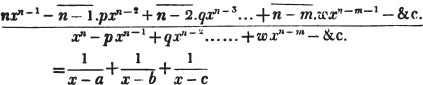whatever be the value of x; and by actual division,

1/x-a = 1/x + a/x² + a²/x³ …. + a^m/x^m+1 + &c.

1/x-b = 1/x + b/x² + b²/x³ …. + b^m/x^m+1 + &c.

1/x-c = 1/x + c/x² + c²/x³ …. + c^m/x^m+1 + &c. &c.

and if x be supposed greater than any of the magnitudes a, b, c, &c. no quantity is lost in the division; therefore by addition,

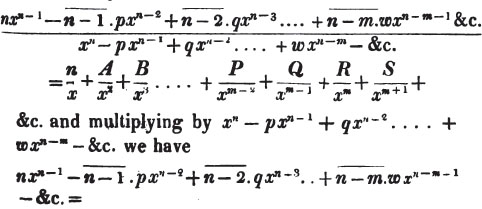
[page] 192

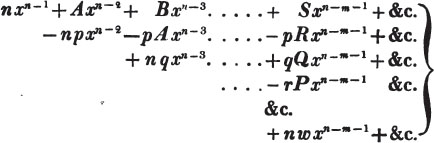
and by equating the coefficients,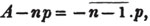

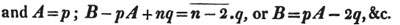
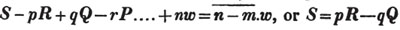
Ex. Let x³ + 5x² - 6x - 8 = 0; then by comparing the terms of this, with the terms of the equation xⁿ - px^{n - 1} + qx^{n - 2}&c. = 0, we have p = -5, q = - 6, r = 8.

Hence, the sum of the roots = p = -5 = A.

Sum of the squares pA- 2q = 25 + 12 = 37 = B.

Sum of the cubes = pB - qA + 3r = - 185 - 30 + 24 = - 191 = C &c.

(353.) The proposition also admits of the following proof.

The same notation being retained; let m and n be equal, and since a, b, c, &c. are roots of the equation,

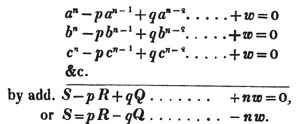
If m be greater than n; multiply the proposed equation by x^m-n, then x^m - px^{m - 1} + qx^{m - 2} + wx^{m - n}

[page] 193

= 0; which equation has the roots a, b, c, &c. and m - n roots each equal to 0; therefore the sum of the m^th powers of the roots of this equation.is equal to the sum of the m^th powers of the roots of the former; that is, S = pR - qQ + &c. to n terms.

When m is less than n: The sum of the m^th powers of the roots may be expressed in terms of p, q, r, …… w, where w is the coefficient of the m + 1^th term of the equation. For p² contains a² + b² + c² + &c. with other combinations of the roots, as ab, ac, bc, &c. which combinations are contained in a multiple of q; also, p³ contains a³ + b³ + c³ + &c., with other combinations, such as a²b, a²c, b²a, &c. abc, acd, bcd, &c. and these combinations may be made up of p, q and r; for p x q contains the quantities a²b, a²c, b²a, &c. and r is the sum of the quantities abc, acd, bcd, &c. In the same manner it appears, that a⁴ + b⁴ + c⁴ + &c. may be found in terms of p, q, r and s; and in general, a^m + b^m + c^m + &c. may be expressed in terms of p, q, r, …..w. Also, the number of combinations of any particular form, as a²b, cannot be altered by the introduction of the root c; consequently the numeral coefficient of the product pq, by which the combinations of that form are taken away, is the same, whatever be the number of roots: Hence the expression for am + bm + cm &c. in the equation xⁿ - px^{n - 1} + qx^{n - 2} ….. + wx^{n - m} - &c. = 0, is the same with the expression for. the sum of the m^th powers of the roots of the equation x^m - px^{m -1} + qx^{m - 2}.....+ w = 0; that is, S = pR - qQ.... -mw.

N

[page] 194

This rule was given by Sir I. Newton for the purpose of approximating to the greatest root of an equation. Suppose the roots all possible, and one greater than the rest, the powers of this root increase in a higher ratio than those of any other, and the 2m^th power of this root will approach nearer and nearer to a ratio of equality with the sum of the 2m^th powers of the roots, as m increases; therefore by extracting the 2m^th root of this sum, an approximation is made to the greatest positive or least negative root (See Art. 306).

ON THE IMPOSSIBLE ROOTS OF AN EQUATION.

(354.) It has before been shewn (Art. 311), that there are as many positive roots in an equation as it has changes of signs, and as many negative roots as continuations of the same sign, when the roots are all possible.

But this rule cannot be applied to impossible roots; which appears by the demonstration there given, as well as from the consideration, that an. impossible expression cannot be said to be either positive or negative.

If then it appear from the terms of an equation that some roots may, according to the above rule, either be called positive or negative, they must be impossible. Thus, two roots of the equation x³ + qx + r = 0, or x³ ± o + qx + r = 0, are impossible; be

[page] 195

cause it has two changes of signs or none, according as the Second term is supposed to be - o, or + o. In the same manner, if any term of an equation be wanting, and the sign of the adjacent terms be both positive or both negative, the equation has, at least, two impossible roots: and if two succeeding terms be wanting, it must always have, at least, two impossible roots.

(355.) Impossible roots enter equations by pairs (Art. 277); they also Ke under the form of two positive or two negative roots.

Let ± a + √-b² and ± a - √-b² be the roots; then 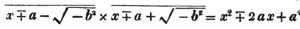+ b² = 0, the signs of which equation shew that it has either two positive, or two negative roots.

(356.) Con. Hence, if the last term of an equation of an even number of dimensions be negative, it will have at least two possible roots, one positive and the other negative (Art. 271).

(357.) Let an equation be transformed into one whose roots are the squares of the roots of the former, (Art. 296), then as many negative roots as the transformed equation contains, so many impossible roots, at least, are in the original equation, because the square of a possible quantity is always positive.

(358.) If any series of magnitudes be substituted in order, for the unknown quantity in an equation, there can only be as many changes of signs in the results, as the equation contains possible roots.

N 2

[page] 196

Let 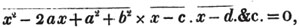be an equation whose roots are a +√-b², a √-b² c, d, &c. whatever magnitude is substituted for x, the quantity x² - 2ax + a² + b², which is the sum of two squares, 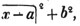is positive; therefore the changes of signs can only arise from the substitution of quantities for x in the product 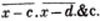which changes, when the quantities are taken in order, are as many as there are positive roots c, d, &c. (See Art. 298.)

(359.) The limiting equation* has, at least, as many possible roots as the original equation, wanting one.

Let 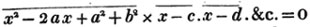be the proposed equation, the limiting equation is

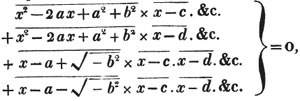
or by adding the two last terms together, it is

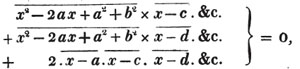
(See Art. 307), in which, if c, d, &c. be successively substituted for x, the results are +, -, &c. therefore there are possible roots in this latter equation which lie between c, d, &c. or this equation contains at least, as many possible roots, wanting one, as the original equation. It may contain more.

* This is the limiting equation mentioned Art. 307; the proposition is not necessarily true of the other limiting equations, Art. 314.)

[page] 197

(360.) COR. 1. Hence it follows, that there are; at least, as many impossible roots in the original equation as in the equation of limits. There may be more; therefore from the number of impossible roots in the limiting equation, we cannot determine, exactly, the number in the original equation.

(361.) COR. 2. Hence also it appears, that if the possible roots of the limiting equation be substituted successively in the original equation, we know from the signs of the results, what possible roots the latter contains. For, roots of the limiting equation lie between the possible roots of the proposed equation (Art. 359.)

Ex. Let x^{n + 3} - a^{n + 1}x² + p²d^{n + 1} = 0.

Its limiting equation is 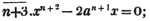whose possible roots, when n is an odd number, are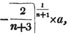 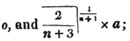which substituted in the original equation give the results either -, +, -, or +, +, +; therefore it has either four possible roots or none.When n is even, the possible roots of the limiting equation are 0 and 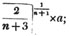therefore the equation itself will have one possible root or three, according as 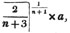when substituted for x, gives a positive or a negative result.

(362.) The roots of a quadratic equation are impossible, if the square of the middle term be less than four times the product of the extremes.

Let ax²+ bx + c = 0; then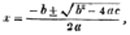

[page] 198

Which expression becomes impossible wheft b² is less than 4ac.

(363.) It appears from Art. 360, that there are impossible roots in an equation, whenever there are impossible roots in its limiting equation. In the same manner, if the next limit be taken, there are impossible roots in the original equation, whenever there are impossible roots in this limit; and if the limit be thus brought down to a quadratic, when the roots of the quadratic are impossible, there are impossible roots in the original equation corresponding to them. On this principle is founded Sir I. Newton's rule for discovering impossible roots in any equation.

Let the proposed equation be xⁿ - px^{n - 1}. … + Dx^{n - r + 1} - Ex^{n - r} + Fx^{n - r - 1} - &c. = 0. To obtain a limiting equation, which shall be a quadratic, corresponding to the terms Dx^{n - r + 1} - Ex^{n - r} + Fx^{n - r - 1} let the succeeding terms be taken away, by multiplying by the terms of the arithmetical progressions n, n -1, n - 2, …., 1, 0; n - 1, n - 2, …. 2, 1, 0; n - 2, n - 3, …. 2, 1, 0; &c. and let the preceding term be taken away, by multiplying by the terms of the progressions, 0, 1, 2, …. r + 1; 0, 1, 2, …. r; &c. as follows;

[page] 199

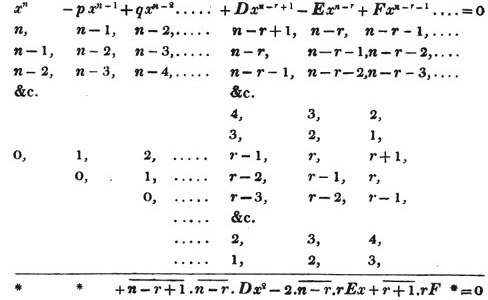
The respective products being taken, and those quantities left out which are found in every product, we obtain a limiting quadratic 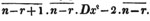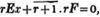corresponding to the three terms Dx^{n - r +1} - Ex^{n - r} + Fx^{n - r - 1} and if two roots of this quadratic be impossible, that is, 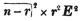be

[page] 200

less than 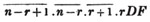(Art. 362), or 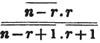E² less than DF, there are impossible roots in the proposed equation, corresponding to them*.

Write down therefore a series of fractions 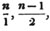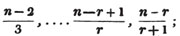divide each fraction by that which precedes it, and place the results,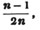 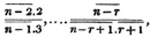over the succeeding terms of the equation, beginning with the second; also, place the sign + under the first and last terms, and under every other term + or -, according as the square of that term multiplied by the fraction which stands over it, is greater or less than the product of the adjacent terms; then there will be as many impossible roots in the equation as there are changes of these signs from + to - and from - to +.

Ex. Let the proposed equation be x³ - 4x² + 4x - 6 = 0.

In the series of fractions 3/1, 2/2, 1/3, if each term be divided by that which precedes it, we obtain 1/3, 1/3 to be placed over the terms of the equation;

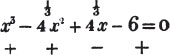
* See Art. 315.

[page] 201

and since 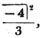or 16/3, is greater than 4×1, the sign + must be placed under the second term; but 4², or 16/3, is less than - 4×-6, or 24, therefore the sign - must be placed under the third term; and + being placed under the first and last terms, there are two changes of signs; therefore the equation contains two impossible roots.

SOCHOLIUM

(364.) The discovery of the number of impossible roots in an equation has given great trouble to Algebraists, and their researches, hitherto, have not been attended with any great success. In a cubic equation x³ - qx + r = 0 two roots are impossible or not, according as r²/4 - q³/27 is positive or negative (Art. 331). A biquadratic, x⁴ + qx² + rx + s = 0, has two impossible roots, when two roots of the equation 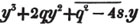- r² = 0, are impossible; and all it's roots are impossible, when the roots of this cubic are all possible and two of them negative (Art. 333).

(365.) Dr. Waring has given a rule for determining the number of impossible roots in an equation of five dimensions, but the investigation cannot properly be introduced into an elementary treatise. See Med. Algebraicœ, p. 82.

[page] 202

(366.) Sir I. Newton's rule (Art. 363) is general and easily applied, but as it is deduced from the nature of the inferior limits, it will not always detect impossible roots (Art. 360). The proof also is defective, as it does not extend to that part of the rale which respects the number of impossible roots. Thus far however it may be depended upon, that it never shews impossible roots, but when there are some such in the proposed equation.

Many other rules, which will frequently discover the impossible roots in any equation, may be seen in the Med. Alg. C. 2.

THE END OF PART II.

[page 203]

THE

ELEMENTS OF ALGEBRA.

PART III.

ON UNLIMITED PROBLEMS.

(367.) WHEN there are more unknown quantities than independent equations, the number of corresponding values which those quantities admit, is indefinite (Art. 145). This number may be lessened, by rejecting all the values which are not integers; it may be farther lessened, by rejecting all the negative values; and still farther, by rejecting all values which are not square, or cube numbers; &c. By restrictions of this kind, the number of answers may be confined within definite limits.

(368.) If a simple equation express the relation of two unknown quantities, and their corresponding integral values be required; divide the whole equation by the coefficient which is the less of the two, and suppose that part of the quotient, which is in a fractional form, equal to some whole number; thus a new

[page] 204

simple equation is obtained, with which we may proceed as before; let the operation be repeated, till the coefficient of one of the unknown quantities is unity, and the coefficient of the other a whole number; then an integral value of the former may be obtained by substituting 0, or any whole number for the other; and from the preceding equations, integral values of the quantities proposed may be found.

Ex. 1.

Let 5x + 7y = 29; to find the corresponding integral values of x and y.

Dividing the whole equation by 5, the less coefficient,

x + y + 2y/5 = 5 + 4/5

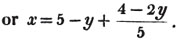
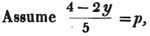or 4 - 2y = 5p

then 2 - y = 2p + p/2.

y = 2 - 2p - p/2

let p = 2s, then y = 2 - 5s, and x = 5 - y + p = 3 +5s + 2s = 3 + 7s.

If s = 0, then x = 3 and y = 2, the only positive whole numbers which answer the condition of the equation; for if s = 1, then x = 10, and y = - 3; and if s = - 1, then x = - 4, and y = 7.

[page] 205

Ex.2.

To find a number which being divided by 3, 4, 5, the remainders are 2, 3, 4, respectively.

Let x be the number,

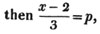a whole number,

or x = 3p + 2; also, from the second condition,

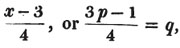a whole number,

that is, 3p - 1 = 4q

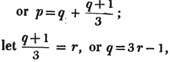
then p = 4r - 1, and x = 3p + 2 = 12r - 1;

again, from the third condition, 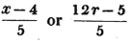is a whole number, that is, 2r + 2r/5 - 1 is a whole number, therefore 2r/5 is a whole number; let 2r/5 = 2m; then r = 5 m, and x = 12r - 1 = 60m - 1; if m = 1, x = 59; if m = 2, x = 119; &c.

(369.) If the simple equation contain more unknown quantities, their corresponding integral values may be found in the same manner.

Ex. 3.

Let 4x + 3y + 10 = 5v; to find corresponding integral values of x, y and v.

[page] 206

Dividing the whole equation by 3, the least coefficient,

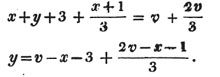
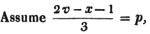or 2v - x - 1 = 3p,

then x = 2v - 3p - 1

and y = v - 2v + 3p + 1 - 3 + p = 4p - v - 2,

and substituting for p and v, nothing, or any whole numbers, integral values of x and y are obtained: If v = 3, and p = 1, then x = 2 and y = 1; if v = 4, and p = 0, then x = 7 and y = - 6; &c.

(370.) In the solution of different kinds of unlimited problems, different expedients must be made use of, which expedients, and their application, are chiefly to be learned by practice.

Ex. 1.

To find what numbers are divisible by 3 without remainders.

Let a, b, c, d, &c. be the digits, or figures in the unit's, ten's, hundred's, thousand's, &c. place of any number, then the number is a + 10b + 100c + 1000d + &c. this divided by 3 is a/3 + 3b + b/3 + 33c + c/3 + 333d + d/3 + &c. or 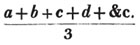+ 3b + 33c + 333d + d/3 + &c. which is a whole number when 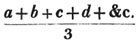is a whole number; that is, any

[page] 207

number is a multiple of 3 if the sum of it's digits be a multiple of 3. Thus 111, 252, 785l, &c are multiples of 3.

In the same manner, any number is a multiple of 9 if the sum of it's digits be a multiple of 9.

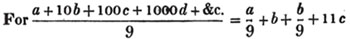
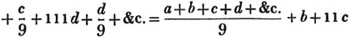+ 111d + &c. which is a whole number when 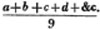is a whole number. Thus 684, 6588, &c. are multiples of 9.

COR. 1. Hence, if any number, and the sum of it's digits, be respectively divided by 9, the remainders are equal.

COR. 2. From this property of 9 may be deduced a rule which will sometimes detect an error in the multiplication of two numbers.

and if the sum of the digits in the multiplicand be divided by 9, the remainder is x; if the sum of the digits in the multiplier be divided by 9, the remainder is y; and if the sum of the digits in the product be divided by 9, the remainder is the same as when the sum of the digits in xy is divided by 9, if there be no mistake in the operation.

[page] 208

Ex. 2.

To find a perfect number, that is, one which is equal to the sum of all the numbers which divide it without remainder.

Suppose yⁿx to be a perfect number; it's divisors are 1, y, y² …. yⁿ, x, xy, xy² …. xy^{n - 1}; therefore yⁿx = 1 + y + y². … + yⁿ + x+ xy + xy² …. + xy^{n - 1}.

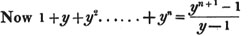
and 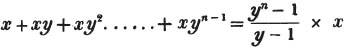(Art. 222);

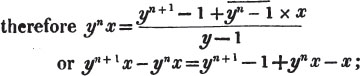
hence, 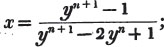and that x may be a whole number, let y^{n + 1} - 2yⁿ = 0, or y - 2 = 0, that is, y = 2; then x = 2^{n + 1}- 1. Also, let n be so assumed that 2^{n + 1} -1 may have no divisor but unity, which was supposed in taking the divisors of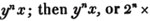 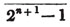is a perfect number. Thus, if n = 1, the number is 2×3 or 6, which is equal to 1 + 2 + 3 the sum of it's divisors: If n = 2, the number is 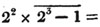4×7 = 28.

Ex. 3.

To find two square numbers, whose sum is a square

Let x² and y² be the two squares;

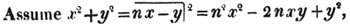
[page] 209

then x² = n²x² - 2nxy

x = n²x - 2ny

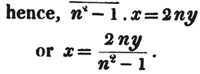
And if n and y be assumed at pleasure, such a value of x is obtained, that x² + y² is a square number.

But if it be required to find integers of this description, let y = n² - 1, then x = 2n, and n being taken at pleasure, integral values of x and y, and consequently of x² and y², will be found. Thus, if n = 2, then y = 3 and x = 4, and the two squares are 9 and 16, whose sum is 25.

Ex. 4.

To find two square numbers, whose difference is a square.

Let x² and y² be the two squares;

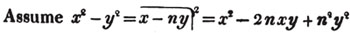
then -y² = - 2nxy + n²y²

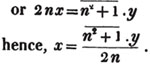
And if y = 2n, then x = n² + 1. Thus, if n = 2, then y = 4, and x = 5; hence, x² - y² = 25 - 16 = 9*.

ON CONTINUED FRACTIONS.

(371.) To represent a/b in a continued fraction.

* On this subject, see the Edinburgh Transactions, Vol. II. p. 198.

O

[page] 210

Let b be contained in a, p times, with a remainder c; again, let c be contained in b, q times, with a remainder d, and so on; then we have

a = pb + c

b = qc + d

c = rd + e

&c.

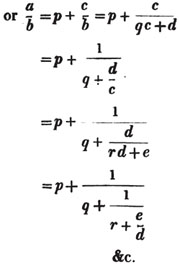
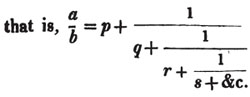
(372.) COR. 1. An approximation may thus be made to the value of a fraction whose numerator and denominator are in too high terms, and the farther the division is continued, the nearer will the approximation be to the true value.

[page] 211

(373.) COR. 2. This approximation is alternately less and greater than the true value. Thus p is less than a/b; and p + 1/q is greater, because a part of the denominator of the fraction is omitted: q + 1/r is too great for the denominator, therefore 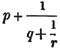is less than a/b; &c;

Ex. To find a fraction which shall be nearly equal to 314159/100000, and in lower terms.

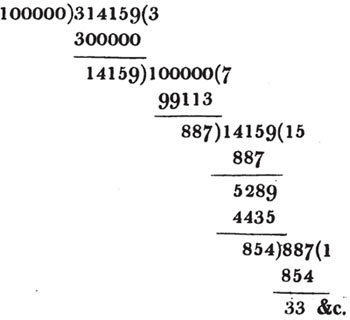
Here, p = 3, q = 7, r = 15, s = 1, &c. therefore 314159/100000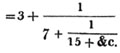

O 2

[page] 212

The first approximation is 3, which is too little; the next is 3 + 3/7 = 22/7, too great; the next is 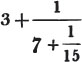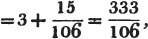too little; and so on. This fraction expresses, nearly, the circumference of a circle whose diameter is unity; therefore the circumference is greater than 3 diameters, less than 22/7 diameters, and greater than 333/106, &c.

(374.) To find the value of a continued fraction, when the denominators q, r, s, &c. recur in any certain order.

Ex. 1.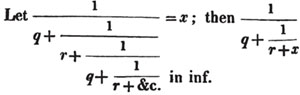

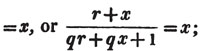hence r + x = qrx + qx² + x, and x² + rx - r/q = 0, from the solution of which quadratic the value of x may be obtained.

Ex. 2. 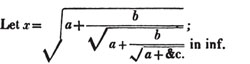by squar-

[page] 213

ing both sides, 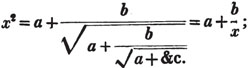and x³ - ax - b = 0; whence the value of x may be found.

(375.) In the same manner may the values of other quantities, which run on in infinitum, be found, if the factors recur.

Ex. 1. To find the value of in infinitum.

Let 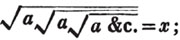by squaring both sides; 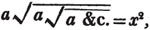that is, ax = x², or x = a.

Ex. 2. Required the value of 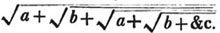in infinitum.

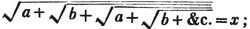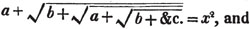
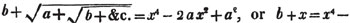
Let by squaring both sides, 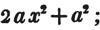= x⁴ - 2ax² + a², or b + x = x² - 2ax² + a²; hence, x² - 2ax² - x + a² - b = 0; from which equation x may be found.

(376.) To find the value of a fraction when the numerator and denominator are evanescent.

Since the value of a fraction depends, not upon the absolute, but the relative magnitude of the numerator and denominator, if in their evanescent state they have a finite ratio, the value of the fraction will be finite. To determine this value, substitute for

[page] 214

the variable quantity, it's magnitude, when the numerator and denominator vanish, increased by another variable quantity; then suppose this latter to decrease without limit, and the value of the proposed fraction will be known.

Ex. 1. Required the value of 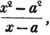x = a.

Let x = a + v, and the fraction becomes 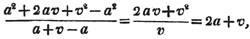and when v = 0, or x = a, the value is 2a.

Ex. 2. Required the value of 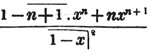when x = 1.

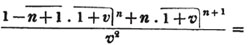
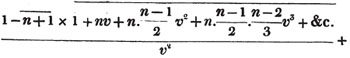
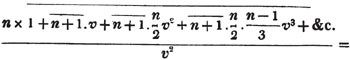
Let x = 1 + v, and the fraction becomes 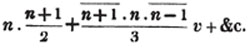and when v = 0, or x = 1, the fraction becomes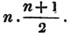

(377.) To find the least common multiple of two quantities; or the least quantity which is divisible by each of them without remainder.

[page] 215

The product of the two quantities divided by their greatest common measure, is their least common multiple.

Let a and b be the two quantities, x their greatest common measure, m their least common multiple, and let m contain a, p times, and b, q times; that is, let m = pa = qb; then a/b = q/p; and since m is the least possible, p and q are the least possible; therefore q/p is the fraction in it's lowest terms, and consequently q = a/x; hence, m = qb = ab/x.

Ex. What is the least common multiple of 18 and 12?

Their greatest common measure is 6; therefore their least common multiple is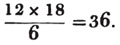

(378.) Every other common multiple of a and b is a multiple of m.

Let n be any other common multiple of the two quantities; and, if possible, let m be contained in n, r times, with a remainder 8, which is less than m; then n - rm = s; and since a and b measure n and rm, they measure n - rm, or s (Art. 91); that is, they have a common multiple less than m, which is contrary to the supposition.

(379.) To find, the least common multiple of three quantities a, b and c, take m the least common mul-

[page] 216

tiple of a and b, and n the least common multiple of m and c; then n is the least common multiple sought.

For every common multiple of a and b is a multiple of m (Art. 378); therefore every common multiple of a, b and c is a multiple of m and c; also, every multiple of m and c is a multiple of a, b and c; consequently the least common multiple of m and c is the least common multiple of a, b and c.

(380.) Three quantities are said to be in harmonical proportion, when the first is to the third, as the difference of the first and second is to the difference of the second and third.

Any magnitudes A, B, C, D, E, &c. are said to be in harmonical progression, if A : C :: A - B : B - C; B : D :: B - C : C - D; C : E :: C - D : D - E; &c.

(381.) The reciprocals of quantities in harmonical progression are in arithmetical progression.

Let A, B, C, &c. be in harmonical progression; then A : C:: A - B : B - C; therefore AB - AC = AC - BC, and dividing both sides by ABC, 1/C - 1/B = 1/B - 1/A.

Again, B : D :: B - C: C - D; therefore BC - BD = DB - DC, and dividing by BCD, 1/D - 1/C = 1/C - 1/B; and 1/C - 1/B has been proved equal to 1/B - 1/A; therefore the quantities 1/A, 1/B, 1/C, 1/D, &c. have a common difference, that is, they are in arithmetical progression.

[page] 217

(382.) Required the cube root of the binomial a + √- b².

Assume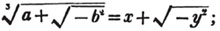

then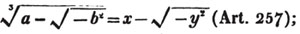

by mult. 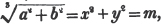by substitution; therefore y² = m - x²; also from the equation, = x³ + 3x² √ - y² = a; or substituting for y² it's value m - x², x³ = a, that is, 4x³ - 3mx - a = 0, a cubic equation, whose roots, which are all possible, may be found by approximation, or by a method which will be given in a following part of the work (Art. 515); hence, y, and consequently x + √- y², the root required, may be determined.

In the same manner it appears, that the c^th root may be extracted by the solution of an equation of c dimensions.

ON LOGARITHMS.

(383.) If there be a series of magnitudes a⁰, a¹, a², a³, … a^x; a^-1, a^-2, a^-3, …. a^-y, the indices, 0, 1, 2, 3, … x; -1, -2, -3, …. -y, are called the measures of the ratios of those magnitudes to 1, or the logarithms of the magnitudes, for the reason assigned Art. 165. Thus, x, the logarithm of any number c, is such a quantity, that a^x = c.

Here a may be assumed at pleasure; and for every different value so assumed, a different system of loga-

[page] 218

rithms will be formed. In the common tabular logarithms, a is 10, and consequently 0, 1, 2, 3, …. x, are the logarithms of 1, 10, 100, 1000, ….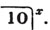

(384.) COR. 1. Since the tabular logarithm of 10 is 1, the logarithm of a number between 1 and 10 is less than 1; and in the same manner, the logarithm of a number between 10 and 100, is between 1 and 2; of a number between 100 and 1000, is between 2 and 3; &c.

These logarithms are also real quantities, to which approximation, sufficiently accurate for all practical purposes, may be made.

Thus, if x be the logarithm of 5, then 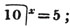let 2/3 be substituted for x, and 10^2/3 is found to be less than 5, therefore 2/3 is less than the logarithm of 5: but 10^3/4 is greater than 5, or 3/4 is greater than the logarithm of 5; thus it appears that there is a value of x between 2/3 and 3/4, such that 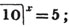the value set down in the tables is .69897, and 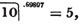nearly.

(385.) COR. 2. If quantities be in geometrical progression, a^x, a^2x, a^3x, &c. their logarithms, x, 2x, 3x, &c. are in arithmetical progression.

The method of finding the logarithms of the natural numbers, or forming a table, is explained in the Doctrine of Fluxions.

(386.) The sum of the logarithms of two numbers is the logarithm of their product; and the difference of the logarithms is the logarithm of their quotient.

[page] 219

Let x = log. of c, and y = log. of d; then a^x = c and a^y = d; hence, a^{x + y} = dc, and a ^{x - y} = c/d; or x + y is the log. of dc, and x - y the log. of c/d.

Ex. 1. Log. of 3×7 = log. of 3 + log. of 7.

Ex. 2. Log. of pqr = log. of pq + log. of r = log. of p + log. of q + log. of r.

Ex. 3. Log. of 5/7 = log. of 5 - log. of 7.

(387.) If the log. of a number be multiplied by n, the product is the log. of that number raised to the n^th power.

Let d be the number whose log. is x, or a^x = d; then a^nx = dⁿ; that is, nx is the log. of dⁿ.

Exs. Log. of 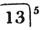= 5×log. 13. Log. b^z = z×log. b.

(388.) If the log. of a number be divided by n, the quotient is the log. of the n^th root of that number.

Let a^x = d, then a^x/n = d^1/n, or x/n is the log. of d^1/n.

Ex. Log. of 5^1/4 = 1/4×log. of 5.

(389.) The utility of a table of logarithms in arithmetical calculations will from hence be manifest; the multiplication and division of numbers being performed by the addition and subtraction of these artificial representatives; and the involution or evolution of numbers, by multiplying or dividing their logarithms by the indices of the powers or roots required.

[page] 220

Ex. Let the value of 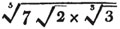the value required.

Log. of 7 = .845098

1/2 log. of 2 = .150515

1/3 log. of 3 = .1590404

5) 1.1546534 sum

.2309306 = log. of 1.70188 &c. the value required.

ON INTEREST AND ANNUITIES.

(390.) Interest is the consideration paid for the use or forbearance of the payment of money. The rate of interest is the consideration paid for the use of a certain sum for a certain time, as of £1. for one year.

When the interest of the principal alone, or sum lent, is taken, it is called simple interest; but if the interest, as soon as it becomes due, be considered as principal, and interest be charged upon the whole, it is called compound interest.

The amount is the whole sum due at the end of any time, interest and principal together.

Discount is the abatement made for the payment of money before it becomes due.

(391.) To find the amount of a given sum, in any time, at simple interest.

Let P be the principal,

r, the interest of one pound for one year,

[page] 221

n, the time for which the interest is to be calculated,

M, the amount.

Then since the interest of a given sum, at a given rate, must be proportional to the time, 1 (year) : n (years) :: r : nr the interest of £1. for n years; and the interest of P£, must be P times as great, or nrP; therefore the amount M = P + nrP.

(392). In this simple equation, any three of the quantities P, n, r, M being given, the fourth may be found; thus,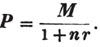

Ex. What sum must be paid down to receive £600, at the end of nine months, allowing 5 per cent, discount? Or, which is the same thing, what principal P will in nine months be equivalent, or amount to £600., allowing 5 per cent, interest?

In this case, M = 600, n = 3/4 = .75, r = .05; hence,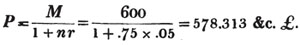

(393.) To find the amount of an annuity, or pension left unpaid any number of years, allowing simple interest upon each sum or pension from the time it becomes due.

Let A be the annuity; then at the end of the first year, A becomes due, and at the end of the second year, the interest of the first annuity is rA (Art. 391); at the end of this year, the principal becomes 2A, therefore the interest due at the end of the third year is

[page] 222

2rA; in the same manner, the interest due at the end of the fourth year is 3rA; &c. hence, the whole interest is 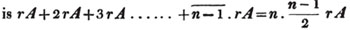(Art. 212); and the sum of the annuities is nA; therefore the whole amount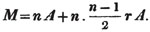

(394.) Required the present value of an annuity to continue a certain number of years, allowing simple interest for the money.

Let P be the present value; then if P, and the annuity, at the same rate of interest, amount to the same sum, they are upon the whole of equal value. The amount of P, in n years, is P + nrP (Art. 391); and the amount of the annuity in the same time is n A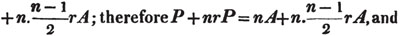

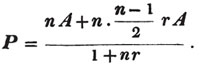
(395.) In this equation any three of the four quantities P, A, n, r being given, the other may be found.

(396.) COR. Let n be infinite, then P = nA/2 an infinite quantity, therefore for a finite annuity to continue for ever, an infinite sum ought, according to this calculation, to be paid; a conclusion which shews the necessity of estimating the value of an annuity upon different principles.

[page] 223

(397.) To find the amount of a given sum at compound interest.

Let R = £1. together with it's interest for a year; then at the end of the first year, R becomes the principal, or sum due; therefore

1 : R :: R : R², the amount in two years;

1 : R :: R² : R³, the amount in three years; &c. in the same manner, Rⁿ is the amount in n years; and if P be the principal, the amount must be P times as great, or PRⁿ = M.

(398.) COR. 1. From this equation we have P = M/Rⁿ.

Ex. What sum must be paid down to receive £600. at the end of three years, allowing 5 per cent, per ann. compound interest;

In this case, R = 1.05, n = 3, M = 600; and consequently P = M/Rⁿ =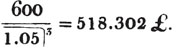

(399.) COR. 2. If P, R and M be given to find n, we have log. P + n×log. R = log. M; and n =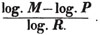

(400.) To find the amount of an annuity in any number of years, at compound interest.

Let A be the annuity, or sum due at the end of the first year; then 1 : R :: A : RA, it's amount at the end of the second year; therefore A + RA is the sum due at the end of the second year; in the same manner, 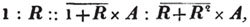the amount of

[page] 224

the two payments at the end of the third year; and 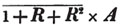is the whole sum due at the end of the third year; in the same manner, 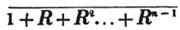is the sum due at the end of n years, that is, 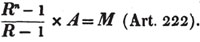(Art. 222).

(401.) COR. In this equation, any three of the quantities being given, the fourth may be found.

(402.) To find the present value of an annuity to be paid for n years, allowing compound interest.

Let P be the present value, A the annuity; then since PRⁿ is the amount of P in n years, and 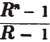×A, the amount of A in the same time; by the question,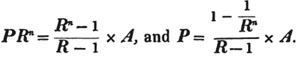

(403.) COR. 1. Any three of the quantities P, A, R, n being given, the fourth may be found.

(404.) COR. 2. If the number of years be infinite, Rⁿ is infinite, and 1/Rⁿ vanishes; therefore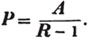

Ex. If the annual rent of a freehold estate be £1., what is it's value, allowing 5 per cent, compound interest?

In this case, A = 1, R - 1 = .05; therefore the present value P = 1/.05 = £20., or 20 years purchase.

[page] 225

(405.) COR. 3. The present value of an annuity, to commence at the expiration of p years, and to continue q years, is the difference between it's present value for p + q years, and it's present value for p years.

Ex. What is the present value of an annuity of £l., for 14 years, to commence at the expiration of 7 years, allowing 5 per cent, compound interest?

The present value for 21 years = 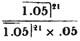= 12.82£ and the present value for 7 years = 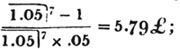hence, the value of the annuity for 14 years after the expiration of 7, is 7.03 £., nearly.

SCHOLIUM.

(406.) The method of determiningthe present value of an annuity at simple interest, given in Art. 394, has been decried by several eminent Arithmeticians, and in it's stead, a solution of the question has been proposed upon the following principle; "If the present value of each payment be determined separately, the sum of these values must be the value of the whole annuity."

Let x be the value or price paid down for the annuity, a the yearly payment, n the number of years for which it is to be paid, r the interest of £1. for one year. The present value of the first payment is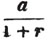

P

[page] 226

(Art. 392), the present value of the second payment, or of a£. to be paid at the end of two years, is 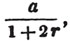and so on; therefore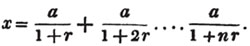

(407.) These different conclusions arise from a circumstance which the Opponents seem not to have attended to. According to the former solution, no part of the interest of the price paid down is employed in paying the annuity, till the principal is exhausted.

Let the annuity be always paid out of the principal x as long as it lasts, and afterwards out of the interest which has accrued; then x, x - a, x - 2a, x - 3 a, &c. are the sums in hand, during the first, second, third, fourth, &c. years, the interest arising from which, rx, rx - ra, rx - 2ra, rx - 3ra, &c. that is, the whole interest, is 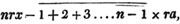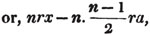which, together with the principal x, is equal to the sum of all the annuities; therefore 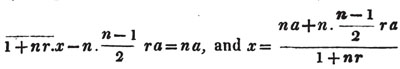(Art. 394).

According to the other calculation, part of the interest, as it arises, id employed in paying the annuity, but hot the whole. Thus, the first payment is made by a part of the principal, and the interest of that part, which together amount to the annuity; and the other payments are made in the same manner; this is, in

[page] 227

effect, allowing interest upon that part of the whole interest which is incorporated with the principal. According to either calculation the seller has the advantage, since the whole, or a part of the interest will remain at his disposal till the last annuity is paid off.

If the whole interest, as it arises, be incorporated with the principal, and employed in paying the annuity, compound interest is, in effect, allowed upon the whole. Let x be the price paid for the annuity, n the number of years for which itis granted, and R = 1£. together with it's interest for one year. Then x in one year amounts to Rx, out of which the annuity being paid, Rx - a is the sum in hand at the end of the first year; R²x - Ra is the amount of this sum at the end of the second year, therefore R²x - Ra - a is the sum in hand at the end of the second year; in the same manner, R^xx - R^{n - 1}a - R^{n - 2}a … a is the sum left, after paying the last annuity, which ought to be nothing; therefore Rⁿx = R^{n - 1}a + R^{n - 2}a …… + a = 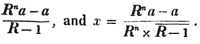(Art. 402).

ON THE SUMMATION OF SERIES.

(408.) We have before seen the method of determining the sums of quantities in arithmetical and geometrical progression, but when the terms increase or decrease according to other laws, different artifices must be used to obtain-general expressions for their sums.

P 2

[page] 228

The methods chiefly adopted, and which may be considered as belonging to Algebra, are, 1. The method of subtraction. 2. The summation of recurring series, by the scale of, relation. 3. The Differential method. 4. The method of Increments.

(409.) The investigation of series whose sums are known by subtraction.

Ex. 1.

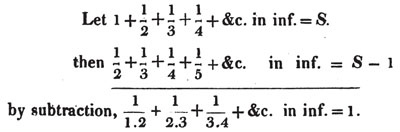
Ex. 2.

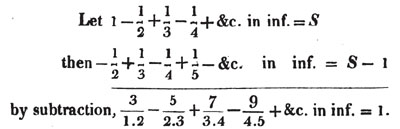
Ex. 3.

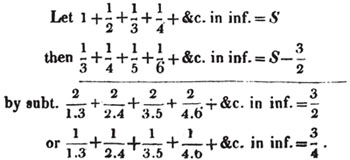
[page] 229

In the same manner, if 1 - 1/2 + 1/3 - 1/4 + &c. in inf. = S, we obtain 1/1.3 - 1/2.4 + 1/3.5 - &c. in inf. = 1/4.

Ex. 4.

Let 1/1.2 + 1/2.3 + 1/3.4 + &c. in inf. = S

then 1/2.3 + 1/3.4 + 1/4.5 + &c. in inf. = S - 1/2

by subt. 2/1.2.3 + 2/2.3.4 + 2/3.4.5 + &c. in inf. = 1/2

and 1/1.2.3 + 1/2.3.4 + 1/3.4.5 + &c. in inf. = 1/4.

Ex. 5.

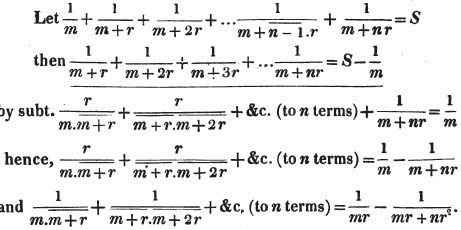
If n be increased without limit, 1/mr + nr² vanishes, and the sum of the series is 1/mr.

If m = r = 1, we have 1/1.2 + 1/2.3 + 1/3.4 + &c. (to n terms) = 1 - 1/ 1 + n = n / 1 + n.

[page] 230

In the same manner,

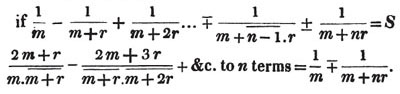
Ex. 6.

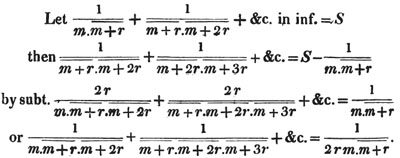
The sum of n terms of the series, determined as in the last example, is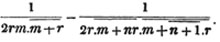

Let m = r = 1; then 1/1.2.3 + 1/2.3.4 + 1/3.4.5 +&c. in infinitum = 1/4; and 1/1.2.3 + 1/2.3.4 + &c. to n terms, =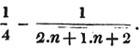

Ex. 7.

(410.) To find the sum of the series 1/2.4.6 + 1/4.6.8 + 1/6.8.10 + &c. in infinitum.

When the sum of a series of this kind is required, take away the last factor out of each denominator, and assume the resulting series equal to S; and then proceed as in the former examples.

[page] 231

Let 1/2.4 + 1/4.6 + 1/6.8 + &c. in inf. = S

then 1/4.6 + 1/6.8 + 1/8.10 + &c. in inf. = S - 1/8

by subt. 4/2.4.6 + 4/4.6.8 + 4/6.8.10 + &c. in inf. = 1/8

and 1/2.4.6 + 1/4.6.8 + 1/6.8.10 + &c. in inf. = 1/32.

Ex. 8.

(411.) To find the sum of the series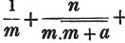

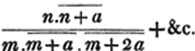in infinitum, when n is less than m.

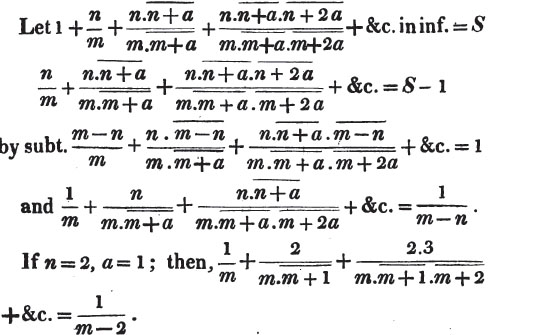
Ex. 9.

To find the sum of the series 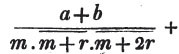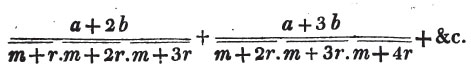infinitum.

[page] 232

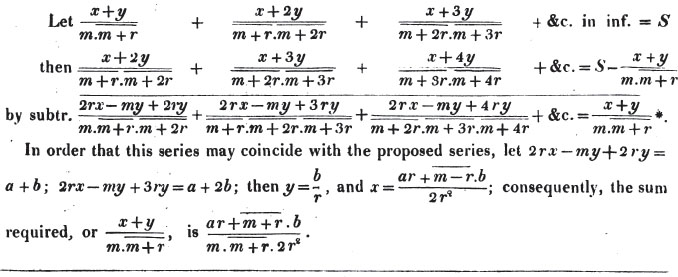
* Here we take for granted that the terms of the assumed series converge to 0.

[page] 233

Let a = 3, b = m = r = 1, then 4/1.2.3 + 5/2.3.4 + 6/3.4.5 + &c. in inf. =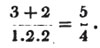

Let a = 0, b = 1, m = 1, r = 2; then 1/1.3.5 + 2/3.5.7 + 3/5.7.9 + &c. in inf. = 1/8.

See Philosophical Transactions, Vol.lxxii. page 389.

(412.) Similar to the method of subtraction is the following, given. by De Moivre, Miscel. Anal. p. 130.

Assume a series, whose terms converge to 0, involving the powers of an indeterminate quantityx; call the sum of the series S, and multiply both sides of the equation by a binomial, trinomial, &c. which involves the powers of x and invariable coefficients; then if x be so assumed that the binomial, trinomial, &c. may vanish, and some of the first terms be transposed, the sum of the remaining series is equal to the terms so transposed.

Ex. 1.

Let 1 + x/2 + x²/3 + x³/4 +&c. in inf. = S.

[page] 234

Multiplying both sides by x-1, we have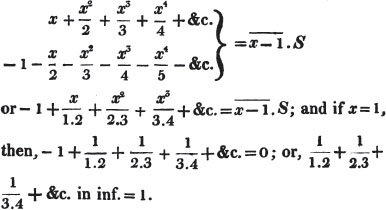

Ex. 2.

Assume 1 + x/2 + x²/3 + x³/4 + &c. in inf. = S, and multiplying both sides by x² - 1; then

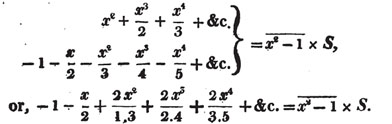
Let x² - 1 = 0, then x = 1, or - 1; if x = 1, then - 1 - 1/2 + 2/1.3 + 2/2.4 + 2/3.5 + &c. in inf. = 0,

that is, 2/1.3 + 2/2.4 + 2/3.5 + &c. in inf. = 3/2,

and 1/1.3 + 1/2.4 + 1/3.5 + &c. in inf. = 3/4.

[page] 235

If x = - 1, then

- 1 + 1/2 + 2/1.3 - 2/2.4 + 2/3.5 - &c. in inf. = 0,

and 1/1.3 - 1/2.4 + 1/3.5 - &c. in inf. = 1/4.

Ex. 3.

Let 1 + x/2 +x²/3 + x³/4 + &c. in inf. = S, and multiply both sides by 2x - 1; then

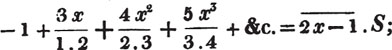let 2x - 1 = 0, or x = 1/2, and - 1 + 3/1.2.2 + 4/2.3.2² + 5/3.4.2³ + &c. = 0,

or 3/1.2.2 + 4/2.3.2² + 5/3.4.2³ + &c. in inf. = 1.

(413.) If both sides of the equation be multiped by a binomial, each term of the series obtained will have two factors in it's denominator; if by a trinomial, each term will have three factors in it's denominator; &c.

Ex. 4.

Let 1 + x/2 + x²/3 + x³/4 + &c. in inf. = S.

multiply both sides by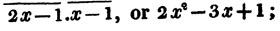

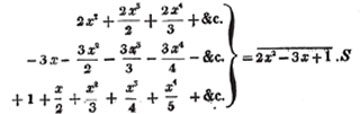
[page] 236

or 1 - 5x/2 + 5x²/1.2.3 + 6³/2.3.4 + 7x⁴/3.4.5 + &c. =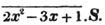

If x = 1, then 1 - 5/2 + 5/1.2.3 + 6/2.3.4 + &c. = 0, and 5/1.2.3 + 6/2.3.4 + 7/3.4.5 + &c. in inf. = 3/2. If x = 1/2 then 1 - 5/4 + 5/1.2.3.2² + 6/2.3.4.2³ + 7/3.4.5.2⁴ + &c. = 0, or 5/1.2.3.2² + 6/2.3.4.2³ + 7/3.4.5.2⁴ + &c. in inf. = 1/4.

Ex.5.

Let 1/m + x/ m + r + x²/m + 2r + &c. in inf. = S;

multiply both sides by the binomial ax - b; then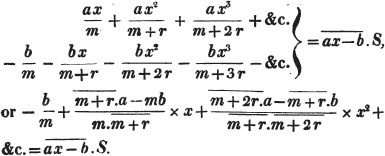

Let ax - b = 0, and transpose - b/m; then 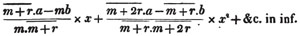= b/m. If the terms of this series can be made to coincide with the terms of a proposed series, the sum of the

[page] 237

latter may be found. Thus, let the sum of the infinite series 2/1.3×1/3 + 3/3.5×1/3² + 4/5.7×1/3³ + &c. be required.

In this case, x = 1/3, therefore a/3 - b = 0, or a = 3b; also, m = 1, r = 2, and 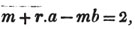that is, 3a - b, or 9b - b = 2; hence, b = 1/4, a = 3/4; and if these values be substituted in the succeeding terms, the general series coincides with the proposed one, whose sum is therefore b/m, or 1/4.

To find the sum of n terms of the series,

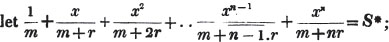
and proceeding as before,

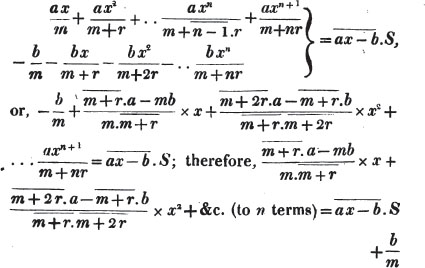
* This assumption renders the restriction in De Moivre's rule, respecting the convergence of the series, unnecessary.

[page] 238

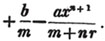If m = 1, r = 2, x = 1/3, a = 3/4, b = 1/4, the series becomes 2/1.3×1/3 + 3/3.5×1/3² + 4/5.7×1/3³ + &c. the sum of which, to n terms, is 1/4 -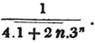

Ex. 6.

To find the sum of 19/1.2.3×1/4 + 28/2.3.4×1/8 + 39/3.4.5×1/16 + 52/4.5.6×1/32 + &c. in inf.

Because the factors in the denominators increase by 1, and begin from 1, assume 1 + x/2 + x²/3 + x³/4 + x⁴/5 + &c. = S, and multiply both sides by ax² - bx + c; then,

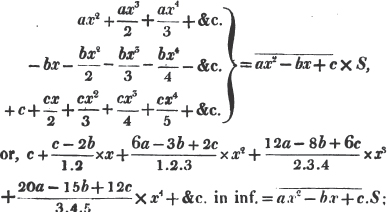
[page] 239

and since ax² - bx + c = 0, and one value of x is 1/2, because the powers of 1/2 are involved in the terms of the proposed series, we have a/4 - b/2 + c = 0; also, 6a - 3b + 2c = 19, and 12a - 8b + 6c = 28; from which three equations it appears that a = 6, b = 7, c = 2; and if these values be substituted in the general series, we have, 2 - 3 + 19/1.2.3×1/4 + 28/2.3.4×1/8 + 39/3.4.5×1/16 + 52/4.5.6×1/32 + &c. = 0, or, 19/1.2.3×1/4 + 28/2.3.4×1/8 + 39/3.4.5×1/16 + &c. = 1.

The sum of n terms of this series, determined as in the last example, is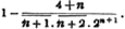

ON RECURRING SERIES.

(414.) If each succeeding term of a decreasing series bear an invariable relation to a certain number of the preceding terms, the sum of the series may be found.

Let a + bx + cx² + &c. be the proposed series; call it's terms A, B, C, D, &c. and let C = fxB + gx²A, D = fxC + gx²B, &c. where f + g is denominated the scale of relation; then, by the supposition,

[page] 240

A = A

B = B

C = fxB + gx²A

D = fxC + gx²B

E = fxD - gx²C

&c. &c.

and if the whole sum A + B + C+ D + &c. in inf. = S, we have,

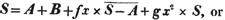
S - fxS - gx²S = A + B - fxA; therefore, S =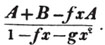

In the same manner, if the scale of relation be f + g + &c. to n factors, the sum of the series is

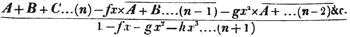
Ex. 1.

To find the sum of the infinite series 1 + 3x + 9x² + &c. when x is less than 1/3.

Here f = 3, and the sum =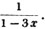

Ex. 2.

To find the sum of the infinite series 1 + 2x + 3x² + 4x² + &c. when x is less than 1.

Here, f = 2, g = - 1, and the sum =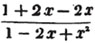

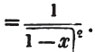
If x be equal to, or greater than 1, the series is infinite; yet we know that it arises from the division of 1 by 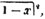and the sum of n terms may be determined.

[page] 241

The series after the n first terms becomes 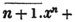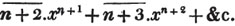in which the scale of relation, as before, is 2 - 1; and therefore the series arises from the fraction 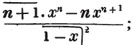therefore 1 + 2x + 3x² + &c. to n terms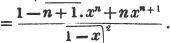

If the sign of x be changed, 1 - 2x + 3x² - &c. to n terms 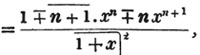where the upper or lower sign is to be used, according as n is an even or odd number.

Ex. 3.

To find the sum of n terms of the series 1 + 3x + 5x² + 7x³ + &c.

Suppose f + g to be the scale of relation; then, 3f + g = 5, and 5f + 3g = 7; hence, f = 2, and g = - 1; and, by trial, it appears that the scale of relation is properly determined; hence,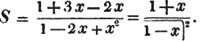

After n terms, the series becomes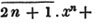

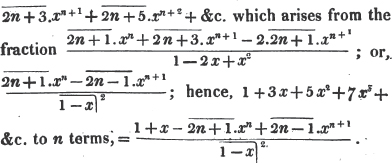
Q

[page] 242

Ex. 4.

To find the sum of 1 + 2x + 3x² + 5x³ + 8x⁴ + &c. in inf. when the series converges.

In this case the scale of relation is 1 + 1, and consequently, the sum is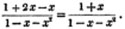

If x becomes negative, 1 - 2x + 3x² - 5x³ + &c. in inf.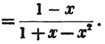

Ex. 5.

To find the sum of n terms of the series

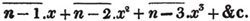
In the series 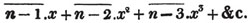the scale relation is 2 - 1; therefore it's sum in inf. is

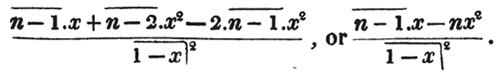After n terms, the series becomes - x^{n + 1} - 2x^{n + 2} - &c. the sum of which is found in the same manner, to be 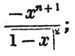therefore 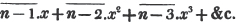to nterms,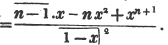

Hence, 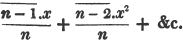to n terms, =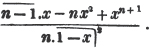

Ex. 6.

To find the sum of n terms of the series 1² + 2²x + 3²x² + 4²x³ = &c.

[page] 243

Let the scale of relation be f + g + h; then

9f + 4g + h = 16

16f + 9g + 4h = 25

25f + 16g + 9h = 36.

From these equations we obtain f = 3, g = - 3, h = 1, which values, when substituted, produce the successive terms of the proposed series; therefore S = 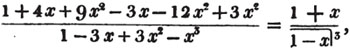the sum of the series in inf. when x is less than 1.

After the first n terms, the series becomes 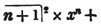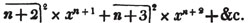which arises from the fraction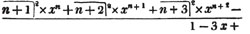 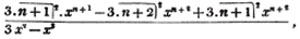or, 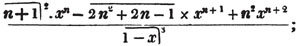and consequently the sum of n terms of the series is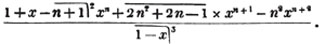

On this subject the Reader may consult De Moivre's Misc. Analyt. p. 72. And Euler's Analys. Infinit. C. XIII.

ON THE DIFFERENTIAL METHOD.

(415.) In any series of quantities a, b, c, d, e, f, &c. if each term be taken from that which follows it, and the differences of these differences be taken, and so on, the following ranks of differences will be obtained;

Q 2

[page] 244

1^st Diff. b - a, c - b, d - c, e - d, f - e, &c.

2^d Diff. c - 2b + a, d - 2c + b, e - 2d + c, f - 2e + d, &c.

3^d Diff. d - 3c + 3b - a, e - 3d + 3c - b, f - 3e + 3d - d, - c, &c.

4^th Diff e - 4d + 6c - 4b + a, f - 4e + 6d - 4c + b, &c.

5^th Diff. f - 5e + 10d - 10c + 5b - a, &c.

&c. &c.

Hence it appears, that the coefficients of the quantities a, b, c, d, &c. in the first term of the n^th differences, are the coefficients of the terms of a binomial raised to the n^th power, and that their signs are alternately positive and negative; that is, the first term of the n^th differences is 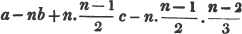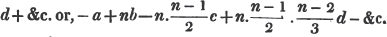according as n^th is an even or an odd number.

COR. If the n^th differences vanish, the term of the original series is 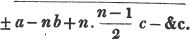to n terms; and the term is 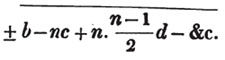that is, the series recurs, and the scale of the relation is 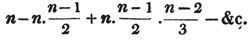continued to n terms.

(416.) Let d^I, d^II, d^III, d^IV, &c. represent the first terms in the first, second, third, fourth, &c. orders of differences; then

d^I = b - a

d^II = c - 2b + a

d^III = d - 3c + 3b - a

d^IV = e - 4d + 6c - 4b + a

&c.

[page] 245

and by transposition,

b = a + d^I

c = 2b - a + d^II = a + 2d^I + d^II

d = 3c - 3b + a + d^III = a + 3d^I+ 3d^II + d^III

e = 4d - 6c + 4b - a + d^IV = a + 4d^I + 6d^II + 4d^III + d^IV

&c. &c.

from which it is manifest, that the coefficients of a, d^I, d^II, d^III, &c. in the expression for the term of the series a, b, c, d, &c. are the coefficients of the terms of a binomial raised to the n^th power; that is, the term of the series is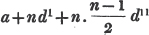

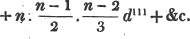
(417.) COR. 1. The n^th term of the series is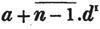

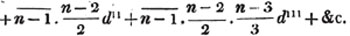
Ex.

Required the n^th term of the series 1, 3, 5, &c.

1, 3, 5, 7

2, 2, 2

0, 0

Here, a = 1, d^I = 2, d^II = 0; therefore the n^th term is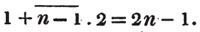

(418.) COR. 2. If the differences at length vanish, the n^th term of the series will be exactly determined; but if the differences do not vanish, we can only approximate to it; and the less the differences become, when compared with the former differences, and with n, the nearer will the approximation be to the true value of that term.

[page] 246

(419.) Let the proposed series be 0, a, a + b, a + b + c, a + b + c +d, &c. then,

1^st Diff. a, b, c, d, &c.

2^d Diff. b - a, c - b, d - c, &c.

3^d Diff. c - 2b + a, d - 2c + b, &c.

4^th Diff. d - 3c + 3b - a, &c.

&c.

Let b - a = d^I, c - 2b + a = d^II, d - 3c + 3b - a = d^III, &c. then the 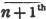term of the series, that is, a + b + c + d + &c. to n terms, is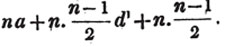 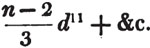(Art. 416).

If therefore a, b, c, d, &c. be the terms of any series, whose first, second, third, &c. differences are represented by d^I, d^II, d^III, &c. the sum, ofn terms of this series is

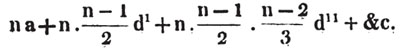
Ex. 1.

Required the sum of the series 1 + 3 + 5 + 7 + &c. continued to n terms.

1, 3, 5, 7

2, 2, 2

0, 0

In this case, a = 1, d^I = 2, d^II = 0; hence, the sum is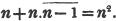

Ex. 2.

Required the sum of the series 1² + 2² + 3² + 4² + &c. continued to n terms.

1, 4, 9, 16

3, 5, 7

2, 2

0

[page] 247

Here, a = 1, d^I = 3, d^II = 2, d^III = 0; therefore the sum =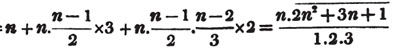

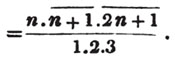
Ex. 3.

To find the sum of the series 1³ + 2³ + 3³ + &c. to n terms.

1, 8, 27, 64, 125

7, 19, 37, 61

12, 18, 24

6, 6

0

Here, a = 1, d^I = 7, d^II = 12, d^III = 6, d^IV = 0; therefore the sum =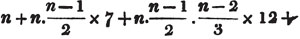

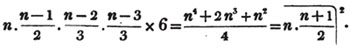
Sometimes the sum may be more readily obtained by beginning the series with one or more cyphers; thus, to find the sum of n terms of the series 1³ + 2³ + 3³ + &c. take n + 1 terms of the series 0 + 1³ + 2³ + 3³ + &c.

0, 1, 8, 27, 64

1, 7, 19, 37

6, 12, 18

6, 6

0

Here, a = 0, d^I = 1, d^II = 6, d^III = 6; and the sum of

[page] 248

of n +1 terms is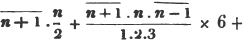

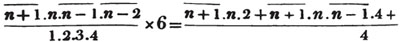
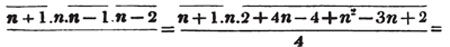
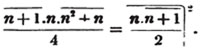
The differential method may also be applied to the interpolation of series, and the quadrature of curves. See Emerson's Tract on this subject.

ON THE METHOD OF INCREMENTS.

(420.) Any variable quantity is called an integral. The magnitude by which it is increased at one step, is called the increment. Thus, 1 + 2 + 3. … + m = and the magnitude by which it increases at one step is m + 1, which is called the increment of the integral

When the quantity decreases, the increment becomes negative.

(421.) If two quantities begin to increase together, and their corresponding increments be always in the same ratio, their integrals, or the whole quantities generated, will be in that ratio.

[page] 249

Let the corresponding increments be A, B, C, &c. and a, b, c, &c. and let A : a :: B : b :: C : c &c. :: m : n; then A + B + C + &c. : a + b + c + &c. :: m : n, (Art. 183).

COR. When m = n, or the increments are equal, the integrals are also equal.

(422.) If an integral be represented by the product of quantities in arithmetical progression, as 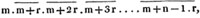where r, is constants, and m is increased at every step by r, the increment of this integral, is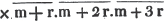

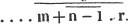
The first value of the integral is 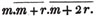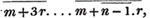and the succeeding value is 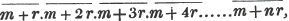the difference of these values, or the increment of the integral, is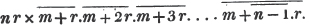

(423.) COR. 1. Since an invariable quantity C has no increment, the increment of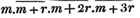

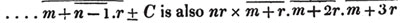
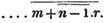
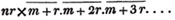
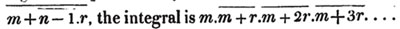
(424) COR. 2 Hence, if the increment of an integral be represented by 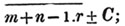where the invariable quantity C must be determined by the nature of the question.

[page] 250

(425.) COR. 3. If the increment be 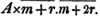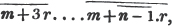where A is invarible, the integral is 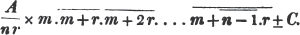(Art. 421.)

(426.) If A be the constant increment, and m the number of times it has been taken, the integral is mA ± C.

(427.) To find, therefore, the integral of any increment, let the increment be reduced to the products of arithmetical progressionals whose common difference is the quantity by which the variable magnitude is increased at every step, and the integral of each increment will be found by multiplying it by the preceding term in the progression, and dividing it by the number of terms, thus increased, and by the common difference.

(428.) The constant quantity which is to be added to, or subtracted from this result, in order to obtain the correct integral, must be determined by the nature of the question; thus, when x, the integral obtained by the rule, is a, suppose the true integral is known to be b; then since x + C is in all cases the integral, a + C = b, or C = b - a; therefore the correct integral is x + b - a.

Ex. 1.

To find the sum of the series 1+ 2 + 3 + &c. continued to n terms.

The n^th term is n, and the increment of the sum is n + 1, whose integral, according to the rule, is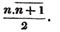

[page] 251

And this is the correct integral, because when n = 1, the sum is 1.2/2 = 1, as it ought to be.

Ex. 2.

To find the n^th term of the series 5, 9, 16, 26, 39, &c.

Take the differences, as in Art. 415,

5, 9, 16, 26, 39,

4, 7, 10, 13

3, 3, 3

It is manifest that the n^th term of any order of differences is the increment of the n^th term of the preceding order; therefore 3 is the increment of the n^th term of the first differences, and 3n + C is the n^th term; when n = 1, 3 + C = 4, or C = 1; hence the n^th term of the first differences, or the increment of the n^th term of the original series, is 3n + 1; consequently the n^th term required is

let n = 1, and 1 + C = 5, or C = 4; therefore the n^th term is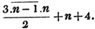

Ex. 3.

To find the sum of the series 1² + 2² + 3² + &c. continued to n terms.

The increment of this sum is 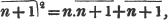and the integral, or sum, is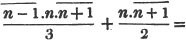 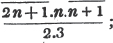which needs no correction.

Ex. 4.

To find the sum of the series 1² + 3² + 5² + &c. continued to n terms.

[page] 252

The increment of the sum is 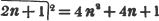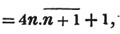, whose integral is 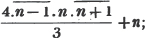which needs no correction.

Ex. 5.

To find the sum of n terms of the series. 1³ + 2³ + 3³ + &c.

The increment of the sum is 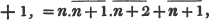whose integral is 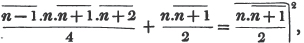the sum required; which needs no correction.

The following table of figurate numbers is formed by making the n^th term of each succeeding rank equal to the sum of n terms of the preceding.

1^st Order 1, 1, 1, 1, 1, 1

2^d 1, 2, 3, 4, 5, 6

3^d 1, 3, 6, 10, 15, 21

4^th 1, 4, 10, 20, 35, 56

5^th 1, 5, 15, 35, 70, 126

&c. &c.

Ex. 6.

To find the sum of n terms of the m^th order of figurate numbers.

The sum of n terms of the 1^st order is n; therefore n + 1 is the increment of the sum of n terms of the 2^d order, and it's integral, 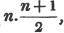is the n^th term of the 3^d order; consequently 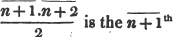term, or increment of the sum of n terms, of the 3^d order,

[page] 253

and it's integral, 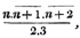is the sum of n terms of the 3^d order, or the n^th term of the 4^th order; &c.

Thus it appears that 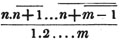is the sum which was to be found; the integrals requiring no correction.

Ex. 7.

To find the sum of n terms of the series 1.2 + 2.3 + 3.4 + &c.

The term, or increment of the sum, is 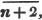and consequently the integral, or sum, is 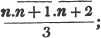which needs no correction.

Ex. 8.

To find the sum of the n first terms of a series whose n^th term is an³ + bn² + cn + d; a, b, c, d, being given quantities.

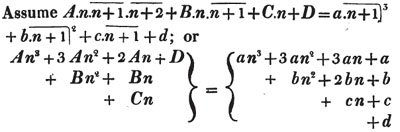
and by equating the coefficients, A = a; 3A + B = 3a + b, or 3a + B = 3a + b, that is, B = b; 2A + B + C = 3a + 2b + c, or 2a + b + C = 3a + 2b + c, hence, C = a + b + c; also, D = a + b + c + d; therefore the increment of the sum is 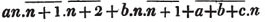+a+b+c+d, and the integral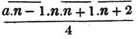

[page] 254

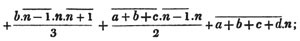
which requires no correction.

(429.) Though in general it is convenient to reduce an increment to the products of arithmetical progressionals, in order to obtain it's integral; yet if a quantity of any other form can be found, whose increment coincides with the increment proposed, this quantity, when properly corrected, is the integral (Art. 421).

Ex. 1.

To find the sum of n terms of the series 5 + 6 + 7 + &c.

Let An + Bn² + Cn³ + &c. be the sum required; it's increment is 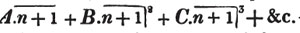- An - Bn² - Cn³ - &c. and the increment of the sum is also n + 5; therefore A + 2Bn + B + 3Cn² + 3Cn + C + &c. = n + 5; and by equating the coefficients, C = 0; 2B = 1, or B = 1/2; A + B = 5, or A = 9/2; hence the sum required is 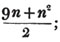which needs no correction.

Ex. 2.

To find the number of shot in a pyramidal pile upon a square base whose side is known.

Let n be the number in one side of the base; then n² is the number contained in the first square; also, since one shot in the next square, will lie between every two in the former, n - 1 is the number contained in the

[page] 255

side of the second square, and 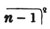the number in that square, &c. therefore the number of shot is n²+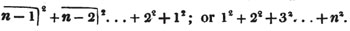

Suppose An + Bn² + Cn³ to be the sum of the series; it's increment is 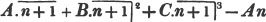- Bn² - CnM³; and the incrrment of the series is also 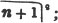therefore

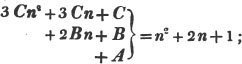
and by equating the coefficients, 3C = 1, or C = 1/3, 3C + 2B = 2, or B = 1/2; C + B + A = 1, or 1/3 + 1/2 + A = 1, hence A = 1/6; and the sum of the series is n/6 + n²/2 + n³/3; which requires no correction. If An + Bn² + Cn³ + Dn⁴ + &c. be assumed for the sum of the series, it is evident, from the process, that D, and the coefficient of every succeeding term, vanishes.

(430.) 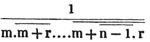represent an integral, where r is constant, and m increases at every step by r it's increments is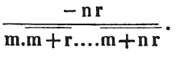

For, the next value of the integral is 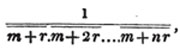from which if the preceding

[page] 256

value be taken, the difference or increment, is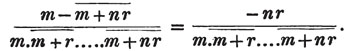

(431.) COR. 1. Hence, if 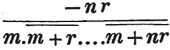represent an increment, it's integral is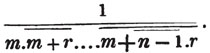

(432.) COR. 2. If the increment be 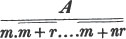the integral is 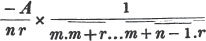(Art. 421).

That is, if an increment can be reduced to the form 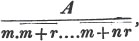it's integral may be found by rejecting the last term in the denominator, and dividing by the number of terms left, and by the common difference taken negatively.

This integral may be corrected as in Art. 428.

Ex. 1.

To find the sum of m terms of the series 1/1.2.3 + 1/2.3.4 + 1/3.4.5 + &c.

The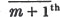 term, or increment of the sum, is 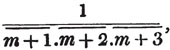whose integralis 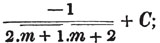and when m = 1, the integral is 1/1.2.3; therefore -1/2.2.3

[page] 257

+ C = 1/1.2.3, and C = 1/1.2.3 + 1/2.2.3 = 3/12 = 1/4; hence, the correct integral, or sum required, is 1/4 -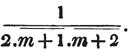

Ex. 2.

To find the sum of n terms of the series 5/1.2.3.4 + 7/2.3.4.5 + 9/3.4.5.6 + &c.

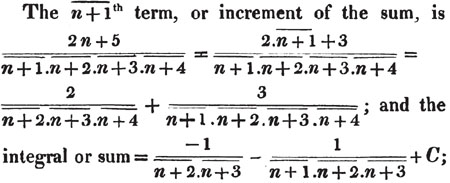and when n = 1, the sum is 5/1.2.3.4; therefore - 1/3.4 - 1/2.3.4 + C = 5/1.2.3.4; hence, 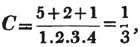and the correct integral, or sum required, is 1/3 -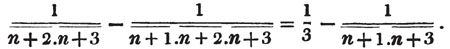

R

[page] 258

Ex. 3.

To find the sum of n terms of the series 1/1.3 + 1/2.4 + 1/3.5 + 1/4.6 + &c.

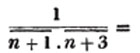
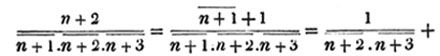
The increment of the sum is 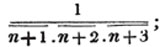therefore the sum is -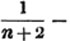 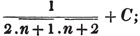let n = 1, then - 1/3 - 1/2.2.3 + C = 1/1.3; hence, C = 2/3 + 1/12 = 9/12 = 3/4, and the sum required is 3/4 -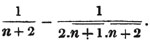

Ex. 4.

To find the sum of n terms of the series 1/1.3 + 1/3.5 + 1/5.7 + &c.

The increment of the sum is 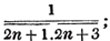assume 2v = 2n + 1, then v increases as fast as n, i.e. it increases by 1 at every step; and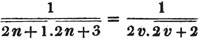

[page] 259

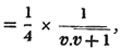whose integral is - 1/4v + C = - 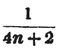+ C; and when n = 1, we have - 1/6 + C = 1/3, or C = 1/2; therefore the sum required is 1/2 -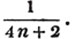

They who wish to prosecute this subject farther, may consult Dr. Waring's Fluxions, Sterling's Summation of Series, and Emerson's Method of Increments.

ON CHANCES.

(433.) If an event may take place in n different ways, and each of these be equally likely to happen, the probability that it will take place in a specified way is properly represented by 1/n, certainty being represented by unity: Or, which is the same thing, if the value of certainty be unity, the value of the expectation that the event will happen in a specified way is 1/n.

For, the sum of all the probabilities is certainty, or unity, because the event must take place in some one of the ways, and the probabilities are equal; therefore each of them is 1/n.

(434) COR. If the value of certainty be a, the value of the expectation is a/n. But in the following articles we suppose the value of certainty to be unity.

R 2

[page] 260

(435.) If an event may happen in a ways, and fail in b ways, any of these being equally probable, the chance of it's happening is 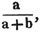and the chance of it's failing is .

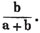The chance of it's happening must, from the nature of the supposition, be to the chance of it's failing, as a to b; therefore the chance of it's happening: chance of it's happening, together with the chance of it's failing :: a : a + b; and the event must either happen or fail, consequently the chance of it's happening together with the chance of it's failing is certainty; hence, the chance of it's happening: certainty :: a : a + b; and the chance of it's happening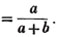

Also, since the chance of it's happening together with the chance of it's failing is certainty, which is represented by unity, 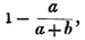that is, 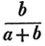is the chance of it's failing.

Ex. 1.

(436.) The probability of throwing an ace with a single die, in one trial, is 1/6; the probability of not throwing an ace is 5/6; the probability of throwing either an ace or a deuce, is 2/6; &c.

[page] 261

Ex. 2.

(437.) If n balls, a, b, c, d, &c. be thrown promiscuously into a bag, and a person draw out one of them, the probability that it will be a is 1/n; the probability that it will be either a or b is 2/n.

Ex. 3.

(438.) The same supposition being made, if two balls be drawn out, the probability that these will be a and b is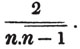

For there are 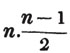combinations of n things taken two and two together (Art. 230); and each of these is equally likely to be taken; therefore the probability that a and b will be taken is

Ex. 4.

(439.) If 6 white and 5 black balls be thrown promiscuously into a bag, and a person draw out one of them, the probability that this will be a white ball is 6/11; and the probability that it will be a black ball is 5/11.

(440.) From the Bills of Mortality in different places, tables have been constructed which shew

[page] 262

how many persons, upon an average, out of a certain number born, are left at the end of each year, to the extremity of life. From such tables, the probability of the continuance of a life, of any proposed age, is known.

Ex. 1.

(441.) To find the probability that an individual of a given age will live one year.

Let A be the number, in the tables, of the given age, B the number left at the end of the year; then B/A is the probability that the individual will live one year; and 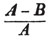the probability that he will die in that time (Art. 435). In Dr. Halley's Tables, out of 586 of the age of 22, 579 arrive at the age of 23; hence, the probability that an individual aged 22 will live one year is 579/586 or 83/84 nearly; and 7/586, or 1/84 nearly, is the probability that he will die in that time.

Ex. 2.

(442.) To find the probability that an individual of a given age will live any number of years.

Let A be the number, in the tables, of the given age, B, C, D,. … X, the number left at the end of 1, 2, 3,. … t, years; then B/A is the probability that

[page] 263

the individual will live one year; C/A the probability that he will live 2 years; and X/A the probability that he will live t years. Also, 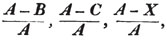are the probabilities that he will die in 1, 2, t, years.

These conclusions follow immediately from Art. 435.

(443.) If two events be independent of each other, and the probability that one will happen be 1/m, and the probability that the other will happen be 1/n, the probability that they will both happen is 1/mn.

For, each of the m ways in which the first can happen or fail, may be combined with each of the n ways in which the other can happen or fail, and thus form mn combinations, and there is only one in which both can happen; therefore the probability that this will be the case is 1/mn (Art. 433.)

(444.) COR. 1. The probability that both do not happen is 1 - 1/mn, or 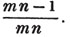For, the probability that they both happen, together with the probability that they do not both happen is certainty; therefore if from unity, the probability that they both happen

[page] 264

be subtracted, the remainder is the probability that they do not both happen.

(445.) COR. 2. The probability that they will both fail is 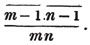For, the probability that the first will fail is 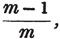and the probability that the second will fail is 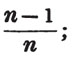therefore the probability that they will both fail is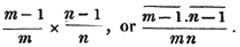

(446.) COR. 3. The probability that one will happen and the other fail is 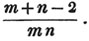For, the probability that the first will happen and the second fail is 1/m× 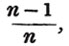and the probability that the first will fail and the second happen is 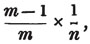and the sum of these, or 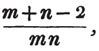is the probability that one will happen and the other fail.

(447.) COR. 4. If there be any number of independent events, and the probabilities of their happening be 1/m, 1/n, 1/r, &c. respectively, the probability that they will all happen is 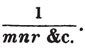For, the probability that

[page] 265

the two first will happen is 1/mn, and the probability that the two first and third will happen is 1/mnr; and the same proof may be extended to any number of events. When m = n = r &c. the probability is 1/m^v, v being the number of events.

Ex. 1.

(448.) Required the probability of throwing an ace and then a deuce with one die.

The chance of throwing an ace is 1/6, and the chance of throwing a deuce in the second trial is 1/6; therefore the chance of both happening is 1/36.

Ex. 2.

(449.) If 6 white and 5 black balls be thrown promiscuously into a bag, what is the probability that a person will draw out first a white, and then a black ball?

The probability of drawing a white ball first is 6/11 (Art. 439), and this being done, the probability of drawing a black ball, is 5/10, or 1/2, because there are 5 white and 5 black balls left; therefore the probability required is 6/11×1/2 = 3/11. Or we may reason thus;

[page] 266

unless the person draw a white ball first, the whole is at an end; therefore the probability that he will have a chance of drawing a black ball is 6/11, and when he has this chance, the probability of it's succeeding is 5/10, or 1/2; therefore, the probability that both these events will take place is 6/11×1/2, or 3/11.

Ex. 3.

(450.) The same supposition being made, what is the chance of drawing a white ball and then two black balls?

The probability of drawing a white ball and then a black one is 3/11 (Art. 449); when these two are removed, there are 5 white and 4 black balls left; and the probability of drawing a black ball, out of these, is 4/9; therefore the probability required is 3/11×4/9 = 4/33.

Ex. 4.

(451.) Required the probability of throwing an ace' with a single die, in two trials.

The chance of failing the first time is 5/6, and the chance of failing the next is 5/6; therefore the chance

[page] 267

of failing twice together is 25/36, and the chance of not failing, both times, is 1 - 25/36, or 11/36.

Ex. 5.

(452.) In how many trials may a person undertake, for an even wager, to throw an ace with a single die?

Let x be the number of trials; then, as in the last Art. the chance of failing x times together is 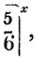and this, by the question, is equal to the chance of happening, or 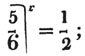hence, x ×log. 5/6 = log. 1/2, or x ×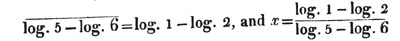 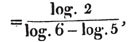since log. 1 = 0; i.e.x = 3.8, nearly.

Ex. 6.

(453.) To find the probability that two individuals, P and Q, whose ages are known, will live a year.

Let the probability that P will live a year, determined by Art. 441, be 1/m; and the probability that Q will live a year, 1/n; then the probability that they will both be alive at the end of that time is 1/m×1/n or 1/mn.

[page] 268

Ex. 7.

(454.) To find the probability that one of them, at least, will be alive at the end of any number of years.

The probability that P will die in a year is 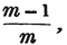and the probability that Q will die is 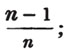therefore the probability that they will not both die is 1 - 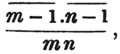and the probability that they will not both die is 1-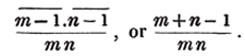

In the same manner, if 1/p be the probability that P will live t years, and 1/q the probability that Q will live the same time (Art. 442); the probability that one of them, at least, will be alive at the end of the time is 1-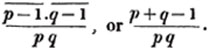

(455.) If the probability of an event's happening in one trial be represented by 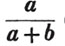(Art. 435), to find the probability of it's happening once, twice, three times, &c. exactly, in n trials.

The probability of it's happening in any one particular trial being 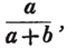the probability of it's failing in a all the other n - 1 trials is 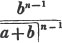(Arts. 435.

[page] 269

447); therefore the probability of it's happening in one particular trial, and failing in the rest, is 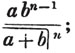and since there are n trials, the probability that it will happen in some one of these, and fail in the rest, is n times as great, or 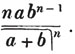The probability of it's happening in any two particular trials, and failing in all the rest, is 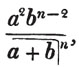and there are 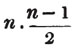ways in which it may happen twice in n trials and fail in all the rest (Art. 230); therefore the probability that it will happen twice in n trials is 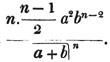In the same manner, the probability of it's happening exactly three times is 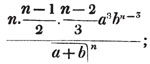and the probability of it's happening exactly t times is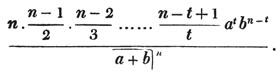

(456.) COR. 1. The probability of the event's failing exactly t times in n trials may be shewn, in the same way, to be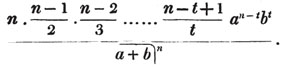

(457.) COR. 2. The probability of the event's

[page] 270

happening at least t times in n trials is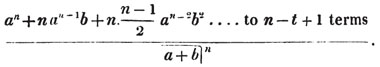

For, if it happen every time, or fail only once, twice, …. n - t times, it happens t times; therefore the whole probability of it's happening, at least t times, is the sum of the probabilities of it's happening every time, of failing only once, twice, …. n - t times; and the sum of these probabilities is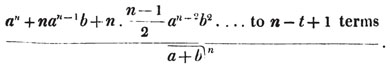

Ex. 1.

(458.) What is the probability of throwing an ace, twice, at least, in three trials, with a single die?

In this case, n = 3, t = 2, a = 1, b = 5; and the probability required is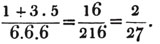

Ex. 2.

(459.) What is the probability that out of five individuals, of a given age, three, at least, will die in a given time?

Let 1/m be the probability that any one of them will die in the given time (Art. 442); then we have given the probability of an event's happening in one instance, to find the probability of it's happening three times, at least, in five instances.

[page] 271

In this case, a = 1, b = m - 1, n = 5, t = 3; therefore the probability required is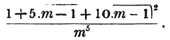

SCHOLIUM.

(460.) Much more might be said on a subject so extensive as the doctrine of chances; the Learner will however find the principal grounds of calculation in Articles 433, 435, 443, 455, and 457, and if he wish for farther information, he may consult De Moivre's work on this subject. It may not be improper to caution him against applying principles which, on the first view, may appear self-evident, as there is no subject in which he will be so likely to mistake as in the calculation of probabilities. A single instance will shew the danger of forming a hasty judgement, even in the most simple case. The probability of throwing an ace with one die is 1/6, and since there is an equal probability of throwing an ace in the second trial, it might be supposed that the probability of throwing an ace in two trials is 2/6.

This is not a just conclusion (Art. 451); for, it would follow, by the same mode of reasoning, that in six trials a person could not fail to throw an ace. The error, which is not easily seen, arises from a tacit supposition that there must necessarily be a second trial, which is not the case if an ace be thrown in the first.

[page] 272

ON LIFE ANNUITIES.

(461.) To find the present value of an annuity of £1. to be continued during the life of an individual of a given age, allowing compound interest for the money.

Let r be the amount of £1., in one year; A the number of persons, in the tables, of the given age; B, C, D, &c. the number left at the end of 1, 2, 3, &c. years (Art. 440); then B/A is the value of the life for one year, C/A, D/A, &c. it's value for 2, 3, &c. years; and the series must be continued to the end of the tables. Now the present value of £1., to be paid at the end of one year, is 1/r (Art. 398); but it is only to be paid on condition that the annuitant is alive at the end of the year, of which event the probability is B/A; therefore the present value of the conditional annuity is B/Ar (Art. 434); in the same manner, the present value of the second year's annuity is C/Ar²; the present value of the third year's annuity is D/Ar³; &c. therefore the whole value required is 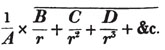to the end of the tables.

[page] 273

(462.) De Moivre supposes, that out of eighty-six persons born, one dies every year, till they are all extinct.

This supposition is sufficiently exact, if our calculations be made for any age above ten, as will appear from an inspection of the tables; and on this supposition, the sum of the series 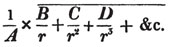may be found.

Let n be the number of years which any individual wants of 86; then will n be the number of persons living, of that age, out of which one dies every year; and 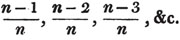will be the probabilities of his living, 1, 2, 3, &c. years; hence, the present value of an annuity of £1., to be paid during his life, is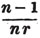 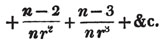continued to n terms. The sun of the series 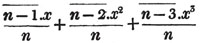to n terms, was found Art. 414. Ex. 3. to be 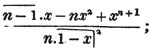let x = 1/r, and the sum of the series,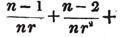 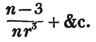to n terms, is 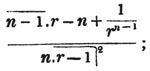the present value of the annuity.

S

[page] 274

(463.) COR. 1. This expression for the sum is the same with 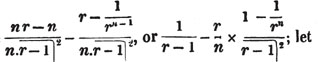P be the present value of an annuity of £.1., to continue certain for n years, then P = 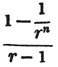(Art.402); and the expression becomes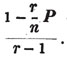

(464.) COR. 2. The present value of the annuity to continue for ever, from the death of the proposed individual, is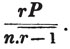

For, the whole present value of the annuity to continue for ever, is 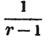(Art. 404); and if from this, it's value for the life of the individual be taken, the remainder 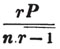is the present value of the annuity to continue for ever, from the time of his death.

(465.) To find the present value of an annuity of £.1., to be paid as long as two specified individuals are both living.

Find, by Art. 453, the probability that they will both be alive at the expiration of 1, 2, 3, &c. years, to the end of the tables; call these probabilities a, b, c, &c.

[page] 275

and r the amount of £1., in one year; then a/r + b/r² + c/r³ + &c. is the present value of the annuity required. (See Art. 461).

(466.) To find the present value of an annuity of £1., to be paid as long as either of two specified individuals is living.

Find, by Art. 454, the probability that they will not both be extinct in 1, 2, 3, &c. years, to the end of the tables, and call these probabilities A, B, C, &c. then the present value of the annuity is A/r + B/r² + C/r³ + &c. (See Art. 461).

(467.) COR. If the annuity be M £., the present value is M times as great as in the former case, or M×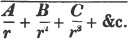

(468.) These are the mathematical principles on which the values of annuities for lives are calculated, and the reasoning may easily be applied to every proposed case. But in practice, these calculations, as they require the combination of every year of each life with the corresponding years of every other life concerned in the question, will be found extremely laborious, and other methods must be adopted when expedition is required. Writers on this subject, are De Moivre, Maseres, Simpson, Price, Morgan, and Waring.

THE END OF PART III.

S 2

[page 276]

THE

ELEMENTS OF ALGEBRA.

PART IV.

THE APPLICATION OF ALGEBRA TO GEOMETRY.

(469.) THE signs made use of in algebraical calculations being general, the conclusions obtained by their assistance may, with great ease and convenience, be transferred from abstract magnitudes to every class of particular quantities; thus, the relation of lines, surfaces, or solids, may generally be deduced from the principles of Algebra, and many properties of these quantities discovered, which could not have been derived from principles purely geometrical.

(470.) Simple algebraical quantities may be represented by lines.

Any line AB, may be taken at pleasure to represent one quantity a, but if we have a second quantity, b, to represent, we must take a line which has to the former line, the same ratio that b has to a.

[page] 277

Instead of saying AB represents a, we may say AB = a, supposing AB to contain as many linear units as a contains numeral ones.

(471.) When a series of algebraical quantities is to be represented on one line, and each of them measured from the same point, the positive quantities being represented by lines taken in one direction, the negative quantities must be represented by lines taken in the opposite direction.

Let a be the greatest of these quantities, then a - x may, by the variation of x, become equal to each of them in succession. Let AB be the given line, and A the point from which the quantities are to be measured; take AB = a; and since a - x must be

measured from A, BD must be taken in the contrary direction = x, then AD = a - x; and that a - x may successively coincide with each quantity in the series, beginning with the greatest positive quantity, x must increase; therefore BD, which is equal to x, must increase; and when x is greater than a, BD is greater than AB, and which represents the negative quantity a - x, lies in the opposite direction from A.

(472.) COR. l. If the algebraical value of a line be found to be negative, the line must be measured in a direction opposite to that which, in the investigation, we supposed to be positive.

(473.) COR. 2. If quantities be measured upon, a line from it's intersection with another, the positive quantities being taken in one direction, the negative quantities must be taken in the other.

[page] 278

(474.) If a fourth proportional, to lines representing p, q, r be taken, it will represent q^r/p; and if p = 1, it will represent q^r; if also, q and r be equal, it will represent q².

(475.) If a mean proportional between lines representing a and b be taken, it will represent √ab, which, when a = 1, becomes √b. Hence it appears that any possible algebraical quantities may be represented by lines; and conversely, lines may be expressed algebraically; and if the relations of the algebraical quantities be known, the relations of the lines are known.

(476.) The relations of surfaces to each other may be expressed algebraically.

Let the sides AB, AC of the rectangle AD contain the linear units a, b, respectively; then ab will be the number of superficial units contained in the

area. For, every unit in AB, or a, has b units in the area, corresponding to it; consequently there are, upon the whole, ab units in the area. Thus ab is a proper representation of the rectangle AD; and by reducing other surfaces, to rectangles, their algebraical values may be found.

[page] 279

COR. Hence, the product of the two quantities a and b, is often called their rectangle; and when b is equal to a, this product is called the square of a.

(477.) In the same manner, if a, b, c represent the linear units in the three sides of a rectangular parallelepiped, abc will be the number of solid units contained in the figure; and consequently solids may be compared, by comparing their algebraical values.

(478.) If the line PM move parallel to itself upon the indefinite line AP, and at the same time increase or decrease, the point M will trace out a straight line, or a curve. AP is called the abscissa, and PM the ordinate; and the straight line, or curve, is said to be the locus of the point M.

The nature of the curve depends upon the relation of AP to PM; and this relation, when expressed algebraically, is called the equation to the curve.

(479.) Having given the nature, or construction of the curve, its equation may be found.

Let BM be a straight line cutting AP in a given

angle at B, the relation of AP to PM is expressed by a simple equation.

Suppose AP = x, PM = y, AB = a; then since the angles at B, P and M are invariable, BP bears an invariable ratio to PM, let this be the ratio of b : c.

[page] 280

Then since BP = AP - AB = x - a, we have x - a : y :: b : c, and by = cx - ca, or by - cx + ca = 0.

(480.) COR. A simple equation belongs to a straight line; because, by altering the values of b, c, cand a, and taking x and a, positive or negative, as the case requires, the equation by - cx + ca = 0 may be made to coincide with any proposed simple equation.

(481.) To find the equation to the Parabola.

Let a point S be taken without the right line CB, and let the indefinite line SM revolve about the point

S in the plane SBC; also, let CM, which is perpendicular to CB, cut SM in M; then, if SM be always equal to CM, the locus of the point M is a parabola.

Through S draw BSP at right angles to CB, and if SB be bisected in A, the curve will pass through A, as appears by the construction; draw MP perpendicular to BP, and let AP = x. PM = y, AS = a; then SP² + PM² = (SM² = CM² =) BP², or 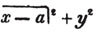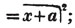that is, x² - 2ax + a² + y² = x² + 2ax + a², or y² = 4ax.

[page] 281

(482.) To find the equation to the Ellipse.

Let two indefinite lines SM, HM, revolve, in a given plane, about the points S, H, and cut each

other in M, in such a manner, that SM + MH may be an invariable quantity; then the locus of the point M is an ellipse.

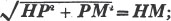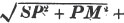
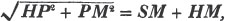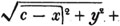
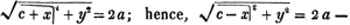
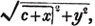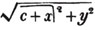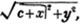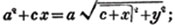Bisect SH in C, and from M draw MP perpendicular to SH, or SH produced; let CP = x, PM = y, CS = c, SM + MH = 2a. Then and therefore or and squaring both sides, c² - 2cx + x² + y² = 4a² - 4a× + c² + 2cx + x² + y²; that is, by transposition, 4a² + 4cx = 4a or and again squaring both sides, a⁴ + 2a²cx + c²x² = a²c² + 2a²cx + a²x² + a² y², or 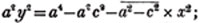let a² - c² = b², then a²y² = a²b²- b²x², and

[page] 282

(483.) COR. l. If S and H coincide, c = 0; hence, a = b, and y² = a² - x², the equation to a circle.

(484.) COR. 2. When x = + a, or - a, then y = 0; therefore taking CA = CD = a, the curve passes through A and D.

(485.) COR. 3. AP = z, then x = a - z; therefore 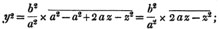the equation which expresses the relation between AP and PM.

(486.) COR. 4. If AS be finite, and SM + MH be indefinitely increased, the limit to which the curve approaches is, at all finite distances from S, a parabola.

In this case z² vanishes when compared with 2az; therefore the limit to which the equation approaches is y² = b²/a²×2az; also, b² = a² - c² = 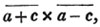and since the difference between a and c is finite and a is infinite, a + c is ultimately equal to 2a; hence, b² = 2a×AS; therefore 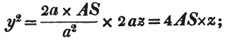the equation to the parabola.

(487.) To find the equation to the Hyperbola.

Let two indefinite lines SM, HM revolve, in a

[page] 283

given plane, about the points S, H, and cut each other in M, in such a manner that HM - SM may be a given quantity; then the locus of the point M is an Hyperbola.

Bisect SH in C, and draw MP perpendicular to HS, or HS produced; let CP = x, PM = y, SC = c, HM - SM = 2a. Then by proceeding as in Art. 482, 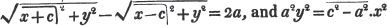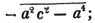in this case, 2c is greater than 2a (Euc. 20.1), let therefore b² = c² - a², then a²b² = b²x² - a²b², or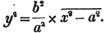

(488.) COR. 1. The equation to the ellipse, y² = b²/a²×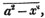 becomes the equation to the Hyperbola, if b² be supposed to be negative.

(489.) COR. 2. The equation 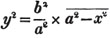may be considered as the equation to any conic section; it is the equation to an Ellipse, when b² is positive; to a Parabola, when b² is infinite (Art. 486); and to an Hyperbola, when b² is negative.

(490.) COR. 3. If SM - HM = 2a, a figure, similar and equal to the former, will be traced out, which is called the opposite Hyperbola.

(491.) COR. 4. If x = ± a, then y = 0; therefore taking CA - CD = a, the curve passes through A and D.

(492.) COR. 5. If AP = z, then CP, or x = z + a,

[page] 284

and x² - a² = z² + 2az; hence, 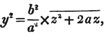the equation which expresses the relation between AP and PM.

(493.) COR. 6. In the opposite Hyperbola, x = z - a; therefore x² - a² = z² - 2az, and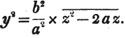

(494.) To find the equation to the Cissoid of Diodes.

Let AB be the diameter of a semicircle ANB; from the points R and P, taken always at equal

distances from A and B, draw RN, PM, at right angles to AB, and join AN meeting PM in M; the point M will trace out a curve called the Cissoid of Diodes.

From the nature of the circle, AR×RB = RN² and by the construction, AR×RB = PB×AP; also, from the similar triangles APM, ARN, AP : PM :: AR : RN, or AP : PM :: PB : 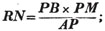and 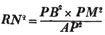= PB×AP; therefore PB ×

[page] 285

PM² = AP³. Let AB = b, AP = x, PM = y; then 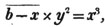the equation required.

(495.) To find the equation to the Conchoid of Nicomedes.

Let AB be a line given in position, and about any point C, taken without it, let the indefinite line CM

revolve, and cut AB in E; then if EM be taken always of the same length, the point M will trace out a curve which is called the Conchoid of Nicomedes. Draw CAD and MP at right angles to AB, and MF parallel to it; let CA = a, AD = EM = b, AP = x, PM = y. Then from the similar triangles CFM, MPE, CF(a + y) : FM(x) :: MP (y) : PE = xy/a + y; and EM² = EP² + PM², that is, 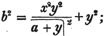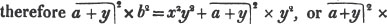the equation to the curve.

If EM be measured in the opposite direction from E, the equation to the curve is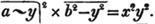

[page] 286

(496.) To find the equation to the Logarithmic Curve.

If in the indefinite line AE, we take AB, BC, CD, &c. always equal to each other, and ordinates

AF, BG, CH, DI, &c. be drawn at right angles to AE, and in geometrical progression, the curve FGHI &c. which passes through their extremities, is called the Logarithmic Curve. From the nature of logarithms (Art. 385), any abscissa AC is the logarithm of the corresponding ordinate CH, in a system which depends upon the magnitudes of AF and BG, supposing AB given; in the same system, let 1 be the logarithm of a; also, let AC = x, CH = y; then x = log.y, and 1 = log. a, or x = x x log.a; therefore log. y = x x log. a = log.a^x; hence, y = a^x, the equation to the curve.

(497.) Having given the relation between one abscissa CP, and ordinate PM, in a curve, to find the

relation between the abscissaSQ, which is measured from a given point S in a given direction, and the ordinate QM, which is inclined to PM at a given angle.

[page] 287

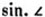
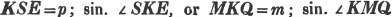
Suppose PM perpendicular to CP; produce MQ and DPC till they meet in G, draw SB, SD, SF respectively parallel to MG, MP, DC; and let 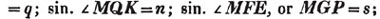to rad. 1; SB = FG = d, DC = f, CP = x, PM = y, SQ = z, QM = v.

Then in the triangle SQF, s : p :: z : pz/s = QF; hence, GM = GF + FQ + QM = d+ pz/s + v; and in the triangle MGP, 1 : s :: d + pz/s + v : sd + pz + sv = PM = y.

Also, in the triangle MKQ, m : q :: v: qv/m = KQ, and SK = SQ - KQ = z - qv/m; again, in the triangle SKE, 1 : m :: z - qv/m; mz - qv = SE = DP; hence, 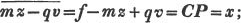and if these values of x and y be substituted in the equation which represents the relation of CP to PM, an equation is obtained which represents the relation of SQ to QM.

(498.) COR. 1. Since the values of x and y are represented in simple terms of z and v, the equation to the curve will rise. to the same number of dimensions, whatever abscissa and ordinate are taken.

(499.) COR. 2. From the principles of Trigonometry it appears, that m, n, and s may be found in terms of p and q; therefore in the values of x and y, before obtained, there are only four independent invariable quantities d, f, p, and q.

[page] 288

(500.) COR. 3. If the curve be a conic section whose centre is C and axis CP, then 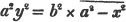(Art. 489), and substituting for x and y their values, we have 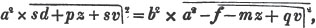or arranging the terms according to the dimensions of v,

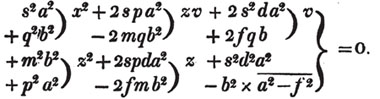
(501.) COR. 4. The equation obtained in the last article may be made to coincide with any equation of two dimensions Av² + Bzv + Cv + Dz² + Ez + F = 0, by equating the coefficients of the corresponding terms; because we shall have six equations to determine the six independent quantities, a², b², d, f, p, q. Hence it follows, that every equation of two dimensions belongs to some conic section.

(502.) Having given the equation which expresses the relation between the abscissa and ordinate, the curve may be described.

For, any abscissa being assumed, the corresponding values of the ordinate are known from the equation; and thus, by assuming different values of the abscissa, the curve may be traced out.

(503.) Ex. 1. If ay = bx + cd be the proposed equa-

tion, it belongs to a right line (Art. 480). Let the abscissa be measured from the point A, along the line

[page] 289

AB; then, when x = 0, we have y = cd/d; from A, therefore, draw AC making a finite angle with AB, and equal to cd/a, and the line which belongs to the proposed equation must pass through C Also, if y = 0, then x = - cd/b; take therefore, upon the line ADP, AD = cd/b, and the line to which the equation belongs must pass through D; therefore DCM is that line.

(504.) COR. If AP be taken to represent any value of x, and the ordinate PM be drawn parallel to AC, PM will represent the corresponding value of y.

(505.) Ex. 2. Let the equation to the curve be ax = y²; then, when x = 0, we have y = 0, or the curve passes through A; when x is positive, y = ± √ax, and when x is infinite, these values are still possible; therefore the curve has two infinite arcs lying the same way from A; but when x is negative, y

becomes impossible; therefore no part of the curve lies the other way.

T

[page] 290

(506.) Ex.3. Let the equation to the curve be xy = ab; then, when x is indefinitely small, y is indefinitely great, and when x is positive and indefinitely great, y is positive and indefinitely small; therefore the curve will have

two infinite arcs between the lines AE and AB; also, when x is negative, y is negative, and when infinite, y is infinitely small, and when x is infinitely small, y is infinitely great; therefore the curve will have two infinite ares between Ab and AF.

These lines EF, Bb, which continually approach to the curve, and whose distances from it become, at length, less than any that can be assigned, but which produced ever so far do not meet it, are called Asymptotes.

(507.) Ex.4. Let x⁴ - a²x² + a²y² = 0; then, y = ± 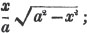and when x is nothing, y is nothing, or the curve passes through A, the point from which x

is measured. When x = ± a, then y = 0; therefore

[page] 291

the curve passes through B, and b, supposing AB = Ab = ± a; but if x be greater than a, y becomes impossible; therefore no part of the curve lies beyond B or b.

(508.) Ex. 5. To find the conic section to which any proposed quadratic equation belongs.

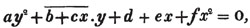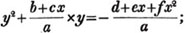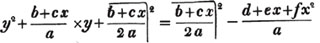
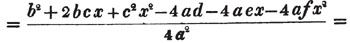
Let a general equation of two dimensions, be the proposed quadratic; then and completing the square, 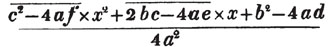and extracting the square root,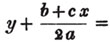 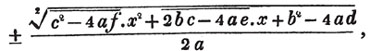and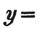

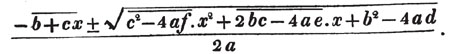
Hence, 1. If c² - 4af be positive, then, when ± x is infinite, y has four possible values; therefore the curve has four infinite arcs, or it is the hyperbola.

2. If c² - 4af = 0, the curve has only two infinite arcs; because, when 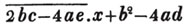becomes negative, thfe values ofy are impossible; and the curve is a parabola. But if 2bc - 4ae be also = 0, then y =

T 2

[page] 292

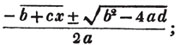and when b² is greater than 4ad, the curve becomes a right line.

3. If c² - 4af be negative, the curve has no infinite arc; for, when ± x is infinite, the values of y are impossible; hence the curve is an ellipse.

4. If c² - 4af be negative, 2bc - 4ae = 0, and b² - 4ad be also 0, or negative, all the values of y are impossible; in this case the ellipse wholly vanishes.

ON THE CONSTRUCTION OF EQUATIONS.

(509.) The relation between the abscissa and ordinate of a conic section is expressed by a quadratic equation, in which, for every different value of the abscissa, there are two corresponding values of the ordinate, and if the abscissa be so drawn, and the conic section so constructed, that it's equation coincides with a proposed quadratic, the two ordinates will be the roots of that quadratic, which may be determined to a tolerable degree of accuracy by actual measurement.

Let be a circle (a figure more easily described than any other conic section) whose centre is A and radius AM; take AP an abscissa, PM an ordinate meeting the circle in M and join AM, and draw MB at right angles to AP; let AP = x, PM = y, AM = r, and the cosine of the angle APM (to the radius l) = c; then 1 : c :: PM : PB = c x PM = cy,

[page] 293

and AM² - AP² + PM² AP² - 2AP x BP, or r² = x² + y²-

2cxy; that is, y² - 2cxy + x² - r² = 0; which equation may be made to coincide with any proposed quadratic.

Ex. Let the roots of the equation y² - py + q = 0 be required.

Here 2cx = p, and x² - r² = q; and since there are three undetermined quantities c, x, and r, and only two conditions to be answered, one of these quantities may be assumed, of any finite magnitude, at pleasure: suppose c = 1, then x = p/2, r² = x² - q = p²/4 - q, and and since the cosine of the 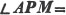radius, PM coincides with PAD; let therefore a circle be described with the radius 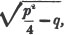cutting the line DAP in D and C; take AP = p/2, and the roots of the equation are PC and PD.

(510.) The intersections of two conic sections, may be determined by a biquadratic equation, and if the figures be so drawn that this biquadratic coincides with a proposed biquadratic, the roots of the latter

[page] 294

equation may be found by measuring the ordinates which determine the points of intersection.

Let be a parabola whose axis is AP, a circle whose centre is C and radius CM, cutting the parabola in the points

from these points draw the ordinates to the axis, MP, 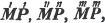and from C draw CD perpendicular to the axis, and CN parallel to it, meeting PM in N. Let AD = a, DC = b, CM = n, the parameter of the parabola = p, AP = x, PM = y; then px = y²; also, CM² = CN² + NM², or 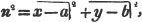i.e. x² - 2ax + a² + y² - 2by + b² = n²; and substituting for x it's value y²/p, and arranging the terms according to the dimensions of y, we obtain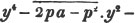 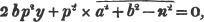a biquadratic eqation whose roots are PM, 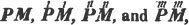and which may be made to coincide with any proposed biquadratic whose second term is wanting.

Ex. Let the roots of the equatiou y² - qy² + ry - s = 0 be required.

[page] 295

Assume p = l; then 2a - 1 = q, or 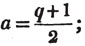- 2b = r, or b = - r/2; a² + b² - n² = - s, or n² = a² - b² + s, and consequently, 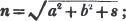describe a parabola whose parameter is 1, and, in the axis, take 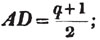draw DC at right angles to it, and = - r²/2; from the centre C, with the radius 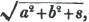describe the circle cutting the parabola in the points then the ordinates to the axis, 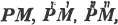and are the roots sought.

(511.) When DC represents a negative quantity, the ordinates on the same side of the axis with C represent the negative roots of the equation; and the contrary.

(512.) COR. 1. If the circle touch the parabola, two root of the equation are equal; if it cut it only in two points, or touch it in one, two roots are. impossible; and if the circle fell wholly within or without the parabola, or, if a² + b² + s be negative, all the roots are impossible.

(513.) COR. 2. If a² + b² = n², or the circle pass, through the point A, the last term of the equation, 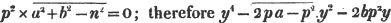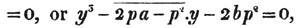a cubic equation, which may be made to coincide with a proposed cubic whose second term is wanting, and the ordinates PM, 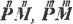are it's roots.

[page] 296

(514.) COR. 3. If a² + b² - n² = 0, and also b = 0, the equation becomes 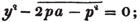by means of which, any quadratic, wanting the second term, may be solved. In this case, the circle passes through the vertex of the parabola, and it's centre falls in the axis.

These solutions may be obtained, and nearly in the same manner, by means of any two of the conic sections.

(515.) If the roots of a cubic equation, x³ - qx + r = 0, be possible, they may be found by means of a table of cosines.

Let DAC be an angle whose cosine, to the radius m, is x; in AD, take AB = m; from B as a centre, with the radius BA, describe a circle cutting AM in C, and

from C, with the same radius, describe a circle cutting AD in D; join BC, CD, and draw BK, DM at right angles to AM, and CL at right angles to AD. Then, the triangles BAC and BCD being isosceles, the angles BAC and BCD are equal, as also CBD and CDB; and the perpendiculars BK, CL bisect the bases AC, BD. Also, 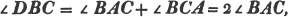and 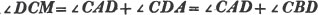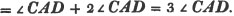Let CM, the cosine of the 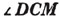to the radius m, be called c; then, from the similar triangles ABK, ACL, AB : AK :: AC : AL, or m : x :: 2x : 2x²/m = AL, and AL - AB

[page] 297

= 2x² - m = BL; hence, AD, or AL + BL, = 4x²/m - m; again, AB : AK :: AD : AM, or m : x :: 4x²/m - m : 4x³/m² - x = AM, and AM - AC = CM = 4x³/m² - x = AM, and AM - AC = CM = 4x³/m² - 3x = c, therefore 4x³ - 3m²x = m²c, and 4x³ - 3m²x - m²c = 0.

Let the equation 4x³ - 3m²x - m²c = 0, or x³ - 3m²/4x - m²/4 = 0, be made to coincide with the equation x³ - qx + r = 0; that is, let 3m²/4 = q, and m²c/4 = - r; or m = √4q/3, and c = - 3r/q; then from a table of cosines, find the angle whose cosine is -3r/q, to the radius √4q/3 and the cosine of one third of this angle, to the same radius, is one value of x.

(516.) COR. 1. If A be the arc whose cosine is c, and P the whole circumference, c is also the cosine of A + P, or A + 2P; therefore the cosines of 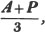and 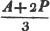are also values of x.

(517.) COR. 2. Since the radius is greater than the cosine, √4q/3 is greater than -3r/q, or 4q/3 is greater than 9r²/q²; that is, is q³/27 greater than r²/4; therefore this solution can only be applied when the roots of the cubic equation are possible. (See Art. 331.)

[page] 298

GENERAL PROPERTIES OF CURVE LINES.

(518.) A Curve is said to be of n dimensions, when the equation belonging to it rises to n dimensions.

Let 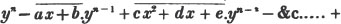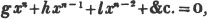a general equation of n dimensions, express the relation between the abscissa and ordinate of a curve, then for every different value of x, there are n values of y; therefore the ordinate will cut the curve in n, or in n - 2, n - 4, &c. points, according as the equation has n, or n - 2, n - 4, &c. possible roots.

(519.) COR. 1. Hence, if the equation be of an odd number of dimensions, the curve will have, at least, one infinite arc on each side of the point from which the absciss± are measured; for, whatever be the value of x, there is, at least, one possible value of y corresponding to it (Art. 278).

(520.) COR. 2. ax + b is the sum of the ordinates, cx² + dx + e the sum of the products of any two, &c. and gxⁿ + hx^{n - 1} + lx^{n - 2} + &c. is the product of all the ordinates (Art. 271).

(521.) If two parallel lines, be drawn

[page] 299

in a curve, and be cut by the right line AQ, in such a manner, that in each case, the sum of the ordinates, on one side of AQ is equal to the sum of the ordinates on the other, all lines drawn in the curve, parallel to these, will be cut by AQ in the same manner.

Let 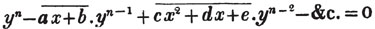be the equation to the curve, reckoning the absciss± from A; also, let AP = q, AQ = r; then the equation, in the two cases, becomes 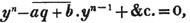and 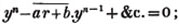since in each case, the sum of the positive ordinates is equal to the sum of the negative, aq + b = 0, and ar + b = 0; and by subtraction and 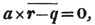or a = 0; hence, b = 0; therefore whatever be the value of x, ax + b = 0; or the sum of the ordinates on one side of AQ is equal to the sum of the ordinates on the other.

(522.) The line AQ is called a diameter of the curve.

(523.) If the abscissa APE, and ordinate NPQ cut a curve in as many points as it has dimensions,

the rectangle under the segments of the abscissa, PB x PC x PD x PE, will be to the rectangle under the ordinates, PM x PN x PO x PQ, in an invariable ratio.

[page] 300

Let be the equation to the curve; then gxⁿ + hx^{n - 1} + lx^{n - 2} + &c. = PM x PN x PO x PQ (Art. 520); also, the values of x, when y = 0, are AB, AC, AD, AE, that is, the roots of the equation gxⁿ + hx^{n - 1} + lx^{n - 2} + &c. = 0, or xⁿ + hx^{n - 1}/g + lx^{n - 2}/g + &c. = 0, are AB, AC, AD, AE; and consequently the quantity 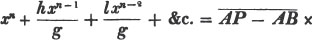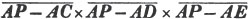= PB x PC x PD x PE (Art. 269); and gxⁿ + hx^{n - 1} + lx^{n - 2} + &c. = g x PB x PC x PD x PE = PM x PN x PO x PQ; therefore PB x PC x PD x PE : PM x PN x PO x PQ :: 1 : g.

(524.) COR. If n = 2, the curve is a conic section (Art. 501); and if the abscissa be a diameter, or the ordinates on each side of it, PM, PO, equal to each other, the rectangle under the segments of the abscissa is to the square of the ordinate in an invariable ratio.

(525.) If there be n right lines, and be ordinates to the abscissa

AP, the relation between the abscissa and ordinates will be expressed by an equation of the form yⁿ -

[page] 301

For if AP = x, then PM = ax + b, 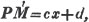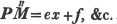where a, b, c, d, e, f are invariable (Art. 479); that is, the values of y are ax + b, cx + d, ex + f, &c. therefore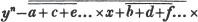 and if A = a + c + e...., B = b + d + f...., &c.

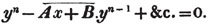
(526.) If a curve have as many asymptotes as it has dimensions, and a right line be drawn which cuts them all, the parts of the line measured from the asymptotes to the curve, will, together, be equal to the parts measured, in the same direction, from the curve to the asymptotes.

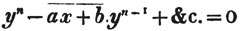the equation to the asymptotes (Art. 525); when x is infinite, the former equation becomes yⁿ - axy^{n - 1} + 1 &c. = 0, and the latter yⁿ - Axy^{n -1} + &c. = 0, and these equations coincide (Art. 506), therefore A = a; also, ax + b is the sum of the ordinates to the curve, and Ax + B, or ax + B, is the sum of the ordinates to the asymptotes, in all cases; hence, the difference of these, b - B, is an invariable quantity, whatever be the value of x; and at an infinite distance this difference is nothing (Art 506); therefore it is always nothing, or b = B; consequently ax + b = Ax + B; that is, the sum of the ordinates to the curve is equal to the sum of the ordinates to the asymptotes.

Let QONM be the curve, AP the abscissa, PQ an ordinate, meeting the curve in the points M, N, O, Q, and the asymptotes in a, b, c, d; then PM + PN + PO + PQ = Pa + Pb + Pc + Pd, and by trans-

[page] 302

position, PM - Pa + PO - Pc = Pb - PN + Pd -

PQ, or aM + cO = Nb + Qd.

(527.) COR. In the common hyperbola MCN, whose centre is O, and asymptotes Oa, Ob, if any line

aMNb be drawn cutting the curve in M, N, and the asymptotes in a, b, then aM is equal to Nb.

(528.) If a straight line be made to revolve about C, and cut the curve in as many points as it has dimensions; and if1/CD be always taken equal

[page] 303

to 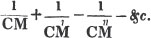the locus of the point D will be a straight line.

Let ABP be an abscissa, and from M and D draw

MP and DB at right angles to ABP, and let 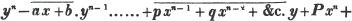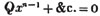be the equation which represents the relation of AP to PM. Also, let AC = z, CM = v, CB = w, BD = u; 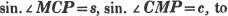radius 1. Then 1 : s :: v : sv = y, and 1 : c :: v : cv = CP; hence, x = AP = z + cv. If these values of x and y be substituted in the equation to the curve, the relation of AC to CM will be known; and the coefficient of the last term but one of the transformed equation, divided by the. last term, will be the sum of the reciprocals of it's roots, or 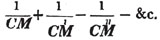(Art. 273). Now, since x = z + cv,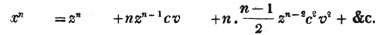

[page] 304

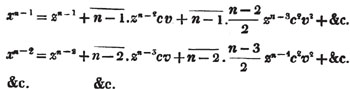
and substituting these values for x and it's powers, and sv for y, in the terms of the original equation, we have the two last terms of the transformed equation, 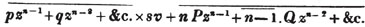x cv and Pzⁿ + Qz^{n - 1} divided, respectively, by sⁿ, all the other terms involving the square or some higher power of v; hence,

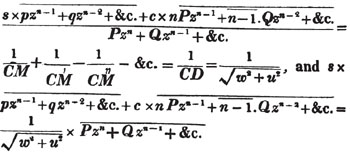
Also, from the similar triangles MCP, BCD,

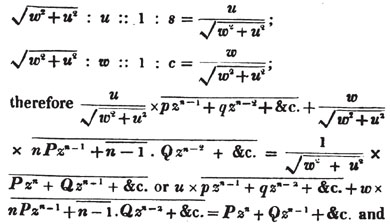
[page] 305

since the point C is fixed, AC, or z, is invariable; therefore the relation between w and u, or CB and BD, is expressed by a simple equation, that is, the locus of the point D is a straight line (Art. 480).

(529.) In the general equation 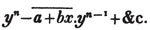if x be so assumed that two roots are impossible, two values of the ordinate belonging to this abscissa are impossible, that is, there are no lines which represent them. Hence it is evident, that in deducing the properties of the ordinates from the equation to the curve, we must suppose all the roots of this equation possible; because, though the sums, powers, products, &c. of such impossible quantities may become possible, and their relations, discovered by an algebraical process, may be expressed by possible quantities, yet the reasoning does not extend to curves, in which the original quantities cannot be represented.

On the subject of Algebraical Curves, the Reader may consult Dr. Waring's Proprietates Algebraicarum Curvarum, and Euler's Anal. Infinitorum.

THE END.